




Physics Experiment - To Determine the Radius of Curvature of a Given Spherical Surface Using a Spherometer
Introduction
Most of us wearing spectacles require at some point of time or the other to go to the optician, who examines the suitable lens and prescribes another pair effectively. But how does this task get carried out? A spherometer is capable of examining a regular curved surface and determining its radius of curvature using the known formula. These measurements are not possible to be measured with conventional measuring scales and, therefore, the use of a spherometer is vital for such measurements. Let us dive in detail on a hands-on of this simple experiment.
Table of Contents
Aim
Procedure
Observations
Results
Aim
To determine the radius of curvature of a given spherical surface by a spherometer.
Apparatus required
Curved surface
Spherometer
White paper sheet
Pencil
Ruler
Flat glass plate or plane mirror
Theory
As a spherometer is placed upon a curved surface convex to the height, its central screw touches the optical centre of the surface, while its legs touch the outer boundaries which are comparatively flat. This causes a difference in heights experienced by the central screw compared to that of the legs, which is readily measured using the main scale of the spherometer. This value combined with the average leg separation of the spherometer is incorporated into a formula, giving the accurate radius of curvature of the given surface.
Procedure
Keep the spherometer on a white sheet of paper on its three legs. Press the spherometer so that the pointed legs leave a slight mark on the paper.
Release the spherometer and rotate the circular scale to the maximum range till the central screw begins to touch the base, the same plane as the legs.
Ensure that at this position, the zero of the circular scale coincides with the zero of the main scale. If it does not, note the main scale division with which the circular scale coincides.
Rotate the circular scale once and note the distance covered by it on the main scale. This is the pitch.
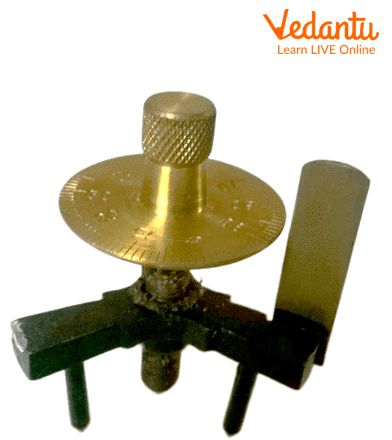
A Spherometer Consists of Three Coplanar Legs and a Circular Scale.
Note the number of divisions on the circular scale and divide the pitch of the spherometer with this. This is the least count.
Lift-off the spherometer from the paper sheet and pinpoint the leg marks as A, B and C using a pencil. Join the points to form an equilateral triangle ABC using a ruler.
Measure AB, BC and AC using a ruler. Note the values.
Now put the given curved surface on a plain glass slab or a plane mirror with the bulging face of the surface outwards.
Put the spherometer on the surface and adjust the central screw such that it touches at the center of the surface gently while the spherometer legs touch the points on the surface equidistant from the center.
Note the main scale division corresponding to the circular scale. This is main scale reading.
Note the circular scale division which coincides with the main scale. This is circular scale reading.
Note both the readings for the surface in a similar fashion two other times. Tabulate the observations.
Observations
Pitch of the spherometer =
Number of divisions on circular scale =
Least count = \[\dfrac{{Pitch}}{{Divisions\;on\;circular\;scale}}\]
Using centimeter scale,
Length AB =
Length BC =
Length AC =
Average leg separation, L = \[\dfrac{{AB + BC + AC}}{3}cm\]
Observation Table
Results
The average height of the curved surface=
Average reading, H = \[\dfrac{{Sum\;of\;readings}}{3}\] =
Using the spherometer formula
\[\dfrac{{{L^2}}}{{6H}} + \dfrac{H}{2}\]
where R = Radius of curvature of the given curved surface
L = Average leg separation
H = Average height of the surface
Putting the values of L and H obtained above, the value of R =
Precautions
Determine the leg separation very carefully by gently pressing the spherometer against the paper sheet.
Ensure to add/subtract the zero error in the final reading.
Determine the pitch very carefully from one rotation.
Lab Manual Questions
1. Does a given object have the same radius of curvature on its both surfaces (i.e. does the thickness of an object plays a role in radius of curvature)?
Ans: No, an object of considerable thickness does not have the same values of radius of curvature on its both surfaces. This is because the finite difference of the distances between the reference of measurement and the object's apparent surface due to thickness causes a finite error in measurement of the height as measured by the spherometer.
2. Name some commercial applications of a spherometer.
Ans: Spherometers are used to measure the radius of curvature of curved surfaces such as balls, optical lenses, mirrors, etc.
3. What is the least count of a spherometer?
Ans: The least count of a spherometer is 0.01 mm. It is the minimum length precisely measured by the spherometer and is obtained by dividing the pitch (1 mm) by the number of circular scale divisions (100).
4. Which instrument would be precise for measuring thickness of a card sheet – a screw gauge or a spherometer?
Ans: For measuring the thickness of a card sheet, a screw gauge would be more precise over a spherometer because a screw gauge is ideal for measuring small linear distances, whereas a spherometer is ideal for measuring the radius of curvature of a curved surface which is not a linear distance as the thickness of a card sheet.
Viva Questions
1. Name the material used for the construction of a spherometer.
Ans: A spherometer is made using stainless steel, copper and iron to make it durable and the readings engraved do not go off soon after usage.
2. What is the pitch of a spherometer?
Ans: The pitch of a spherometer is defined as the distance covered by the circular scale over the main scale on one complete rotation of the circular scale. It is roughly 1 mm.
3. Give the final calculation formula for the spherometer.
Ans: It is given by:
\[\dfrac{{{L^2}}}{{6H}} + \dfrac{H}{2}\]
where R = Radius of curvature of the given curved surface
L = Average leg separation
H = Average height of the surface
4. How does a spherometer differ from a vernier calliper/screw gauge?
Ans: A spherometer is used for the precise determination of radius of curvature of a given curved surface, whereas a vernier calliper/screw gauge is used for measuring very fine linear distances of the order of the diameter of a fine wire or the thickness of a sheet.
5. Explain the zero error of a spherometer. How to check it?
Ans: The zero error of a spherometer arises when the zero of the circular scale does not coincide with the zero of the main scale, causing an additional distance error. To check it, note the main scale division at which the circular scale coincides at the full range of the circular scale when all the four legs of the spherometer lie in the same plane on a base.
6. Give the formula for calculating the least count of the spherometer.
Ans: The least count of a spherometer can be calculated by dividing the distance traveled by the circular scale in one complete rotation along the main scale by the number of divisions on the circular scale.
7. Give three commercial uses of the spherometer.
Ans: The three commercial uses of a spherometer are as follows:
To measure the thickness of a micrometer chip.
To measure the elevation or depression in a slide.
To measure the curvature of a curved surface in aerodynamics.
8. Name the ideal quantity a spherometer is suitable for measuring.
Ans: A spherometer is ideally suitable for measuring the radius of curvature of a given curved surface using the surface elevation. This finds many applications such as in getting the right curvature for a given surface for critical engineering and medical purposes.
9. Explain the working principle of a spherometer.
Ans: A spherometer is based on the principle of a rotating screw. As the screw rotates, it covers a linear distance on the main scale. The difference between the levels of planes in which the legs and the central screw lie gives the estimate of the required curvature of the surface.
10. How many markings are there on the circular scale on a spherometer?
Ans: There are 100 markings on the circular scale on a spherometer.
Practical Based Questions
1. The least count of a spherometer is given by:
1 mm
0.1 mm
0.01 mm
0.001 mm
Ans: (C)
2. How many legs are there in a spherometer?
3
2
1
4
Ans: (A)
3. How many scales does a spherometer have?
2
3
5
6
Ans: (A)
4. The ratio of the least counts of the centimeter scale to that of a spherometer is:
1
10
100
1000
Ans: (C)
5. A spherometer can be used to accurately measure:
Edge of a dice
Thickness of a wire
Length of a notebook
Radius of curvature
Ans: (D)
6. State the value of radius of curvature of plane mirror:
Zero
10 m
1 km
Infinite
Ans: (D)
7. The error that arises when the zeroes of the main scale and the circular scale do not coincide is called:
Systematic error
Random error
Backlash error
Zero error
Ans: (D)
8. Accuracy of measurement can be increased by:
Increasing the number of divisions on circular scale
Using a magnifying glass
Using a thick wire for measuring diameter
Using more circular scales
Ans: (A)
9. How many types of zero errors are there?
One
Two
Three
Four
Ans: (B)
10. A spherometer is not suitable for measuring:
Radius of curvature
Elevation in a curved sheet
Diameter of a vessel
Depression in a curved sheet
Ans: (C)
Conclusion
From this experiment, we can conclude that the spherometer finds immense applications in the field of experimental physics in order to accurately measure the radius of curvature of curved surfaces, find the elevation/ depression of a given surface, etc. using its simplified formula. It finds various commercial applications in numerous engineering and medical fields in the society for the same. We have covered all the basic and fundamental concepts related to the spherometer along with its important commercial applications.
We hope that the reader is sufficiently enlightened regarding the very concept of the spherometer and is motivated to explore the field in the time to come.
FAQs on CBSE Physics Experiment To Determine the Radius of Curvature of a Given Spherical Surface Using a Spherometer
1. Explain in brief the difference between a spherometer and a vernier calliper.
A spherometer is a device consisting of a main scale with a circular scale sliding over it. It is capable of accurately measuring distances up to 0.01 mm. It is used for precise measurements of the radius of curvature of a curved surface, the minute depression /elevation etc.
On the other hand, a vernier calliper is a device consisting of a main scale with a small linear scale sliding over it. It is capable of accurately measuring distances up to 1 mm. It is used for measuring comparatively larger lengths than a screw gauge such as the sides of a square, the diameter and depth of a vessel etc.
2. How do you define the pitch and least count of a spherometer?
The pitch of a spherometer is defined as the linear distance covered by the circular scale over the main scale when the circular scale is given one complete rotation.
The least count is defined as the pitch divided by the number of divisions on the circular scale.
3. Explain the formula to take final readings.
The final reading is calculated by the formula
\[\dfrac{{{L^2}}}{{6H}} + \dfrac{H}{2}\]
where R = Radius of curvature of the given curved surface
L = Average leg separation
H = Average height of the surface
4. Explain the range of the spherometer.
The range of a spherometer is from 0 mm to 25 mm, with the least count 0.01 mm.
5. What is a backlash error? How is it eliminated?
The backlash error arises in the observations when the screw rotates but does not traverse any distance on the main scale. This usually arises when the screw is rotated in both the directions. Hence, it can be eliminated by rotating the screw in one direction only.






















