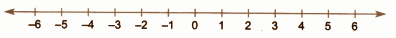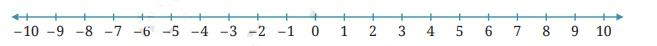Download CBSE Class 6 Maths Chapter 10 The Other Side of Zero Important Questions with Answers FREE PDF
FAQs on CBSE Class 6 Maths Important Questions Chapter 10 - The Other Side of Zero
1. What is the main focus of Chapter 10, The Other Side of Zero, in Class 6 Maths?
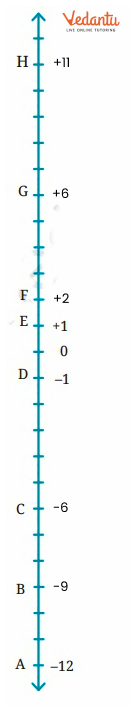
This chapter focuses on understanding positive and negative numbers, their placement on the number line, and how to compare values across zero.
2. Why is it important to learn about positive and negative numbers in Class 6 Maths ?
Learning about positive and negative numbers helps students understand everyday situations involving opposite values, like temperatures, bank balances, and elevations.
3. What is the significance of zero in CBSE Class 6 Maths Chapter 10?
Zero acts as the central point on the number line, separating positive and negative numbers, and it helps students understand the concept of opposite values.
4. According to CBSE Class 6 Maths Chapter 10 The Other Side Of Zero, how are integers useful in real life?
Integers are used in various real-life situations, such as tracking temperature changes, financial transactions, and altitude differences.
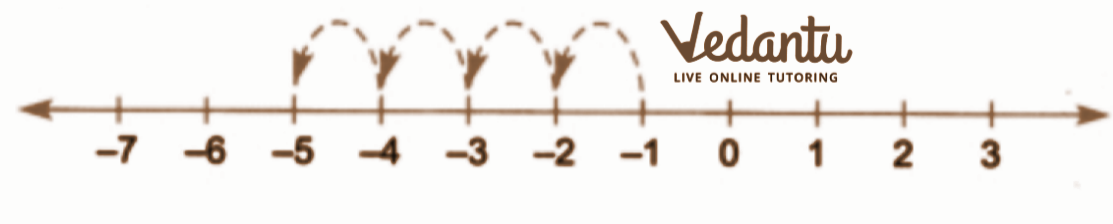
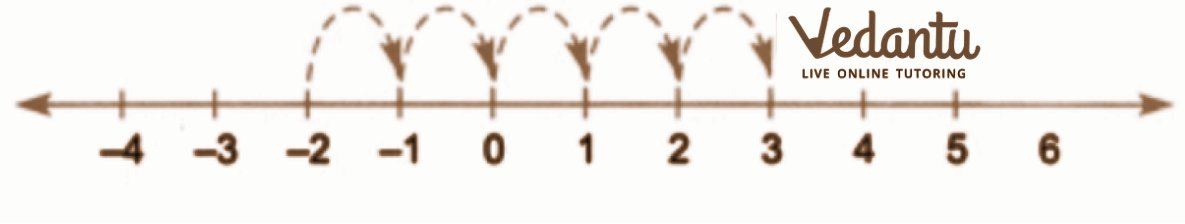
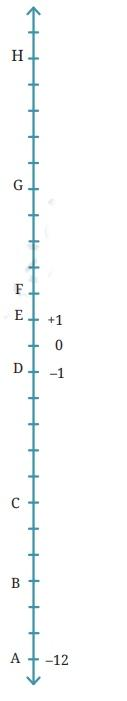
5. What is the role of the number line in Class 6 Maths Chapter 10, The Other Side of Zero?
The number line helps students visualise the position of integers, making it easier to understand positive, negative, and zero values.