Important Practice Problems for CBSE Class 7 Maths Chapter 5: Lines and Angles FREE PDF
Very Short Answer Type Questions 1- Mark
1. Define the following:
(a) Adjacent Angles
Ans: The angles are said to be adjacent only if they have a common arm/side and a common vertex and they do not overlap.
(b) Supplementary Angles
Ans: When the sum of two angles is ${180^\circ }$, then they are said to be supplementary angles.
(c) Complementary Angles
Ans: When the sum of two angles is ${90^\circ }$, then they are said to be complementary angles.
(d) Linear Pair of Angles
Ans: When a straight line is divided into two parts, i.e., two different angles. Then those angels are said to be linear pairs.
The measure of a straight angle is ${180^\circ }.$ So a linear pair of angles must add up to ${180^\circ }$.
(e) Vertically Opposite Angle
Ans: When two lines cross then they share the same vertex, vertically opposite angles are the angles opposite to one another having a common vertex.
Short Answer Type Questions 2- Marks
2. Write the complementary angle of ${57^\circ }$.
Ans: The sum of complementary angles is ${180^\circ }$.
Let the other angle be x, then
$x + {57^\circ } = {90^\circ }$
$x = {90^\circ } - {57^\circ }$
$x = {33^\circ }$
3. Write the supplementary angle of ${103^\circ }$.
Ans: The sum of complementary angles is ${180^\circ }$.
Let the other angle be x, then
$x + {103^\circ } = {180^\circ }$
$x = {180^\circ } - {103^\circ }$
$x = {77^\circ }$
4. Find the value of x in the given figure.
Ans:
$ACB{\text{ is a straight line}}{\text{.}}$
$\therefore \,\,\angle ACD + \angle BCD = {180^{\circ \,}}\,\,\,\,\left( {{\text{Linear}}\,{\text{pair}}} \right)$
$x + {130^\circ } = {180^\circ }$
$x = {180^\circ } - {130^\circ }$
$x = {50^\circ }$
5. Identify the supplementary and complementary angles.
${60^\circ },\,\,{120^\circ }$
${30^\circ },\,\,{60^\circ }$
${35^\circ },\,\,{145^\circ }$
${12^\circ },\,\,{78^\circ }$
Ans: Pair of angles whose sum is ${180^\circ }$ are called supplementary angles.
Here,
${60^\circ },\,\,{120^\circ }$ and ${35^\circ },\,\,{145^\circ }$ are supplementary angles.
Pair of angles whose sum is ${90^\circ }$ are called complementary angles.
Here,
${30^\circ },\,\,{60^\circ }$ and ${12^\circ },\,\,{78^\circ }$ are complementary angles.
6. In the following figures is $\angle 1{\text{ and }}\angle 2$ are adjacent? Give reason.
Ans: Adjacent angles are those that arise from the same vertex and have one arm/side in common.
Here,
$\angle 1{\text{ and }}\angle 2$ has a common arm/side but since they do not have a common vertex. Therefore, the angles are not adjacent.
7. Find the value of \[x,\,y\,{\text{ and }}z\].
Ans: From the figure it is clear that $\angle x$ and ${50^\circ }$ are vertically opposite angles
$\therefore \angle x = {50^\circ }$
$\angle x + \angle y = {180^\circ }\,\,\,\,\,\,\,\,\,\,\,\left( {{\text{Linear pair}}} \right)$
${50^\circ } + \angle y = {180^\circ }$
$\angle y = {180^\circ } - {50^\circ }$
$\angle y = {130^\circ }$
Similarly, $\angle y$ and $\angle z$ are vertically opposite angles.
$\therefore \angle y = \angle z = {130^\circ }$
8. If $\angle ABC = {55^\circ }$, then find
$\mathbf{\angle DGC}$
Ans: From the figure we can conclude that and a transversal line ${\text{BC}}$ is intersecting them.
$\angle DGC = \angle ABC\,\,\,\,\,\,\,\left( {{\text{corresponding angles}}} \right)$
$\therefore \angle DGC = {55^\circ }$
$\mathbf{\angle DEF}$
Ans: From the figure we can conclude that a traversal line DE is intersecting them.
$\angle DEF = \angle DGC\,\,\,\,\,\,\,\,\left( {{\text{corresponding angles}}} \right)$
$\therefore \angle DEF = {55^\circ }$
9. Find the angle which is equal to its complement.
Ans: Let the angle equal to its complement be ${\text{x}}$.
Since the complement of this angle is also ${\text{x}}$. Therefore,
The sum of the measures of a complementary angle pair is ${90^\circ }$.
$x + x = {90^\circ }\,\,\,\,\,\,\,\,\,\,\left( {{\text{complementary angles}}} \right)$
$\,\,\,\,\,2x = {90^\circ }$
$\,\,\,\,\,\,\,x = {45^\circ }$
10. Find the angle which is equal to its supplement.
Ans: Let the angle equal to its supplement be ${\text{x}}$.
Since the supplement of this angle is also ${\text{x}}$. Therefore,
The sum of the measures of a supplementary angle pair is ${180^\circ }$.
$x + x = {180^\circ }$
$\,\,\,\,\,2x = {180^\circ }$
$\,\,\,\,\,\,\,\,x = {90^\circ }$
Short Answer Type Questions 3 - Marks
11. Find the value of \[x,\,y\,{\text{ and }}z\] in each of the following:
Ans:
$\angle z = {30^\circ }$ (vertically opposite angles)
$\angle y + \angle z = {180^\circ }$ (linear pair)
$\angle y + {30^\circ } = {180^\circ }$
$\,\,\,\,\,\,\,\,\,\,\,\,\angle y = {150^\circ }$
${30^\circ } + \angle x + {30^\circ } = {180^\circ }$ (angles on a straight line)
${60^\circ } + \angle x = {180^\circ }$
$\,\,\,\,\,\,\,\,\,\,\,\angle x = {120^\circ }$
12. In the adjoining figure, identify:
(a) The Pairs of Corresponding Angles
Ans: When two parallel lines are intersected by any other line and the angle formed in the corresponding corner are called corresponding angles.
Here,
$\angle 1$ and $\angle 5,\,\,\,\angle 2$ and $\angle 6,\,\,\,\angle 3$ and $\angle 7,\,\,\,\angle 4$ and $\angle 8$
(b) The Pairs of Alternate Angles
Ans: they are the angles that lie on the inner side of the parallel lines but on the opposite sides of the transversal.
$\angle 3$ and $\angle 5,\,\,\,\angle 4$ and $\angle 6$
(c) The Pairs of Interior Angles on the Same Side of Traversal
Ans: when a pair of the parallel lines is intersected by a transversal, the pair of interior angles on the same side of the transversal are supplementary (sum to 180°).
$\angle 4$ and $\angle 5,\,\,\,\angle 3$ and $\angle 6$
(d) Vertically Opposite Angles
Ans: When two lines cross then they share the same vertex, vertically opposite angles are the angles opposite to one another having a common vertex.
$\angle 1$ and $\angle 3,\,\,\,\angle 2$ and $\angle 4,\,\,\,\angle 5$ and $\angle 7,\,\,\,\angle 6$ and $\angle 8$
13. Find the value of $x$ in the following figure.
Ans: From the figure, line $l$ is parallel to $m$ and a transversal passes through them. Hence,
$\angle y = {105^\circ }$ (corresponding angles)
$\angle x + \angle y = {180^\circ }$
$\angle y = {180^\circ } - {105^\circ }$
$\angle y = {75^\circ }$
14. Find the value of ${\text{x}}$ in each of the following figures is a parallelogram.
Ans: From the figure, line $l$ is parallel to $m$ and a transversal passes through them. Hence,
$\angle x = {120^\circ }$ (corresponding angles)
15. In the given figure check whether parallelogram.
Ans: Consider a pair of parallel lines l and m and a traversal line n which intersects them. Sum of the interior angles on the same side of traversal,
$ = {116^\circ } + {54^\circ } = {170^\circ }$
As the sum of interior angles on the same side of traversal is not ${180^\circ }$.
Therefore, l is not parallel to m.
5 Important Topics of Class 7 Chapter 5 Maths Lines and Angles You Shouldn’t Miss!
Nearly every aspect of our everyday lives includes lines and angles. To excel in the exams, students must be competent in calculating angles, measuring angles, and drawing angles. However, a proper understanding of lines and angles is essential for understanding the universal problems on lines and angles.
Let us have a look at important topics from the Lines and Angles Chapter.
Important Definitions of Class 7 Maths Chapter 5 - Lines and Angles
Line
A line is a one-dimensional figure that is parallel, has no thickness, and stretches in both directions indefinitely. It's commonly referred to as the shortest distance between two points.
There are 2 Types of Lines:
Intersecting Lines: Intersecting lines are created when two or more lines in a plane cross each other. The point of intersection is where the intersecting lines share a common point that occurs on all intersecting lines.
Non-Intersecting Lines: Non-intersecting lines are made up of two or more lines that do not intersect. These lines that do not intersect will never cross. The parallel lines are another name for them. They remain at the same distance from one another at all times.
Angles
In geometry, an angle is known as the figure created by two rays meeting at a common endpoint.
Pairs of Angles
Complementary Angles: If the degree measurements of two angles add up to 90 degrees, they are complementary angles. That is, if we link both angles and position them next to each other, they will form a right angle.
Supplementary Angles: If the sum of the degree measurements is 180° and one angle is said to be the supplement of the other then these angles are called supplementary angles. If we put the angles side by side, we get a straight line in supplementary angles.
Vertical Angles: At the intersection of two sides, vertical angles are the angles that are opposite each other. Since they have a common vertex, they are called vertical angles.
Alternate Interior Angles: When a transversal occurs, alternate interior angles are created. They are the angles on opposite sides of the transversal, but the transversal intersects inside the two lines. If the two lines intersected by the transversal are parallel, alternate interior angles are congruent.
Alternate Exterior Angles: Alternate exterior angles are congruent to each other in the same way as alternate interior angles are if the two lines intersected by the transversal are parallel. These angles are on opposite sides of the transversal, but the transversal intersects outside of the two lines.
Corresponding Angles: The pairs of angles on the same side of the transversal and on the corresponding sides of the two other lines are known as corresponding angles. When the two lines intersected by the transversal are parallel, these angles are equal in degree measure.
Benefit of Important Questions for CBSE Class 7 Chapter 5- Lines and Angles
Important Questions for CBSE Class 7 Chapter 5- Lines and Angles are crafted to help students understand and strengthen their basics in lines and angles.
These practice problems assist students in revising key topics, ensuring they grasp essential points in the chapter.
By solving various questions, students improve their problem-solving and analytical thinking abilities.
Covering a broad range of topics, the Important Questions for Class 7 Maths equip students well for exams.
The questions encourage students to apply geometric principles in real-life scenarios, making learning more relevant.
They support academic growth and enhance critical thinking skills that are useful beyond mathematics.
Conclusion
Lines and Angles is one of the most scoring topics for Class 7 students. Students can download the free PDF for Lines and Angles Class 7 Important Questions from Vedantu to prepare for their exams. We provide step-by-step solutions to help students understand the concepts easily. All solutions are according to the CBSE guidelines. So download the Class 7 Maths Chapter 5 Extra Questions and prepare well for your exams.
Important Study Materials for Class 7 Maths Chapter 5
CBSE Class 7 Maths Important Questions for All Chapters
Class 7 Maths Important Questions and Answers cover key topics, aiding in thorough preparation and making revision simpler.
Important Study Materials for Class 7 Maths
FAQs on CBSE Class 7 Maths Important Questions Chapter 5 - Lines and Angles
1. What are the benefits of solving important questions for Chapter 5 – Lines and Angles in Class 7 Maths?
Solving important questions for this chapter offers several benefits. First, it helps in strengthening core concepts related to lines, angles, and their relationships, making it easier to understand advanced geometry. The questions also cover various difficulty levels, which improves students’ problem-solving skills by encouraging them to think critically and analytically. Additionally, practising these questions prepares students well for exams, as they get familiar with different types of problems that may appear in assessments. This practice reinforces understanding, enhances recall, and boosts confidence in the subject.
2. How do these important questions help in preparing for exams?
Important questions for Chapter 5 are designed to cover all major topics within lines and angles, making them an effective study tool. They focus on key areas that are commonly tested in exams, such as types of angles, angle pairs, and properties of parallel lines intersected by a transversal. By working through these questions, students gain clarity on critical concepts and improve their accuracy in solving related problems. This comprehensive preparation helps students feel confident and better equipped to handle exam questions efficiently, reducing exam-related stress.
3. How can important questions enhance understanding of concepts like supplementary, complementary, and vertically opposite angles?
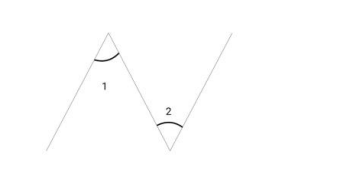
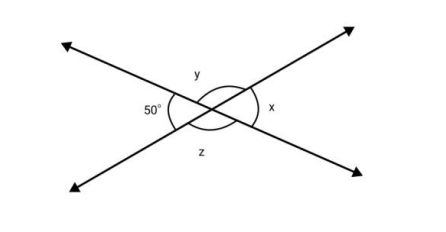
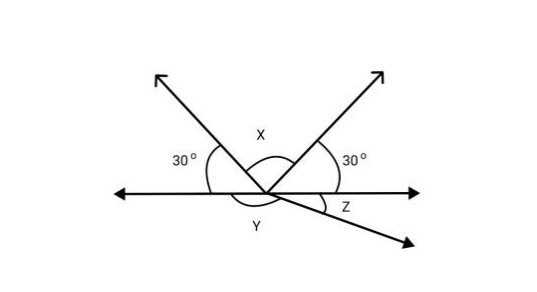
Important questions often include problems that focus on identifying and calculating supplementary, complementary, and vertically opposite angles, which are essential in geometry. These questions provide a step-by-step approach to learning these concepts, making it easier to grasp their relationships and properties. For instance, by practising questions on complementary and supplementary angles, students learn how to solve problems involving angle sums. This repetition of concepts helps reinforce memory and boosts the ability to quickly identify angle types, improving overall comprehension.
4. Are important questions for Lines and Angles useful for real-life applications?
Yes, solving important questions on lines and angles helps students see the practical side of geometry. Concepts such as angles, lines, and their properties have applications in fields like architecture, engineering, and art. By understanding how lines and angles work together, students can relate these ideas to real-world scenarios, such as calculating the angles in structures or understanding the layout of buildings. This not only deepens their understanding but also shows the relevance of mathematics beyond the classroom, helping to make learning more engaging and meaningful.
5. Why are important questions essential for developing critical thinking in students?
Important questions are crafted to challenge students at multiple levels, encouraging them to think beyond straightforward calculations. Many questions require logical reasoning, especially when proving relationships between angles or solving complex angle-based problems. This type of questioning helps develop critical thinking skills, as students learn to approach problems methodically, consider different angles (both literal and figurative), and find solutions. These skills are beneficial not only for maths but also for subjects that require logical analysis and reasoning skills.
6. How do important questions help students understand the relationship between parallel lines and a transversal?
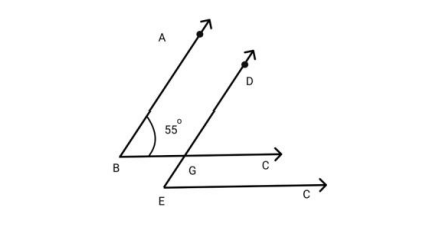
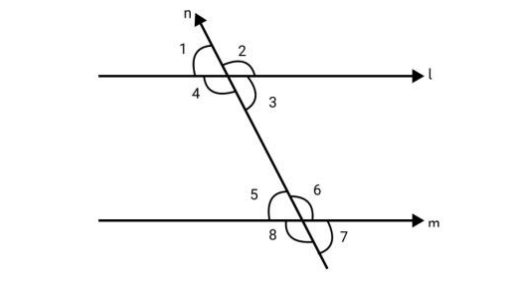
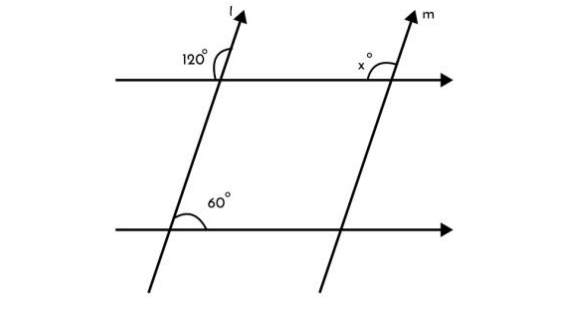
Important questions often include scenarios with parallel lines intersected by a transversal, which helps students explore angle relationships like corresponding, alternate, and interior angles. By working on these types of problems, students learn how to identify these angles and understand the properties that apply. This practice also aids in visualising how different angles relate to each other, helping students to solve geometry problems with greater confidence and accuracy.
7. In what ways do important questions assist students in mastering angle calculations?
Important questions cover a range of angle calculation problems, from basic to complex, helping students practise calculating unknown angles in various shapes and situations. This consistent practice enables them to learn and apply formulas for angle sums in triangles, quadrilaterals, and around intersecting lines. As a result, students become more skilled at angle calculations, which are crucial in both basic geometry and more advanced maths topics.
8. How are these important questions helpful for understanding adjacent and linear pair angles?
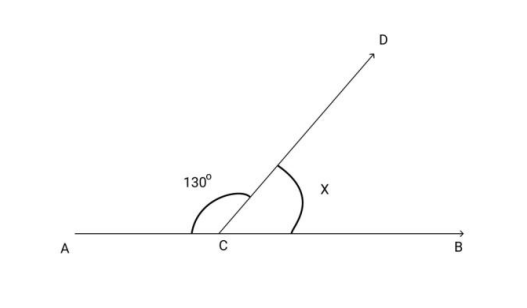
These questions often include problems that specifically focus on adjacent and linear pair angles, allowing students to observe how these angles are related. For example, they learn that the sum of angles in a linear pair is always 180°, and adjacent angles share a common arm. Through various examples and problem-solving exercises, students strengthen their ability to identify and work with these types of angles, which is a foundation for many geometric proofs and applications.
9. Do important questions improve students' time management skills in exams?
Yes, practising important questions can significantly improve time management skills. By familiarising themselves with different types of questions, students learn how to quickly recognise problem types and apply suitable methods, which reduces time spent on each question. This preparation allows students to complete exams within the time limit and also provides more time to review their answers, ultimately improving their performance.
10. How do important questions aid in revising the entire chapter on lines and angles?
Important questions are chosen to cover all significant concepts in the chapter, making them a comprehensive revision tool. By solving these questions, students review concepts like types of angles, angle relationships, and properties of lines. This targeted revision helps in reinforcing learning and ensures that students have a well-rounded understanding of the chapter, which is particularly beneficial before exams.
11. Can important questions help identify areas where students need improvement?
Yes, working on important questions helps students identify which areas of the chapter they are struggling with. If they find specific types of questions challenging, such as those involving angle calculations or angle relationships, they can focus more on those areas. This self-assessment approach enables them to concentrate on weak spots, allowing for focused improvement before assessments.
12. Why is consistent practice with important questions beneficial for long-term learning?
Consistent practice with important questions not only prepares students for immediate exams but also builds a strong foundation for future maths topics that require geometric knowledge. When students repeatedly solve angle and line-related problems, these concepts become second nature, making it easier for them to tackle more complex geometry topics in higher classes. This long-term retention is beneficial as it reinforces fundamental skills that are useful beyond a single chapter or exam.
