Exponents and Powers Class 8 Important Questions: CBSE Maths Chapter 10

Very Short Answer Questions                                                   1 Marks

1. \[\mathrm{3}\] multiplied fifteen times is written as?

Ans:

\[3\] Multiplied for fifteen times is written as 

\[3\times 3\times 3\times 3\times 3\ldots 15 times={{3}^{15}}\]

Answer will be \[{{3}^{15}}\]

2. What is the base of the exponent \[{{\mathrm{6}}^{\mathrm{9}}}\]?

\[\begin{align} & \mathrm{(a)6} \\  & \mathrm{(b)2} \\  & \mathrm{(c)9} \\  & \mathrm{(d)None} \\  \end{align}\]

Ans:

The base of the exponent \[{{6}^{9}}\] is \[6\]

3. Find the missing number \[{{\mathrm{7}}^{\mathrm{5}}}\mathrm{=}\dfrac{\mathrm{1}}{{{\mathrm{7}}^{\square }}}\]?

\[\begin{align} & \mathrm{(a)2} \\  & \mathrm{(b)-5} \\  & \mathrm{(c)1} \\  & \mathrm{(d)None} \\  \end{align}\]

Ans:

The missing number should be  \[-5\]

So the answer will be \[{{7}^{5}}=\dfrac{1}{{{7}^{-5}}}\]

4. Find the value of  \[{{\left( {{\mathrm{5}}^{\mathrm{2}}} \right)}^{\mathrm{2}}}\]

\[\begin{align} & \mathrm{(a)125} \\  & \mathrm{(b)625} \\  & \mathrm{(c)25} \\  & \mathrm{(d)0} \\  \end{align}\]

Ans:

The solution will be

\[\begin{align} & {{\left( {{5}^{2}} \right)}^{2}}={{5}^{4}} \\  & {{\left( {{5}^{2}} \right)}^{2}}=5\times 5\times 5\times 5 \\  & {{\left( {{5}^{2}} \right)}^{2}}=625 \\  \end{align}\]

5. Find the value of \[\mathrm{x}\], when \[{{\mathrm{2}}^{\mathrm{x}}}\mathrm{=}{{\mathrm{4}}^{\mathrm{4}}}\]

\[\begin{align} & \mathrm{(a)x=6} \\  & \mathrm{(b)x=2} \\  & \mathrm{(c)x=8} \\  & \mathrm{(d)x=-5} \\  \end{align}\]

Ans:

The solution will be 

\[{{2}^{x}}={{4}^{4}}\]

\[\begin{align} & {{4}^{4}}={{\left( {{2}^{2}} \right)}^{4}} \\  & {{4}^{4}}={{2}^{8}} \\  \end{align}\]

\[{{2}^{x}}={{2}^{8}}\]

\[x=8\]

So the answer will be \[x=8\]

6. Find the value of \[{{\left( {{\mathrm{2}}^{\mathrm{11}}}\mathrm{+}{{\mathrm{6}}^{\mathrm{2}}}\mathrm{-}{{\mathrm{5}}^{\mathrm{1}}} \right)}^{\mathrm{0}}}\]

\[\begin{align} & \mathrm{(a)0} \\  & \mathrm{(b)-1} \\  & \mathrm{(c)1} \\  & \mathrm{(d)None} \\  \end{align}\]

Ans:

The solution will be 

\[\begin{align} & {{\left( {{2}^{11}}+{{6}^{2}}-{{5}^{1}} \right)}^{0}}={{\left( anything \right)}^{0}} \\  & {{\left( {{2}^{11}}+{{6}^{2}}-{{5}^{1}} \right)}^{0}}=1 \\  \end{align}\]

So the solution will be 

\[{{\left( {{2}^{11}}+{{6}^{2}}-{{5}^{1}} \right)}^{0}}=1\]

7.\[{{\mathrm{I}}^{\mathrm{3}}}\mathrm{+}{{\mathrm{I}}^{\mathrm{-3}}}\mathrm{=?}\] What is the solution?

\[\begin{align} & \mathrm{(a)2} \\  & \mathrm{(b)3} \\  & \mathrm{(c)-3} \\  & \mathrm{(d)None} \\  \end{align}\]

Ans:

The solution will be 

\[\begin{align} & {{I}^{3}}+{{I}^{-3}}=\left( I\times I\times I \right)+\dfrac{1}{\left( I\times I\times I \right)} \\ & {{I}^{3}}+{{I}^{-3}}=\left( -I \right)+\dfrac{1}{\left( -I \right)} \\  & {{I}^{3}}+{{I}^{-3}}=\dfrac{-1+1}{\left( -I \right)} \\  & {{I}^{3}}+{{I}^{-3}}=0 \\  \end{align}\]

So the solution is (d)

Short Answer Questions                                                          2 Marks

8. Follow the pattern and complete

Ans:

The pattern for the solution is square root of the numbers which continue as 

\[\begin{align} & 1234321={{1111}^{2}} \\  & 123454321={{11111}^{2}} \\ \end{align}\]

9. If \[{{\mathrm{2}}^{\mathrm{x}}}\mathrm{ }\!\!\times\!\!\text{ }{{\mathrm{5}}^{\mathrm{x}}}\mathrm{=1000}\] then \[\mathrm{x=?}\]

Ans:

For solving we will just factorise 

\[\begin{align} & {{2}^{x}}\times {{5}^{x}}=1000 \\  & {{2}^{x}}\times {{5}^{x}}=5\times 5\times 5\times 2\times 2\times 2 \\  & {{2}^{x}}\times {{5}^{x}}={{2}^{3}}\times {{5}^{3}} \\  & x=3 \\ \end{align}\]

10.Find\[{{\mathrm{3}}^{\mathrm{4}}}\mathrm{+}{{\mathrm{4}}^{\mathrm{3}}}\mathrm{+}{{\mathrm{5}}^{\mathrm{3}}}\] and give the answers in cube 

Ans:

Solve the expression 

\[\begin{align} & {{3}^{4}}+{{4}^{3}}+{{5}^{3}}=27+64+125 \\  & {{3}^{4}}+{{4}^{3}}+{{5}^{3}}=216 \\  & {{3}^{4}}+{{4}^{3}}+{{5}^{3}}=6\times 6\times 6 \\  & {{3}^{4}}+{{4}^{3}}+{{5}^{3}}={{6}^{3}} \\  \end{align}\]

11. Find the missing number \[\mathrm{x}\] in  \[{{\mathrm{5}}^{\mathrm{2}}}\mathrm{+}{{\mathrm{x}}^{\mathrm{2}}}\mathrm{=1}{{\mathrm{3}}^{\mathrm{2}}}\]

Ans:

Solve the expression

\[\begin{align} & {{5}^{2}}+{{x}^{2}}={{13}^{2}} \\  & 25+{{x}^{2}}=169 \\  & {{x}^{2}}=144 \\  & x=\sqrt{144} \\  & x=12 \\  \end{align}\]

12. Simplify in exponent form \[\left( {{\mathrm{3}}^{\mathrm{4}}}\mathrm{ }\!\!\times\!\!\text{ }{{\mathrm{3}}^{\mathrm{2}}} \right)\mathrm{ }\!\!\div\!\!\text{ }{{\mathrm{3}}^{\mathrm{-4}}}\]

Ans:

Solve the expression 

\[\begin{align} & \left( {{3}^{4}}\times {{3}^{2}} \right)\div {{3}^{-4}}=\dfrac{{{3}^{4+2}}}{{{3}^{-4}}} \\  & \left( {{3}^{4}}\times {{3}^{2}} \right)\div {{3}^{-4}}=\dfrac{{{3}^{6}}}{{{3}^{-4}}} \\  & \left( {{3}^{4}}\times {{3}^{2}} \right)\div {{3}^{-4}}={{3}^{6+4}} \\  & \left( {{3}^{4}}\times {{3}^{2}} \right)\div {{3}^{-4}}={{3}^{10}} \\  \end{align}\]

 13. Expand 

\[\begin{align} & \mathrm{(a)1526}\mathrm{.26} \\  & \mathrm{(b)8379} \\ \end{align}\]Using exponents

Ans:

Solve in exponential form 

\[\begin{align} & (a)1526.26=1\times {{10}^{3}}+5\times {{10}^{2}}+2\times {{10}^{1}}+6\times {{10}^{\circ }}+2\times {{10}^{-1}}+6\times {{10}^{-2}} \\  & (b)8379=8\times {{10}^{3}}+3\times {{10}^{2}}+7\times {{10}^{1}}+9\times {{10}^{0}} \\ \end{align}\]

14. Simplify using laws of exponents

\[\begin{align} & \mathrm{(a)}\dfrac{\mathrm{1}}{\mathrm{9}}\mathrm{ }\!\!\times\!\!\text{ }{{\mathrm{3}}^{\mathrm{5}}} \\  & \mathrm{(b)}{{\mathrm{5}}^{\mathrm{a}}}\mathrm{ }\!\!\times\!\!\text{ 2}{{\mathrm{5}}^{\mathrm{b}}} \\  \end{align}\]

Ans:

Solve in exponential form 

\[\begin{align} & (a)\dfrac{1}{9}\times {{3}^{5}}=\dfrac{{{3}^{5}}}{9}=\dfrac{{{3}^{5}}}{{{3}^{2}}}={{3}^{5-2}}={{3}^{3}}=27 \\  & (b){{5}^{a}}\times {{25}^{b}}={{5}^{a}}\times {{\left( {{5}^{2}} \right)}^{b}}={{5}^{a}}\times {{5}^{b}}={{5}^{a+2b}} \\  \end{align}\]

15. Express the following number as a product of powers of prime factors.

\[\begin{align} & \mathrm{(a)1225} \\  & \mathrm{(b)3600} \\  \end{align}\]

Ans:

Solve in exponential form 

\[\begin{align} & (a)1225=5\times 5\times 7\times 7 \\  & 1225={{5}^{2}}\times {{7}^{2}} \\  \end{align}\]

\[\begin{align} & (b)3600=2\times 2\times 2\times 2\times 3\times 3\times 5\times 5 \\  & 3600={{2}^{4}}\times {{3}^{2}}\times {{5}^{2}} \\  \end{align}\]

16. Express the following large no’s in its scientific notation. 

\[\begin{align} & \mathrm{(a)650200000000} \\  & \mathrm{(b)301000000} \\ \end{align}\]

Ans:

Solve in exponential form

\[(a)650200000000=6.502\times {{10}^{11}}\] 

\[(b)301000000=3.01\times {{10}^{8}}\]

17. Express the following in expanded form \[\begin{align} & \mathrm{(a)1}\mathrm{.682 }\!\!\times\!\!\text{ 1}{{\mathrm{0}}^{\mathrm{5}}} \\  & \mathrm{(b)0}\mathrm{.86 }\!\!\times\!\!\text{ 1}{{\mathrm{0}}^{\mathrm{4}}} \\  \end{align}\]

Ans:

Solve in exponential form

\[\begin{align} & (a)168200 \\  & (b)8600 \\  \end{align}\]

Short Answer Questions                                                          3 Marks

18. State true or false

$\begin{align} & \mathrm{(a)}\left( \mathrm{1}{{\mathrm{0}}^{\mathrm{0}}}\mathrm{+1}{{\mathrm{2}}^{\mathrm{0}}} \right)\left( \mathrm{1}{{\mathrm{6}}^{\mathrm{0}}}\mathrm{+1}{{\mathrm{2}}^{\mathrm{0}}} \right)\mathrm{}{{\mathrm{8}}^{\mathrm{2}}} \\  & \mathrm{(b)}{{\left( {{\mathrm{3}}^{\mathrm{4}}} \right)}^{\mathrm{2}}}\mathrm{=}{{\mathrm{3}}^{\mathrm{8}}} \\  & \mathrm{(c)}{{\left( {{\mathrm{5}}^{\mathrm{2}}} \right)}^{\mathrm{3}}}\mathrm{=100000} \\  \end{align}$

Ans:

(a) False

(b) True

(c) False

19. Simplify \[\dfrac{{{\mathrm{5}}^{\mathrm{2}}}\mathrm{ }\!\!\times\!\!\text{ }{{\mathrm{a}}^{\mathrm{-4}}}}{{{\mathrm{5}}^{\mathrm{-3}}}\mathrm{ }\!\!\times\!\!\text{ 10 }\!\!\times\!\!\text{ }{{\mathrm{a}}^{\mathrm{-8}}}}\] , where \[\mathrm{a}\ne \mathrm{0}\]

Ans:

Solve the expression 

\[\begin{align} & \dfrac{{{5}^{2}}\times {{a}^{-4}}}{{{5}^{-3}}\times 10\times {{a}^{-8}}}={{5}^{2}}\times {{a}^{-4}}\times {{5}^{3}}\times 10\times {{a}^{8}} \\ & =\left( {{5}^{2}}\times {{5}^{3}} \right)\times \left( {{a}^{8}}\times {{a}^{-4}} \right)\times 10 \\  & =\left( {{5}^{2+3}} \right)\left( {{a}^{8-4}} \right)\times 10 \\  & ={{5}^{5}}\times {{a}^{4}}\times 10 \\  & \dfrac{{{5}^{2}}\times {{a}^{-4}}}{{{5}^{-3}}\times 10\times {{a}^{-8}}}=10{{(a)}^{4}}{{(5)}^{5}} \\ \end{align}\]

20. Find the area of the square attached to the hypotenuse in the diagram. Express the solution in exponential form.

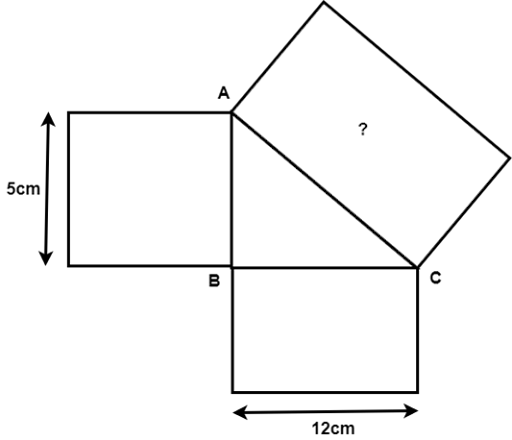
Ans:

From Pythagoras theorem 

\[\begin{align} & Are{{a}_{(longside)}}={{A}_{side1}}+{{A}_{side2}} \\  & A={{5}^{2}}+{{12}^{2}} \\  & A=169 \\  & A={{13}^{2}}c{{m}^{2}} \\  \end{align}\]

21.Simplify\[\left[ \left\{ {{\left( \dfrac{\mathrm{1}}{\mathrm{3}} \right)}^{\mathrm{-3}}}\mathrm{-}{{\left( \dfrac{\mathrm{1}}{\mathrm{2}} \right)}^{\mathrm{-3}}}\mathrm{ }\!\!\div\!\!\text{ }{{\left( \dfrac{\mathrm{1}}{\mathrm{5}} \right)}^{\mathrm{-2}}} \right\} \right]\]

Ans:

Solve the expression
\[\begin{align} & \left[ \left\{ {{\left( \dfrac{1}{3} \right)}^{-3}}-{{\left( \dfrac{1}{2} \right)}^{-3}}\div {{\left( \dfrac{1}{5} \right)}^{-2}} \right\} \right] \\ & =\left[ \dfrac{{{1}^{-3}}}{{{3}^{-3}}}-\dfrac{{{1}^{-3}}}{{{2}^{-3}}}\div \dfrac{{{1}^{-2}}}{{{5}^{-2}}} \right] \\ & =\left[ \left( \dfrac{{{3}^{3}}}{{{1}^{3}}}-\dfrac{{{2}^{3}}}{{{1}^{3}}} \right)\div \dfrac{{{5}^{2}}}{{{1}^{2}}} \right] \\  & =\left( \dfrac{27}{1}-\dfrac{8}{1} \right)\div 25 \\  & =\dfrac{(27-8)}{25} \\  & =\dfrac{19}{25} \\ \end{align}\]

22. if \[\mathrm{x=}{{\left( \dfrac{\mathrm{5}}{\mathrm{2}} \right)}^{\mathrm{2}}}\mathrm{ }\!\!\times\!\!\text{ }{{\left( \dfrac{\mathrm{2}}{\mathrm{5}} \right)}^{\mathrm{-3}}}\] find the value of \[{{\mathrm{x}}^{\mathrm{-2}}}\]

Ans:

\[\begin{matrix} x={{\left( \dfrac{5}{2} \right)}^{2}}\times {{\left( \dfrac{2}{5} \right)}^{-3}}  \\ x={{\left( \dfrac{5}{2} \right)}^{2}}\times \dfrac{{{5}^{3}}}{{{2}^{3}}}  \\ x=\dfrac{{{5}^{4}}}{{{2}^{4}}}={{\left( \dfrac{5}{2} \right)}^{4}}  \\ \end{matrix}\]

The value of \[{{x}^{-2}}={{\left[ {{\left( \dfrac{5}{2} \right)}^{4}} \right]}^{-2}}\]

\[\begin{align} & {{x}^{-2}}={{\left[ {{\left( \dfrac{5}{2} \right)}^{4}} \right]}^{-2}} \\  & {{x}^{-2}}={{\left( \dfrac{5}{2} \right)}^{4\times (-2)}} \\  & {{x}^{-2}}={{\left( \dfrac{5}{2} \right)}^{-8}} \\  & {{x}^{-2}}={{\left( \dfrac{2}{5} \right)}^{8}} \\  \end{align}\]

23. Prove that \[{{\left[ {{\left( \dfrac{\mathrm{1}}{\mathrm{2}} \right)}^{\mathrm{2}}} \right]}^{\mathrm{3}}}\mathrm{ }\!\!\times\!\!\text{ }{{\left( \dfrac{\mathrm{1}}{\mathrm{3}} \right)}^{\mathrm{-4}}}\mathrm{ }\!\!\times\!\!\text{ }{{\mathrm{3}}^{\mathrm{-2}}}\mathrm{ }\!\!\times\!\!\text{ }\dfrac{\mathrm{1}}{\mathrm{6}}\mathrm{=}\dfrac{\mathrm{3}}{\mathrm{128}}\]

Ans:

Solve the left hand side and equate with the right

\[\begin{align} & LHS={{\left[ {{\left( \dfrac{1}{2} \right)}^{2}} \right]}^{3}}\times {{\left( \dfrac{1}{3} \right)}^{-4}}\times {{3}^{-2}}\times \dfrac{1}{6} \\  & ={{\left( \dfrac{{{1}^{2}}}{{{2}^{2}}} \right)}^{3}}\times {{\left( {{3}^{-1}} \right)}^{-4}}\times {{3}^{-2}}\times \dfrac{1}{2}\times \dfrac{1}{3} \\ & =\dfrac{1}{{{2}^{6}}}\times {{3}^{4}}\times {{3}^{-2}}\times \dfrac{1}{2}\times {{3}^{-1}} \\  & =\dfrac{1}{{{2}^{7}}}\times {{3}^{4-3}} \\  & =\dfrac{3}{128} \\  \end{align}\]

24. A dish holds \[\mathbf{100}\] bacteria. It is known that the bacteria double in number every hour.

How many bacteria will be present after each number of hours?

\[\begin{align} & \mathrm{(a)1} \\  & \mathrm{(b)5} \\  & \mathrm{(c)n} \\  & \mathrm{(d)}\dfrac{\mathrm{3}}{\mathrm{2}} \\  \end{align}\]

Ans:

Number of hours will multiply with the number of bacteria two the power of two 

\[\begin{align} & (a)100\left( {{2}^{1}} \right)=200 \\  & (b)100\left( {{2}^{5}} \right)=100\left( 32 \right) \\  & 100\left( {{2}^{5}} \right)=3200 \\  & (c)100\left( {{2}^{n}} \right) \\  & (d)100\left( {{2}^{\dfrac{3}{2}}} \right) \\ \end{align}\]

25. What is the area of the rectangle with the width of \[\mathrm{6}{{\mathrm{x}}^{\mathrm{2}}}\]and the length of\[\mathrm{12}{{\mathrm{x}}^{\mathrm{3}}}\]? After finding the area, find the solution at \[\mathrm{x=2m}\] .

Ans:

\[Area\text{ }of\text{ }rectangle\text{ }=\text{ }length\times ~breadth\]

\[\begin{align} & A=12{{x}^{3}}\times 6{{x}^{2}} \\  & A=72{{x}^{5}} \\  & at,x=2m \\  & A=72\times {{\left( 2 \right)}^{5}} \\  & A=72\times 32 \\  & A=2304{{m}^{2}} \\  \end{align}\]

Class 8 Maths Exponents and Powers Important Questions

The Exponents and Powers Class 8 Important Questions are also available in the PDF format which students can download on their devices and access offline as per their convenient time. With these questions in hand, students will surely get a better conceptual understanding and learn how to divide their time into more important topics than the less significant ones. The questions are based on the topics that are outlined below.

List of Formulas of Class 8 Maths Chapter 10 Exponents and Powers

Find here the list of all important formulas discussed in the chapter. Students can solve all the questions asked in the chapter using the formulas given below.

Practice Questions of Chapter 10 Exponents and Powers

For more practice, we have provided some practise questions for the students. Try to solve these questions.

1. What is the value of 90?

2. What is the value of 143/53?

3. Find the value of 75 x 95.

4. Find the value of (62)3.

5. Evaluate the value of 87 x 85.

These questions are very beneficial for the students. Practice the important questions along with the practice question given above. These questions are solved by the subject-matter experts following the pattern of CBSE guidelines.

Why are Important Questions for Class  8 Maths Chapter 10 - Exponents and Powers Necessary?

• Important questions in Vedantu's Class 8 Maths Chapter 10 - Exponents and Powers help reinforce key concepts covered in the chapter.

• They serve as a targeted study resource for students preparing for exams, as they often focus on the types of questions commonly found in examinations.

• These questions are designed to challenge students' problem-solving skills, promoting a deeper understanding of the topic.

• Students can use these questions to self-assess their understanding of the chapter, identifying areas that may need further review.

• By practising important questions, students can improve their time management skills, essential for completing exams within the allocated time.

• These questions often involve the application of theoretical knowledge to practical problems, enhancing the student's ability to apply concepts in different scenarios.

• Regular practice with important questions can boost a student's confidence, helping them approach exams with a more positive mindset.

• Important questions typically cover a range of topics within the chapter, ensuring a comprehensive review of the material.

• For students aspiring to pursue further studies or competitive exams, practising these questions can be valuable in building a strong foundation in mathematics.

Tips to learn Class 8 Maths Chapter 10 Exponents and Powers using Important Questions

• Before solving questions, get clear on concepts like laws of exponents, negative exponents, and standard form. This foundation makes it easier to tackle problems.

• Focus on the key formulas, like multiplying or dividing exponents and simplifying powers. Write them down and practice their application in different types of questions.

• When working on important questions, break the problem into smaller steps. Don’t rush; focus on accuracy to avoid mistakes.

• Practice a variety of problems, including MCQs, short answers, and word problems. This will prepare you for different question formats in exams.

• After solving questions, check your answers carefully. Understand any mistakes and revisit the concepts behind them to improve.

Conclusion

Chapter 10 of CBSE Class 8 Maths focuses on Exponents and Powers, which are essential concepts in understanding how numbers grow when raised to different powers. Key topics include laws of exponents, such as product rule, quotient rule, and power rule, which help simplify expressions with powers. Students also learn how to express large numbers in scientific notation and calculate values for negative exponents. Mastering these concepts is crucial for solving complex mathematical problems. Overall, this chapter helps build a strong foundation for understanding higher-level mathematics and problem-solving in various real-world scenarios.

Related Study Materials for CBSE Class 8 Maths Chapter 10

CBSE Class 8 Maths Chapter-wise Important Questions

Other Important Related Links for CBSE Class 8 Maths