What is the Area of Hexagon? And its Formula Derivation
The area of a hexagon is the space enclosed by its six sides. A hexagon is a closed shape made up of six straight line segments and six interior angles. The word "hexagon" is derived from the Greek terms ‘Hexa,’ meaning six, and ‘Gonia,’ meaning angles or corners. In a hexagon, the sum of all interior angles is always 720°. This article will guide you through the process of calculating the area of a hexagon using these properties for JEE Main 2025.
What is a Hexagon?
A polygon having six sides and six angles is called a Hexagon. Regular hexagons have six equal sides and six angles and are composed of six equilateral triangles. There are a variety of ways to calculate the area of a hexagon, whether you're working with an irregular hexagon or a regular hexagon. There are various ways to determine the area of hexagon formula. The various methods are mainly based on how you spit the hexagon. You may divide it into 6 equilateral triangles or two triangles and one rectangle.In this article we will study various methods to calculate the area of the hexagon
Where ‘s’ is the sides of the hexagon
Types of Hexagon
Types of hexagons can be classified as follows:
Regular Hexagon
Irregular Hexagon
In the case of a regular hexagon, all the sides are of equal length and the internal angles are of the same value.Whereas in the case of irregular hexagons, neither the sides are equal, nor the angles are the same.
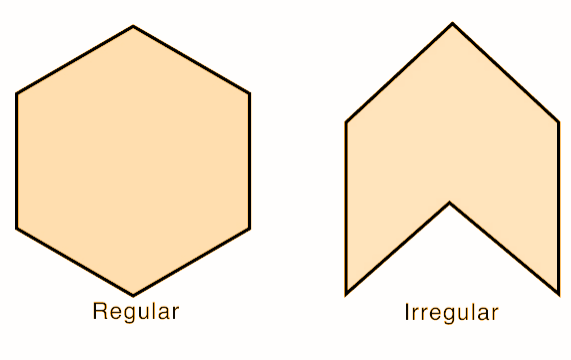
Regular and Irregular Hexagon Diagram
How to Find Area of Hexagon?
There are several ways to find the area of a hexagon.
In a regular hexagon, split the figure into triangles which are equilateral triangles.
Find the area of one triangle.
Multiply this value by six.
Alternatively, the area can be found by calculating one-half of the side length times the apothem.
Formula for the Area of a Hexagon
The area of Hexagon formula is given by
Area of Hexagon = $\dfrac{3\sqrt{3}}{2}\times{s^2}$
where “s” denotes the sides of the hexagon.
Second area of regular hexagon formula is given by:
Area of Hexagon = $\dfrac{3}{2}\times{s}\times{h}
Where “s” is the length of each side and “h” is the height of the hexagon when it is made to lie on one of the bases of it.
Method 1
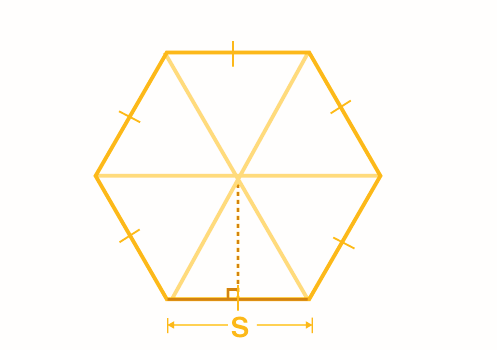
One of the easiest methods that can be used to find the area of a polygon is to split the figure into triangles. Let's start by splitting the hexagon into six triangles.
In this figure, the center point,x, is equidistant from all of the vertices. As a result, the six dotted lines you can say diagonals within the hexagon are of the same length. Likewise, all of the triangles within the hexagon are congruent by the side-side-side rule: each of the triangle's share two sides inside the hexagon as well as a base side that makes up the perimeter of the hexagon. In a similar fashion, each of the triangles have the same angles. There are
3600 in a circle and the hexagon in our image has separated it into six equal parts; therefore, we can write the following:
θ=3600/6
θ=600
We also know the following:
∠AXB=∠BXC=∠CXD=∠DXE=∠EXF=∠FXA=600
Now, let's look at each of the triangles in the hexagon. We know that each triangle has two two sides that are equal; therefore, each of the base angles of each triangle must be the same. We know that a triangle has 180 and we can solve for the two base angles of each triangle using this information.
2x + 600 = 1800
2x = 1200
x = 600
Each angle in the triangle equals 600
We now know that all the triangles are congruent and equilateral: each triangle has three equal side lengths and three equal angles. Now, we can use this vital information to solve for the hexagon's area. If we find the area of one of the triangles, then we can multiply it by six in order to calculate the area of the entire figure.
We have, Area of equilateral triangle = (√3/4) x s x s
So, Area of hexagon = 6 x (√3/4) x s x s
+= 3 x (√3/2) x s x s
Area of Hexagon = 3 √3/2 x s2
Method 2
If we divide the hexagon into two isosceles triangles and one rectangle, then we can show that the area of the isosceles triangles are (1/4)th of the rectangle whose area is l*h.
So, area of regular Hexagon formula is given by:
Area= (3/2) x h x s
Where “s” is the length of each side and “h” is the height of the hexagon when it is made to lie on one of the bases of it.
Solved Examples
Example 1: Find the area of a regular hexagon whose side is 7 cm.
Solution:
Formula of regular hexagon is = 3√3/ 2 x s2
where, s = side = 7 cm
Substitute the value of side in area formula,
Area = (3 x 1.7320) / 2 x 7 x 7
= 127. 31 cm2.
Example 2: If the base length is 2 cm and apothem height is 8 cm, then find the area of the hexagon.
Solution: Formula of regular hexagon = (3/2) × s × h
Here apothem height is given so we need to multiply it by 2 and substituting the value in the above formula, we get
= (3/2) × 2 × (8*2)
= 48cm2.
JEE Main Maths Study and Practice Materials
Explore an array of resources in the JEE Main Maths Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Maths preparation.
JEE Main 2025 Subject-Wise Important Formulas
The JEE Main 2025 subject-wise important formulas provide a focused strategy for Chemistry, Physics, and Maths. These formulas help students prioritise revision in their preparation, ensuring better performance in the exam.
Conclusion
Mastering the area of geometric shapes, such as the hexagon, is essential for excelling in JEE and other competitive exams. Understanding the underlying properties, such as the number of sides and internal angles, allows you to apply the correct formulas and solve problems with confidence. Regular practice and application of these concepts will not only help you perform well in exams but also strengthen your overall problem-solving skills in mathematics. With the right approach, you can easily tackle questions related to areas, shapes, and trigonometric calculations in competitive exams.
JEE Main 2025 Subject-Wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Check Other Important Links for JEE Main 2025
JEE 2025 needs well-structured study materials. Here are some valuable resources to help you plan your preparation and practice efficiently.
FAQs on JEE Main 2025 Maths: Area of Hexagon Formula
1. What is Polygon?
A polygon is a closed geometric figure that consists of straight line segments connected end-to-end. These line segments are called sides, and the points where two sides meet are called vertices (singular: vertex). A polygon can have any number of sides, but it must have at least three sides to be considered a polygon.
Types of Polygons:
Triangle: A polygon with 3 sides.
Quadrilateral: A polygon with 4 sides.
Pentagon: A polygon with 5 sides.
Hexagon: A polygon with 6 sides.
Heptagon: A polygon with 7 sides.
Octagon: A polygon with 8 sides.
Polygons can be regular (all sides and angles are equal) or irregular (sides and/or angles are not equal). The sum of the interior angles of a polygon depends on the number of sides it has.
2. State some of the popular polygons along with its number of sides and Measure of Interior Angles.
Here is a table listing some popular polygons, along with their number of sides and the measure of their interior angles:
Polygon | Number of Sides | Measure of Interior Angles |
Triangle | 3 | $180^\circ$ (Sum of all interior angles) |
Quadrilateral | 4 | $360^\circ$ (Sum of all interior angles) |
Pentagon | 5 | $540^\circ$ (Sum of all interior angles) |
Hexagon | 6 | $720^\circ$(Sum of all interior angles) |
Heptagon | 7 | $900^\circ$ (Sum of all interior angles) |
Octagon | 8 | $1080^\circ$ (Sum of all interior angles) |
Nonagon | 9 | $1260^\circ$ (Sum of all interior angles) |
Decagon | 10 | $1440^\circ$ (Sum of all interior angles) |
3. What is the area of a hexagon?
The area of a hexagon is the space enclosed within its six sides. For a regular hexagon, the area can be calculated using the formula:
$\text{Area} = \frac{3\sqrt{3}}{2} \times a^2$
where aa is the length of a side. For irregular hexagons, different methods may be used depending on the known dimensions.
4. How do you derive the area of a hexagon formula?
The area of hexagon formula derivation is based on dividing the hexagon into 6 equilateral triangles. The area of each triangle is $\frac{\sqrt{3}}{4} \times a^2$, and since there are 6 triangles, the total area becomes:
$\text{Area}=6 \times \frac{\sqrt{3}}{4} \times a^2=\frac{3\sqrt{3}}{2} \times a^2$
5. How To Calculate Area Of Hexagon with a given side length?
To find the area of a regular hexagon with a given side length aa, use the formula:
$\text{Area} = \frac{3\sqrt{3}}{2} \times a^2$
Simply square the length of the side and multiply by the constant.
6. How to calculate the area of a hexagon using the apothem?
If the apothem $a_p$ (the distance from the center to the midpoint of a side) is known, the area of the hexagon can be calculated by the formula:
$\text{Area} = \frac{1}{2} \times Perimeter \times Apothem$
The perimeter of a regular hexagon is $6 \times a$, where a is the side length.
7. How to calculate the area of a hexagon if you know the perimeter?
If you know the perimeter of a regular hexagon, $P = 6a$, the area can be calculated using the formula:
$\text{Area} = \frac{1}{2} \times P \times a_p$
where $a_p$ is the apothem.
8. What is the formula for the area of an irregular hexagon?
For an irregular hexagon, the area is calculated by dividing the hexagon into simpler shapes (such as triangles or rectangles) and calculating their individual areas. Alternatively, you can use the shoelace theorem for coordinates of vertices.
9. Can I use the same area formula for any type of hexagon?
No, the formula $\frac{3\sqrt{3}}{2} \times a^2$ is only applicable to regular hexagons. For irregular hexagons, the area formula depends on the specific dimensions or angles of the hexagon.
10. What is the relationship between the side length and the area of a hexagon?
The area of a regular hexagon increases with the square of the side length. As the side length a doubles, the area increases by a factor of four.
