Work, Energy and Power Solutions for ICSE Board Class 10 Physics

EXERCISE- 2 (A)

1. Define work. When is work said to be done by a force?

Ans: In physics, work is the displacement that occurred in an object over a distance after applying force. Work is a scalar quantity; S.I. the unit of work is Joule. The mathematical formula for work is ${\text{W = }}{\text{fd}}$ (Where W is work, f is the force, and d is the distance)

Work is said to be done when external force displaces an object.

2. How is the work done by a force measured when 

(i) force is in the direction of displacement, 

Ans: $W=f\times d$ is when force is in the direction of displacement

(ii) force is at an angle θ  to the direction of displacement?

Ans: W=fs cos θ when force is at an angle θ to the direction of displacement

3. A force F acts on a body and displaces it by a distance S in a direction at an angle θ  with the direction of force. 

(a) Write the expression for the work done by the force. 

Ans: W= FS cosθ for work done by force at θ to the direction of displacement.

(b) What should be angle between the force and displacement so that the work done is (i) zero, (ii) maximum?

Ans: (i) There should be a $90^{\circ}\left(\cos 90^{\circ}=0\right)$ angle between the force and displacement at an angle $\theta$ with the direction of force, for zero work done.

$\mathrm{W}=\mathrm{FS} \cos 90^{\circ}$

$=\mathrm{FS} \times 90^{\circ}$

$=0$

(ii) There should be $0^{\circ}\left(\cos 0^{\circ}=1\right)$ angle between the force and displacement at an angle $\theta$ with the direction of force, for maximum work done.

Therefore, $W=F S \cos 0^{\circ}=F S$

4. A body is acted upon by a force. State two conditions when the work done is zero. 

Ans: The two conditions are: 

1. When  S = 0  i.e. no force or displacement 

2. When ${\theta  = {90}^\circ }$ i.e. displacement is normal to the direction of the force.

5. State the condition when the work done by a force is (a) positive. (b) negative. Explain with the help of examples.

Ans: (i) Work done is said to be positive when the displacement of the body is in the direction of the force. 

Thus, W = F × S

Example:  When you push a table then the force applied by you and the displacement of the table are in the same direction, so work done is said to be positive.

(ii) Work done is said to be negative, when the displacement of the body is in the opposite direction to the force.

Thus, W = -F × S

Example:  When a child throws a ball upward with a force, the ball moves to a height (h).  But the displacement is in the opposite direction of the force of gravity.

6. A body is moved in a direction opposite to the direction of force acting on it. State, whether the work is done by the force or work, is done against the force.

Ans: When the displacement of the body is in the opposite direction to the force, work done is said to be negative. Therefore, work done is against the force.

7. When a body moves in a circular path, how much work is done by the body? Give reason. (Hint : The body is acted upon by the centripetal force)

Ans: No work is considered to be done by a body moving on a circular path. Because the force of the body is controlled by the center of the circular path, while the displacement at all instances is along the tangent to the circular path, i.e. normal to the direction of the force.

8. A satellite revolves around the earth in a circular orbit. What is the work done by the satellite? Give reasons.

Ans: If the force and displacement are perpendicular to each other then the work done is said to be zero. When a satellite revolves around the earth in a circular orbit the work done is zero.

9. State whether work is done or not, by writing yes or no, in the following cases: 

a. A man pushes a wall.

Ans: No work is done because there is no displacement

b. A coolie stands with a box on his head for 15 minutes.

Ans: No work is done because there is no displacement

c. A boy climbs up 20 stairs.

Ans: Yes, the work is done.

10. A coolie X carrying a load on his head climbs up a slope and another coolie Y carrying identical load on his head moves the same distance on a frictionless horizontal platform. Who does more work? Explain the reason.

Ans: Work done in case of coolie X (by assuming the angle of inclination/slope as $\theta$)

\[X=F\times S\ \text{Cos}\theta \]

Work done in case of coolie Y (The angle between force and displacement is 90 degree on a horizontal platform) 

Y = F × S Cos 90°

Y = F × S × 0

Y = 0

Therefore, coolie X does more work than zero i.e. coolie Y

11. The work done by a fielder when he takes a catch in a cricket match is negative. Explain.

Ans: The force applied by a fielder is in the opposite direction to the displacement of the ball. Therefore the work done by a fielder is negative.

12. Give an example when work done by the force of gravity acting on a body is zero even though the body gets displaced from its initial position.

Ans: Example- A coolie moving on a horizontal ground carrying loads. The displacement in the horizontal direction and the force of gravity acts vertically downward. So, in this case the work done by the force of gravity is zero even though the body gets displaced from its initial position.

13. What are the S.I. and C.G.S units of work?  How are they related? Establish the relationship.

Ans: S.I unit of work is Joule.

C.G.S unit of work is erg.

Relation between joule and erg:

1 joule $=1 \mathrm{~N}\times1 \mathrm{~m}$

But $1 \mathrm{~N}=10^{5} \mathrm{dyne}$

And $1 \mathrm{~m}=100 \mathrm{~cm}=10^{2} \mathrm{~cm}$

Hence, 1 joule $=10^{5} \mathrm{dyne}\times10^{2} \mathrm{~cm}$

$=10^{7} \mathrm{dyne}\times\mathrm{cm}=10^{7} \mathrm{erg}$

Thus, 1 Joule $=10^{7}$ erg

14. State and define the S.I. unit of work.

Ans:  The S.I unit of work is Joule.

1 joule of work is said to be done when a force of 1 newton displaces a body through a distance of 1 metre in its own direction. 

15.  Express joule in terms of erg

Ans:  Joule is a derived unit of energy. 

$1 \mathrm{~J}=10^{7} \mathrm{erg}$

Explanation: 1 Joule $=1 \mathrm{~N} \times 1 \mathrm{~m}$

But, as we already know $1 \mathrm{~N}=10^{5} \mathrm{dyne}$

and $1 \mathrm{~m}=10^{2} \mathrm{~cm}$

Therefore, 1 Joule $=10^{5} \mathrm{dyne} \times 10^{2} \mathrm{~cm}$

1 Joule $=10^{7}$ dyne $\mathrm{cm}$

So, 1 Joule = $=10^{7}$  erg.

16. A body of mass m falls down through a height h. Obtain an expression for the work done by the force of gravity

Ans:  Work done = Force × Displacement

However, the mass of the body is = m

so the force of the gravity will be =  mg

and the displacement, S = h

Therefore, W = FS

 W = mgh

17. A boy of mass m climbs up the stairs of vertical height h. 

a. What is the work done by the boy against the force of gravity? 

Ans:  Mass of the boy is = m

so the force of the gravity will be =  mg

and the displacement, S = h

Therefore, W = FS = mgh is the work done by the boy against the force of gravity.

b. What would have been the work done if he uses a lift in climbing the same vertical height?

Ans: When the boy uses lift the work done will be the same as the boy is not doing the work but the lift is. Therefore, W = FS = mgh

18. Define the term energy and state its S.I. unit.

Ans: Energy can be defined as the capacity of the body to do work.

S.I. the unit of energy is Joule (J).

19. What physical quantity does the electron volt (eV) measure? How is it related to the S.I. unit of that quantity?

Ans: The electron volt (eV)measures electrical Energy.

1eV = charge on an electron ×1 volt

$1 \mathrm{eV}=1.6 \times 10^{-19}$ coulomb $\times 1$ volt

$1 \mathrm{eV}=1.6 \times 10^{-19} \mathrm{~J}$

The electron volt equals $1.602 \times 10^{-12}$ or $1.602 \times 10^{-19}$ joule.

20. Complete the following sentences:

(a) $1 \mathrm{~J}=$ calorie.

Ans: a. $1 \mathrm{~J}=0.24$ calorie.

(b) $1 \mathrm{kWh}=$ . J

Ans: $1 \mathrm{kWh}=3.6 \times 10^{6} \mathrm{~J}$.

21.  Name the physical quantity which is measured in calorie?  How is it related to the S.I. unit of that quantity?

Ans: Heat energy is measured in Calorie.

1 calorie = 4.18 J 

22. Define kilowatt-hour. How is it related to joule?

Ans: Kilowatt is a unit of power. 1kWh is equal to 1000 watts.

$1 \mathrm{kWh} =(1000 \mathrm{watt}) \times(3600 \mathrm{sec})$

$=36 \times 10^{5} \mathrm{~J}$

$=3.6 \times 10^{6} \mathrm{~J}$

23. Define the term power. State its S.I. unit.

Ans: Power is defined as the rate of doing work in unit time.

The S.I. the unit of power is watt (W).

24. State two factors on which power is spent by a source depends. Explain your answer with examples.

Ans:  Power spent  by a source depends on the following factors:

1. The amount of work done by the source

2. The time taken by the source to do the given work

Example : Let’s suppose worker A takes 5 minutes to lift a load upstairs and worker B takes 10 minutes to lift the same load upstairs. The work done by both the workers remains the same, but power spent by worker A is twice the power spent by worker B. As worker A does the work faster than worker B.

25. Differentiate between work and power.

Ans: The difference between work and power is: 

26. Differentiate between energy and power.

Ans: The difference between energy and power. 

27.  State and define the S.I. unit of power.

Ans: The S.I. unit of power is watt (W)

The power of body is 1W if it does work at the rate of 1J/S

28.  (a) Name the physical quantity measured in terms of horsepower.

Ans:  Power is the physical quantity measured in horsepower

(b) How is horsepower related to S.I. unit of power?

Ans: Horsepower is related to S.I. unit as 

1 H. P. = 746 W

29. Differentiate between watt and watt hour.

Ans: Watt is a unit of power i.e. the rate at which work is performed while watt-hour is the unit of work.

30. Name the quantity which is measured in

(a) kWh 

Ans: Energy is measured in kWh

(b) kW

Ans: Power is measured in kW

(c) Wh

Ans: Energy is measured in Wh

(d) eV

Ans: Energy is measured in eV

(Energy is measured in bigger and smaller units such as kWh, kW.

The energy of atomic particles is measured in eV (electron volt))

31. Is it possible that no transfer of energy takes place even when a force is applied to a body?

Ans: Yes, if the force and displacement are perpendicular to each other then there is no transfer of energy.

Multiple Choice Type

1.  One horse power is equal to: 

(a)1000 W (b) 500 W (c) 764 W (d) 746 W.

Ans: (d) 746 W

2. kWh is unit of : 

(a)power (b) force (c) energy (d) none of these.

Ans: (c) energy

Numericals

1. A body, when acted upon by a force 10 kgf, gets displaced by 0.5 m. Calculate the work done by the force, when the displacement is 

(i) in the direction of force, 

Ans:  Given,

Force = 10 kgf , g = 10N per kg

Therefore force acting on the body = 10× 10N =100N 

As we already know Work done = force × displacement in the direction of force

W = F × S

W = 100 × 0.5= 50J

Work done in the direction of force is 50J

(ii) Work = force $x$ displacement in the direction of force

Ans: $W=F\times S \cos {\theta}$

$W=100\times0.5 \cos 60^{\circ}$

$W=100\times0.5\times0.5\left(\cos 60^{\circ}=0.5\right)$

$W=25 \mathrm{~J}$

(iii) Normal to the force:

Ans: Work = force $x$ displacement in the direction of force

$W=F\times S \cos {\theta}$

$W=100\times0.5 \cos 90^{\circ}$

$W=100\times{0.5}\times0=0 \mathrm{~J}\left(\cos 90^{\circ}=0\right)$

Work done is 0 when the displacement is normal to the force.

2. A boy of mass 40 kg climbs up the stairs and reaches the roof at a height of 8 m and 5 s. Calculate : 

(i) the force of gravity acting on the boy.

(Take g = 10 m s-2)

Ans:  Given, Mass of boy = 40kg

Height moved = 8m, Time = 5s

The force of gravity acting on the boy = F = mg

= 40 × 10

=400N

The force of gravity acting on the boy is 400N

(ii) the work done by him against the force of gravity,

Ans:  The work done by him against the force of gravity while climbing,

Work done by the boy in climbing = Force × distance moved in the direction

W = F × S

=400 × 8

=3200J

The work done by him against the force of gravity is 3200J

(iii) the power spent by the boy.

Ans: The power spent by the boy 

$=\dfrac{\text { Work done }}{\text { time }}=\dfrac{3200}{5}=640 \mathrm{~W}$

3. A man spends 6.4 kJ energy in displacing a body by 64 m in the direction in which he applies force, in 2.5 s. 

Calculate : (i) the force applied, and 

Ans: Given, Work done=6.4 kJ

S=64m, t=2.5s

The force applied W = F × S

$\text { Force }=\dfrac{\text { Work done }}{\text { distance }}$

$=\dfrac{6.4 \times 10^{-3}}{64}$

$\mathrm{~F}=100 \mathrm{~N}$

(ii) the power spent (in H.P.) by the man.

Ans: The power spent by the man

$\text { Power spent }=\dfrac{\text { Work done }}{\text { time taken }}$

$=\dfrac{6.4 \times 10^{3}}{2.5}$

$\mathrm{P}=2560 \mathrm{~W}$

Now to convert Watt into Horsepower

As we know $1 \mathrm{H} . \mathrm{P} .=746 \mathrm{~W}$

$1 \mathrm{~W}=\dfrac{1}{746} \mathrm{H} . \mathrm{P}$

$2560 \mathrm{~W}=\dfrac{2560}{746}$

$=256 \mathrm{~W}$

$=3.43 \mathrm{H} . \mathrm{P}$

The power spent by the man is $3.43$ Horsepower

 4. A weight lifter lifted a load of 200 kgf to a height of 2.5 m in 5s. 

Calculate : (i) the work done, and 

Ans: Given, m=200

s=2.5 m, t=5s

$g=10 \mathrm{~N} / \mathrm{kg}^{-1}$

The work done, Force $=$ mass $x$ gravity

$F=200 \times 10$

$=2000 \mathrm{~N}$

We know that, $\mathrm{W}=\mathrm{F} \times \mathrm{S}$

$\mathrm{W}=2000 \times 2.5$

$=5000 \mathrm{~J}$

The work done is $5000 \mathrm{~J}$

(ii) the power developed by him. Take $g=10 \mathrm{~N} / \mathrm{kg}^{-1}$

Ans: The power developed by him 

$\text { Power }=\dfrac{\text { work }}{\text { time }}$

$\mathrm{P}=\dfrac{5000}{5}$

$=1000 \mathrm{~W}$

The power developed by him is 1000W

5. A machine gun raises a load of 750 N through a height of 16m in 5s

Calculate: i. the energy spent by the machine.

Ans: Given, F=750 N

Height/distance moved=16m

time=5s

Energy spent by machine =F×S

i.e. Work done = 750×16

W=12000J

ii. the power of the machine if it is 100% efficient

Ans: The energy spent by machine is 12000J

The power of the machine if it is 100% efficient

$\text { Power }=\dfrac{\text { work }}{\text { time }}$

$\mathrm{P}=\dfrac{12000}{5}$

$=2400 \mathrm{~W}$

6. An electric heater of power 3kW is used for 10h. How much energy does it consume? 

Express your answer in 

(i) kWh 

Ans: As we already know 

Power = Work × Time

Energy consumed = power × time

Energy = 3kW × 10h

=30kWh

(ii) joule

Ans: Energy consumed in joules

1 kilowatt hour (kWh) = 3.6 × 106 J

30kWh = 30 × 3.6 × 106 J

=1.08 × 106 J

7. A water pump raises 50 litres of water through a height of 25m in 5s. Calculate the power of the pump required 

(Take $g=10 \mathrm{~N} \mathrm{~kg}^{-1}$ 

and density of water $=1000 \mathrm{~kg}\mathrm{~m}^{-3} \text { ) }$

Ans: Given, $\mathrm{m}=50 \mathrm{~kg}$

$\mathrm{~g}=10 \mathrm{~N} \mathrm{~kg}^{-1}$

$\mathrm{~d}=25 \mathrm{~m}, \mathrm{t}=5 \mathrm{~s}$

Volume of water $=50 \mathrm{~L}=50 \times 10^{-3} \mathrm{~m}^{3}$

Density of water $=1000 \mathrm{kgm}^{-3}$

Mass of water = Volume of water  $\times$ density of water

$=50 \times 10^{-3} \times 1000=50 \mathrm{~kg}$

Work done in raising 50kg water to a height 25m

against the force of gravity 

W=mg×h

W=mgh

The power of the pump required 

$\mathrm{P}=\dfrac{\text { Work done }}{\text { time taken }}$

$\mathrm{P}=\dfrac{\mathrm{mgh}}{\mathrm{t}}$

$\mathrm{P}=\dfrac{50 \times 10 \times 25 \mathrm{~J}}{5 \mathrm{~s}}$

$\Rightarrow 2500 \mathrm{~W}$

8. A pump is used to lift 500 kg of water from a depth of 80m in 10s. 

Calculate : a. The work done by the pump,

$\left(\text { Take } g=m s^{-2}\right)$

$\text { Hint: Efficiency }=\dfrac{\text { useful power }}{\text { power input }}$

Ans: Work done in raising a 500kg mass to a height of 80m against the force of gravity is : W = mgh

W = 500 × 10 × 80

$W=4 \times 10^{5} \mathrm{~J}$

The work done by pump is $4 \times 10^{5} \mathrm{~J}$

b. The power at which the pump works, and

Ans: Power at which pump works 

$\mathrm{P}=\dfrac{\text { Work done }}{\text { time taken }}=\dfrac{\mathrm{mgh}}{\mathrm{t}}$

$\mathrm{P}=\dfrac{50 \times 10 \times 80 \mathrm{~J}}{10 \mathrm{~s}}$

$\mathrm{P}=\dfrac{4 \times 10^{5}}{10}$

$\mathrm{P}=40 \mathrm{KW}$

c. The power rating of the pump if its efficiency is $40 \%$

Ans: Efficiency $=\dfrac{\text { usefulpower }}{\text { powerinput }}$

Efficiency $=40 \%=0.4$

$0.4=\dfrac{40 \mathrm{KW}}{\text { PowerInput }}$ 

PowerInput $=\dfrac{40 \mathrm{KW}}{0.4}$ 

PowerInput $=100 \mathrm{kw}$

The power rating of the pump if its efficiency is 40% is 100kw

9. An ox can apply a maximum force of 1000 N. It is taking part in a cart race and is able to pull the cart at constant speed of 30 m s -1 while making its best efforts. Calculate the power developed by the ox.

Ans: Given, force=1000N

velocity = 30m/s

As we know,  

Power = force × velocity

P = 1000 × 30

P = 30,000W

P = 30kW

The power developed by the ox is 30kW

10. The power of a motor is 40 kW. At what speed can the motor raise a load of 20,000 N?

Ans: Given, Power = 40kW

Force=20,000N

As we know, Power = force × velocity

$\text { Velocity }=\dfrac{\text { power }}{\text { force }}$

$\text { Velocity }=\dfrac{40 \mathrm{~kW}}{20000}=\dfrac{40000}{20000}$

$\text { Velocity }=2 \mathrm{~m} / \mathrm{s}$

At the speed 2m/s the motor can raise a load of 20,000 N.

11. Rajan exerts a force of $150 \mathrm{~N}$ in pulling a cart at a constant speed of $10 \mathrm{~m} \mathrm{~s}^{-1}$. Calculate the power exerted.

Ans: Given, Pulling force $\mathrm{F}=150 \mathrm{~N}$

Pulling Speed V $=10 \mathrm{~m} / \mathrm{s}$

Power $=$ Force $\times$ Speed

$P=150 \times 10$

$P=1500 \mathrm{~W}$

$1500 \mathrm{~W}$ is the required power exerted.

12. A boy weighing 350 N climbs up 30 steps, each 20 cm high in 1 minute. 

Calculate: (i) Work done, and 

Ans: Given, 

Force (F) = 350N

Time (T) = 1 min = 60s

The distance covered in 30 steps i.e. Height = Height of one step × no. of steps

Height = 0.2 × 30

= 6m

Work done = F × S

Work = F × S

W = 350 × 6

W= 2100J

Work done is 2100J against gravity.

(ii) the power spent.

Ans: The power spent by the boy = 35 W.

13. It takes 20 s for A person of mass 50 kg to climb up the stairs, while another person B of the same mass does the same in 15 s.

Compare the (i) work done and 

Ans: As we already know work done =force × displacement

It is independent of time

Therefore, ratio of work done by person A and person B will be 1:1.

(ii) power developed by the person A and B.

Ans: The power developed by person A and person B

Person A takes 20s to climb up the stairs

Person B takes 15s to climb up the stairs

Work done is same by both 

Therefore, more power is spent by person B, as B does the work faster.

$\dfrac{\text { Power developed by A }}{\text { Power deveoped by B }}=\dfrac{15}{20}=3: 4$

Power developed by person A and person B is in the ratio $3: 4$

14. A boy doing $40 \mathrm{kgf}$ climbs up a stair of 30 steps each $20 \mathrm{~cm}$ high in 4 minutes and a girl weighing $30 \mathrm{kgf}$ does the same in 3 minutes.

Compare : (i) the work done by them, and

Ans: Force of gravity of the boy, $F_{b}=40 \mathrm{~kg}$

Time taken by him, tb $=4$ minutes $=4 \times 60 \mathrm{~s}=240 \mathrm{~s}$

Force of gravity of the girl, Fg $=30 \mathrm{kgf}$

Time taken by her, $\operatorname{tg}=3$ minutes $=3 \times 60 \mathrm{~s}=180 \mathrm{~s}$

Distance covered by both in 30 steps is

$D=30 \times 20=600 \mathrm{~cm}$

While climbing, both have to do work against the force of gravity.

Work done by the boy in climbing the stairs:

$W_{b}=F \times D=40 \mathrm{~kg} \times 600 \mathrm{~cm}$

$W_{b}=24000 \mathrm{~J}$

Work done by the girl in climbing the stairs:

$W_{g}=F \times D=30 \mathrm{~kg} \times 600 \mathrm{~cm}$

$W_{g}=18000 \mathrm{~J}$

$\rightarrow \dfrac{W_{b}}{W_{g}}=\dfrac{24000 \mathrm{~J}}{18000} J$

$\therefore \dfrac{W_{b}}{W_{g}}=\dfrac{4}{3}$

(ii) the power developed by them.

Ans: Power developed $=\dfrac{\text { Work done }}{\text { Time Taken }}$

For the boy:

Power developed $=\dfrac{24000 \mathrm{~J}}{240 \mathrm{~s}}=100 \mathrm{~W}$

For the girl:

Power developed $=\dfrac{18000 J}{180 s}=100 W$

Thus, power developed by them is $1: 1$

15. A man raises a box of mass 50 kg to a height of 2 m in 20 s, while another man raises the same box to the same height in 50 s. 

(a) Compare : (i) the work done, and 

(Take $g=10Nkg \mathrm{~kg}^{-1}$ )

Ans: Work done = mg × h

Both men did the same work here

Therefore, $\dfrac{\text { Work done by A }}{\text { Work done by B }}=\dfrac{\mathrm{mgh}}{\mathrm{mgh}}=\dfrac{50 \times 10 \times 2}{50 \times 10 \times 2}$

$=\dfrac{1000}{1000}$

$=\dfrac{1}{1}$

$\Rightarrow \dfrac{{\text{1}}}{{\text{1}}}{\text{ = 1:1}}$

(ii) the power developed by them.

Ans: The power developed by them

Work done is same but the power developed by them is 50:20= 5:2

(b) Calculate : (i) the work done and 

Work done by 1st man =1000J

Work done by 2nd man =1000J

(ii) the power developed by each man.

Power developed by A =50W

Power developed by B=20W

16. A boy takes 3 minutes to lift a 20 litre water bucket from of 20 m deep well, while his father does it in 2 minutes.

(a) Compare : (i) the work, and 

Ans: Given,

Density of water $=10^{3} \mathrm{~kg} \mathrm{~m}^{-3}$

$g=9.8 \mathrm{~N} \mathrm{~kg}^{-1}$

Time taken by boy =3 min=180s

Time taken by father=2min=120s

Work done=Force × displacement

W=mgh

But here the weight and the distance covered by both are the same.

Therefore work done will be the same 

Work done by the boy and father will be 3920J each

Ratio of work done by boy and work done by father will be 1:1

(ii) power developed by them.

Ans: Power developed by a boy 

$=\dfrac{\text { work done }}{\text { time taken by the boy }}=\dfrac{3920}{3} \times 60=21.78 \mathrm{~W}$

Power developed by the father:

$=\dfrac{\text { work done }}{\text { time taken by the father }}=\dfrac{3920}{2} \times 60=32.67 \mathrm{~W}$

(b) How much work each does? 

( Take density of water $=10^{3} \mathrm{~kg} \mathrm{~m}^{-3}$ and $g=9.8 \mathrm{~N} \mathrm{~kg}^{-1}$)

Ans:  Work done in both cases=20×9.8×20

=400×9.8

=3920J

EXERCISE - 2(B)

1. What are the two forms of mechanical energy?

Ans: The two forms of mechanical energy are:

1. Kinetic energy

2. Potential energy

2. Name the forms of energy which a wound up watch spring possesses.

Ans: A wound-up watch spring possesses Elastic potential energy. The potential energy changes into kinetic energy while unwinding of spring.

3. Name the type of energy (kinetic energy K or potential energy U) possessed in the following cases: 

1. A moving cricket ball 

2. A compressed spring

3. A moving bus

4. A stretched wire 

5. An arrow shot out of a bow

6. A piece of stone placed on the roof 

Ans:

1. A moving cricket ball –Kinetic energy (K)

2. Compressed spring-Potential energy (U)

3. A moving bus – Kinetic energy (K)

4. A stretched wire – Potential energy (U)

5. An arrow shot out of a bow – Kinetic energy (K)

6. A piece of stone placed on the roof – Potential energy (U)

4. Define the term potential energy of a body. Name its two forms and give one example of each.

Ans: The energy possessed by a body virtue of its position or size and shape is called the potential energy.

Forms of the energy 

i) A spring has elastic potential energy due to its compressed state.

ii) A stone at a height has gravitational potential energy due to its raised height.

5. Name the forms of energy that a body may possess even when it is not in motion. Give an example to support your answer.

Ans: Potential energy is possessed by the body when it is not in motion.

Example: A stone raised at a height has gravitational potential energy.

6. What is meant by gravitational potential energy? Derive an expression for it for a body placed at a height above the ground.

Ans: The potential energy possessed by a body due to its position relative to the center of earth is known as gravitational potential energy. 

For a body placed at a height above the ground, the gravitational potential energy is measured by the amount of work done in lifting it up to that height against the force of gravity.

Therefore, when we lift a body of mass m from the ground to the height h. Then the force of gravity (mg) acts on the body. 

Work done = Force of gravity(mg) × displacement (h)

W=mgh

And the work done on the body is stored in the form of its gravitational potential energy when it is at a height h. 

Hence, Gravitational potential energy U = mgh 

7. Write an expression for the potential energy of a body of mass M placed at a height h above the Earth's surface. State the assumptions made, if any.

Ans: The work done W on the body in lifting it to height h is

Work done = Force of gravity × displacement

W = mgh

This is the work stored in the body in the form of its gravitational potential energy (U)

U=mgh

8. What do you understand by the kinetic energy of a body? 

Ans: Kinetic energy is the energy possessed by the body when it is in motion.

Kinetic energy $=\left[\dfrac{1}{2} \mathrm{mv}^{2}\right]$

9. (a) A body of mass $m$ is moving with a velocity v. Write the expression for its kinetic energy.

Ans: Kinetic energy

$\left[=\dfrac{1}{2} \mathrm{\times mass \times (velocity) }\right]$ Kinetic energy 

$\left[=\dfrac{1}{2} \mathrm{mv}^{2}\right]$

(b) Show that the quantity $2 K / v^{2}$ has the unit of mass, where $\mathrm{k}$ is the kinetic energy of the body.

Ans: Given,

A quantity - $\left[\dfrac{2 \mathrm{~K}}{\mathrm{~V}^{2}}\right]$

Velocity - V, Mass - m

Kinetic energy of the body,

$\left[\mathrm{K}=\dfrac{1}{2} \mathrm{MV}^{2}\right]$

$m$ is the mass of the body,

So,$\left[\Rightarrow\dfrac{2 \mathrm{~K}}{\mathrm{~V}^{2}}= M\right]$

Therefore $\left[\dfrac{2 \mathrm{~K}}{\mathrm{~V}^{2}}\right]$ is supposed to be the mass of the body.

The obtained physical quantity

$\left[\dfrac{2 \mathrm{~K}}{\mathrm{~V}^{2}}\right]$ has S.I. unit $\mathrm{K} . \mathrm{g}$.

Unit of mass is given by $\mathrm{Kg}$.

10. State the work energy theorem.

Ans: The work energy theorem is: The work done by a force on a moving body is equal to the increase in its kinetic energy.

11. A body of mass M is moving with a uniform velocity u. A force is applied on the body due to which its velocity increases from u to v. How much work is being done by the force.

Ans: Given, Mass=M

Force= f

Work done = force× displacement

W=F × S  

From equation of motion, $V^{2}-U^{2}=2 a s$ $\therefore \mathrm{a}=\dfrac{\left(\mathrm{v}^{2}-\mathrm{u}^{2}\right)}{2 \mathrm{~s}}$

As we already know, $\mathrm{F}=\mathrm{ma}$ $\Rightarrow \mathrm{F}=\dfrac{\mathrm{m}\left(\mathrm{v}^{2}-\mathrm{u}^{2}\right)}{2 \mathrm{~s}}$

Work done W=Fs

Thus,

$\mathrm{W}=\dfrac{\mathrm{m}\left(\mathrm{v}^{2}-\mathrm{u}^{2}\right) \mathrm{s}}{2 \mathrm{~s}}$

$\mathrm{~W}=\dfrac{1}{2} \mathrm{~m}\left(\mathrm{v}^{2}-\mathrm{u}^{2}\right)$

W=F s

Thus $W=\dfrac{m\left(v^{2}-u^{2}\right) s}{2 s}$

$W=\dfrac{1}{2} m\left(v^{2}-u^{2}\right)$

This is the work done by the force.

12. A light mass and a heavy mass have equal momentum. Which will have more kinetic energy? Hint : kinetic energy $=p^{2} / 2 m$ where p is the momentum

Ans: Given,

Kinetic energy $=P^{2} / 2 m$ where p is the momentum

Both light mass and a heavy mass have the same momentum $p$.

Kinetic energy $(K)$ is inversely proportional to mass of the body.

Therefore,

Light mass body has more kinetic energy because smaller the mass larger the kinetic energy.

13. Two bodies of A and B of masses m and M (M>>m) have same kinetic energy. Which body will have more momentum? 

Ans: Both of the bodies have same kinetic energy, momentum is directly proportional to the square root of mass.

As we know Kinetic energy and momentum are related as $p=\sqrt{2mk}$.

As given M>>m

The mass of body B is greater than that of body A.

Therefore, body B will have more momentum than body A.

14. Name three forms of kinetic energy and give one example of each. 

Ans: The three forms of kinetic energy are:

(i) Translational kinetic energy.

Ex. A freely falling object

(ii) Rotational kinetic energy.

Ex. A spinning wheel

(iii) Vibrational kinetic energy

Ex. A clamped wire at both ends vibrates when struck.

15. State two differences between potential energy and kinetic energy.

Ans: The difference between potential energy and kinetic energy.

16. Complete the following sentences : 

(a)The kinetic energy of a body is the energy by virtue of its…….

Ans: (a)The kinetic energy of a body is the energy by virtue of its motion.

(b)The potential energy of a body is the energy of virtue of its…….

Ans: (b)The potential energy of a body is the energy of virtue of its position.

17. When an arrow is shot from a bow, it has kinetic energy in it. Explain briefly from where does it get its kinetic energy.

Ans: A stretched bow has potential energy due to the change in its shape.

The bow has to be released, to be shot. Hence, the potential energy in the bow is converted to kinetic energy of the arrow.

18. A ball is placed on a compressed spring. What form of energy does the spring possess? On releasing the spring, the ball flies away. Give a reason.

Ans: The compressed spring has elastic potential energy because of its compressed state. When it is released the potential energy of the spring is changed in the kinetic energy. As a result potential energy is changed into kinetic energy and due to which it flies away.

19. A pebble is thrown up. It goes to a height and then comes back on the ground. State the different changes in form of energy during its motion.

Ans: When a pebble is thrown up, the kinetic energy of the pebble changes into potential energy. At the greatest height the potential energy is maximum and at this point the velocity becomes zero. After this position in mid-air, the pebble starts to travel downwards as a result of gravitational pull. The potential energy slowly decreases as the pebble falls downwards. The potential energy is completely converted into kinetic energy when the pebble reaches the ground.

20. In what way does the temperature of water at the bottom of a waterfall differ from the temperature at the top? Explain the reason.

Ans: There is potential energy in stored water. When water is falling down from the height, the potential energy stored in water changes into kinetic energy. On hitting the ground some part of the kinetic energy changes into heat energy, therefore water temperature is slightly high at the bottom. 

21. Name the form of energy in which potential energy can change. 

Ans: Potential energy can  be changed into Kinetic energy only.

22. Name the form of mechanical energy, which is put to use. 

Ans: Kinetic energy is put to use in the form of mechanical energy. 

23. Name six different forms of energy.

Ans: Six different forms of energy are;

1. Solar energy

2. Light energy

3. Heat energy

4. Hydro energy

5. Nuclear Energy

6. Chemical or Fuel energy

24. Energy can exist in several forms and may change from one form to another. For each of the following, state the energy changes that occur in :

(a) the unwinding of a watch spring, 

(b) a loaded truck when started to set in motion, 

(c) a car going uphill,

(d) photosynthesis in green leaves, 

(e) charging of a battery,

(f) respiration,

(g) burning of a match stick,

(h)explosion of crackers.

Ans: (a) the unwinding of a watch spring- Potential energy of sound of spring converts into kinetic energy

(b) a loaded truck when started to set in motion-Chemical energy of petrol or diesel converts into mechanical energy

(c) a car going uphill-Kinetic energy to potential energy

(d) photosynthesis in green leaves-Light energy changes into chemical energy

(e) charging of a battery-Electrical energy changes into chemical energy

(f) respiration-Chemical energy changes into heat energy

(g) burning of a match stick-Chemical energy changes into heat and light energy

(h)explosion of crackers-Chemical energy changes into heat, light and sound energy 

25. State the energy changes in the following cases while in use :

(a) loudspeaker

(b) a steam engine 

(c) microphone 

(d) washing machine 

(e) a glowing electric bulb 

(f) burning coal 

(g) a solar cell 

(h) bio-gas burner 

(i)an electric cell in a circuit 

(j) a petrol engine of a running car 

(k) an electric iron 

(l) a ceiling fan 

(m) an electromagnet

Ans:

(a) loudspeaker-Electrical energy into sound energy

(b) a steam engine Heat energy into mechanical energy.

(c) microphone –Sound energy into electrical energy

(d) washing machine – Electrical energy into mechanical energy.

(e) a glowing electric bulb – Electrical energy into light energy 

(f) burning coal – Chemical energy into heat energy

(g) a solar cell – Light energy into electrical energy

(h) bio-gas burner – Chemical energy into heat energy

(i)an electric cell in a circuit – Chemical energy into heat energy. 

(j) a petrol engine of a running car – Chemical energy into mechanical energy.

(k) an electric iron – Electrical energy  into heat energy.

(l) a ceiling fan –light energy into electrical energy.

(m) an electromagnet- Electrical energy into magnetic energy.

26. Name the process used for producing electricity from nuclear energy. 

Ans: Electricity is produced from nuclear energy by the process known as nuclear fission.

27. Is it practically possible to convert a form of energy completely into another useful form? Explain your answer. 

Ans: No, it is practically impossible to convert a form of energy completely into another useful form. Some part of energy always dissipates in the form of heat which is lost to the surrounding.

28. What is degraded energy? 

Ans: The degraded energy is the one that is lost to the surrounding in the form of heat at the time of conversion of energy from one form to another.

29. What do you mean by degradation of energy? Explain it by taking an example from your daily life.

Ans: The degraded energy is the one that is lost to the surrounding in the form of heat at the time of conversion of energy from one form to another. There is a gradual decrease of useful energy due to friction and that is known as degradation energy.

Example: When a car runs on fuel i.e. chemical energy a major part is wasted as heat and sound.

30. Complete the sentence:

The conversion of a part of energy into an undesirable form is called….

Ans: The conversion of a part of energy into an undesirable form is called the dissipation of energy/degradation of energy.

Multiple Choice Type

1. A body at the height possess : 

(a) kinetic energy (b) potential energy (c) solar energy (d) heat energy 

Ans: (b) Potential energy

2. In an electric cell while in use, the change in energy is from: 

(a) electrical to mechanical

(b) electrical to chemical 

(c) chemical to mechanical

(d) chemical to electrical

Ans: (d) chemical to electrical

Numericals

1. Two bodies of equal masses are placed at height h and 2h. Find the ratio of their gravitational potential energies.

Ans:  Given,

Height $h_{1}=h$, Height $h_{2}=2 h$

Mass is same for both the bodies $=\mathrm{m}$

Gravitational potential energy of body $1=m g h_{1}=m g h$

Gravitational potential energy of body $2=m g h_{2}=m g(2 h)$

$=\dfrac{\mathrm{mgh}}{\mathrm{mg}(2 \mathrm{~h})}=\dfrac{\mathrm{mgh}}{2 \mathrm{mgh}}=\dfrac{1}{2}$

$\Rightarrow 1: 2$

2. Find the gravitational potential energy of 1 kg mass kept at a height of 5m above the ground. Calculate its kinetic energy when it falls and hits the ground. 

Ans: Given, Mass m=1kg

Height h=5m

Gravitational potential energy =mgh

= 1×10×5

=50J

3. A box of weight 150 kgf has gravitational potential energy stored in it equal to 14700 J. Find the height of the box above the ground.

Ans: Given, Mass m= 150 kgf

Gravitational potential energy=14700J

Force of gravity =mg

150×9.8 N/kg

=1470 N

Gravitational potential energy=mgh

147000=1470×h

h=10m

Therefore height of the box above ground is 10m

4.  A body of mass 5 kg falls from a height of 10 m to 4 m. Calculate:

(i) the loss in potential energy of the body,

Ans: Given, Mass m =5kg

Height $h_1=10 m$, Height $h_2=4m$

 $g = 10 ms^{-2}$

Potential energy at height 10m = 5 × 10 × 10 = 500J

Potential energy at height 4m = 5 × 10 × 4 = 200J

Therefore loss in potential energy is 300 J

(ii) the total energy possessed by the body at any instant? (Take $g = 10 ms^{-2}$).

Ans: The total energy possessed by the body remains constant for free fall

Total energy =Kinetic energy + Potential energy

 Total energy = 0 + 500

Total energy = 500J

5. Calculate the height through which a body of mass 0.5 kg is lifted if the energy spent in doing so is 1.0 J. Take g = 10m/s-2.

Ans: Given, Mass m=0.5kg

Energy= 1 J,  $g = 10 ms^{-2}$

Gravitational potential energy=mgh

1 = 0.5 × 10 × h

1=5h

h=1/5

h=0.2m

6. A boy weighing 25 kg climbs up from the first floor at a height of 3 m above the ground to the third floor at a height of 9 m above the ground. What will be the increase in his gravitational potential energy?

(Take $g=10 \mathrm{~N} \mathrm{~kg}^{-1} \text { ).}$

Ans: Given, Mass m =25 kg, $g=10 \mathrm{~N} \mathrm{~kg}^{-1}$

Force of gravity on boy=mg

$=25 \times 10=250 \mathrm{~N}$

Increase in gravitational potential energy $=M g\left(h_{2}-h_{1}\right)$ $=250 \times(9-3)$

$=250 \times 6$

$=1500 \mathrm{~J}$

Increase in gravitational potential energy will be $1500 \mathrm{~J}$

7. A vessel containing 50 kg of water is placed at a height 15m above the ground. Assuming the gravitational potential energy at the ground to be zero, what will be the gravitational potential energy of water in the vessel? ($g = 10ms{-2}$)

Ans: Given,

Mass m=50kg

Height h=15 m

$g = 10ms^{-2}$

Gravitational potential energy=mgh

=50×10×15

=7500J

Gravitational potential energy of water in the vessel will be 7500J

8. A man of mass 50 kg climbs up a ladder of height 10m. Calculate: (i) the work done by the man, $\left(g=9.8 m s^{-2}\right)$

Ans: Given,

Mass $m=50 \mathrm{~kg}$

Height of the ladder $h_{2}=10 \mathrm{~m}$

Work done by $\mathrm{man}=\mathrm{mgh}_{2}$

$=50 \times 9.8 \times 10$

$=4900 \mathrm{~J}$

(ii) the increase in his potential energy.

Ans: Increase in his potential energy

Height, $\mathrm{h}_{2}=10 \mathrm{~m}$

Ground, $h_{1}=0 \mathrm{~m}$

Gravitational potential energy 

$=\mathrm{Mg}\left(\mathrm{h}_{2}-\mathrm{h}_{1}\right)$

  ${\text{ = 50 }\times{ 9}}{\text{.8 }\times{ (10 - 0)}}$

  ${\text{ = 50 }\times{ 9}}{\text{.8 }\times{ 10}}$

  ${\text{ = 4900}}{\text{J}}$

Increase in his potential energy will be 4900 J

9. A block A, whose weight is 100N, is pulled up a slope of length 5m by means of a constant force F (=100N) as illustrated in fig. 

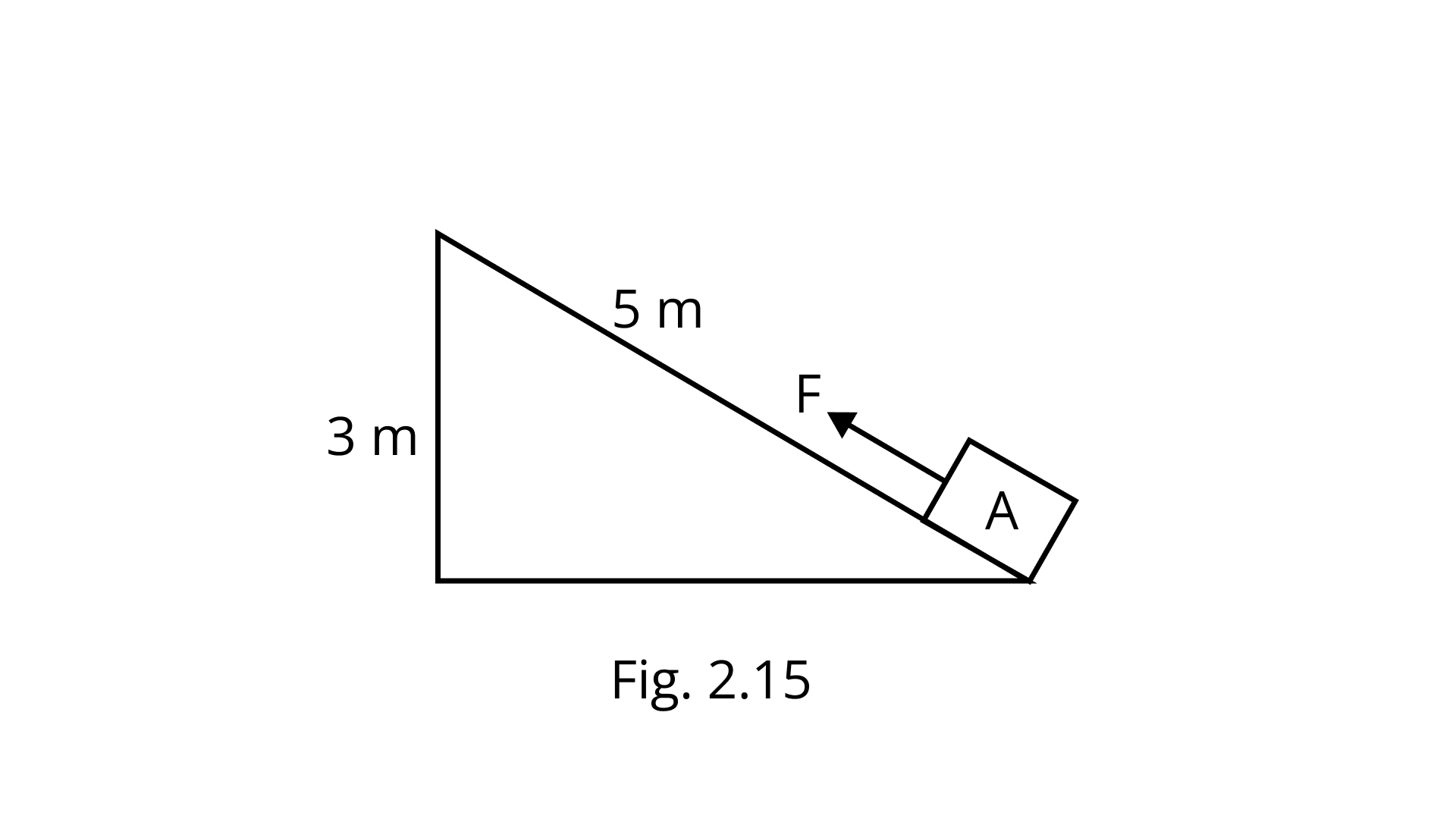
(a) What is the work done by the force F in moving the block A, 5m along the slope?

Ans: Given,

F=100N

Work done by the force in moving the block 5m along the slope =Force x displacement in the direction of force
=100 x 5=500 J.

Work done by the force in moving the block 5m along the slope will be 500 J

(b) What is the increase in potential energy of the block A?

Ans: The potential energy gained by the block U =mgh where h =3m
=100 x 3=300 J
The potential energy gained by the block A will be 300 J 

(c) Account for the difference in the work done by the force and the increase in potential energy of the block.

Ans: The difference in the work done by the force and the increase in potential energy of the block will be 200 J

10. Find the kinetic energy of a body of mass $1 \mathrm{~kg}$ moving with a uniform velocity of $10 m s^{-1}$

Ans: Given,

Mass m =1kg

Velocity v = 10m/s

Kinetic energy $=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$=\dfrac{1}{2} \times 1 \times(10)^{2}$

$=\dfrac{1}{2} \times 1 \times 100$

$=50 \mathrm{~J}$

Kinetic energy of the body will be 50J. 

11. If the speed of a car is halved, how does its kinetic energy change?

Ans: If the speed of a car is halved , the kinetic energy decreases as the mass is the same. It becomes one-fourth since the kinetic energy is proportional to the square of velocity.

12. Calculate the decrease in the kinetic energy of a moving body if its velocity reduces to half of the initial velocity.

Ans: Initial velocity= V

Final velocity $=\mathrm{v}=\dfrac{\mathrm{V}}{2}=\dfrac{\mathrm{V}}{2}$

Initial kinetic energy $=\mathrm{k}_{1}=\dfrac{1}{2} \mathrm{mv}^{2}$ Final kinetic energy $=$

$\mathrm{K}_{2}=\dfrac{1}{2} \mathrm{~m}\left(\dfrac{\mathrm{v}}{2}\right)^{2}$

$\mathrm{~K}_{2}=\dfrac{1}{4} \times \dfrac{1}{2} \mathrm{mv}^{2}$

Change in kinetic energy

$=\dfrac{1}{2} \mathrm{mv}^{2}-\dfrac{1}{4} \times \dfrac{1}{2} \mathrm{mv}^{2}$

$\Rightarrow \dfrac{3}{4} \times \dfrac{1}{2} \mathrm{mv}^{2} \Rightarrow \dfrac{3}{4} \times$ initial K.E.

13. Two bodies of equal masses are moving with uniform velocities v and 2v. Find the ratio of their kinetic energies.

Ans: Given,

Velocity of first body $\mathrm{V}_{1}=\mathrm{V}$

Velocity of second body $\mathrm{V}_{2}=\mathrm{2V}$

Their masses are same, so kinetic energy is directly proportional to the square of the velocity $\left( K\ \alpha \ {{v}^{2}} \right)$

Therefore, ratio of their kinetic energies will be:  

$\dfrac{\mathrm{K}_{1}}{\mathrm{~K}_{2}}=\dfrac{\left(\mathrm{V}_{1}\right)^{2}}{\left(\mathrm{~V}_{2}\right)^{2}}$

$=\dfrac{\mathrm{V}^{2}}{(2 \mathrm{~V})^{2}}$

$=\dfrac{\mathrm{V}^{2}}{4 \mathrm{~V}^{2}}$

$=\dfrac{1}{4}$

=1: 4

The ratio of their kinetic energies will be 1:4.

14. A car is running at a speed of $15 \mathrm{~km} \mathrm{~h}^{-1}$ while another similar car is moving at a speed of $45 \mathrm{~km} \mathrm{~h}^{-1}$. Find the ratio of their kinetic energies.

Ans: Given,

Velocity of first car, $V_{1}=15 \mathrm{~km} \mathrm{~h}^{-1}$

Velocity of second car, $V_{2}=45 \mathrm{~km} \mathrm{~h}^{-1}$

Masses are same,

So kinetic energy is directly proportional to the square of the velocity $\mathrm{KE} \alpha \mathrm{v}^{2}$

Therefore,

$\mathrm{KE}=\mathrm{mv}^{2}$

$\mathrm{KE} \alpha, \mathrm{V}^{2}$

$\dfrac{\mathrm{KE}_{1}}{\mathrm{KE}_{2}}=\dfrac{\mathrm{V}_{1}{ }^{2}}{\mathrm{~V}_{2}{ }^{2}}$

$\dfrac{\mathrm{KE}_{1}}{\mathrm{KE}_{2}}=\left(\dfrac{15}{45}\right)^{2}$

$\dfrac{\mathrm{KE}_{1}}{\mathrm{KE}_{2}}=\left(\dfrac{1}{3}\right)^{2}$

$\dfrac{\mathrm{KE}_{1}}{\mathrm{KE}_{2}}=\dfrac{1}{9}$

$rac{\mathrm{KE}_{1}}{\mathrm{KE}_{2}}=1: 9$

Kinetic energy ratio will be 1:9

15. A ball of mass $0.5 \mathrm{~kg}$ slows down from a speed of $5 \mathrm{~m} / \mathrm{s}^{-1}$ to that of $3 \mathrm{~m} / \mathrm{s}^{-1}$. Calculate the change in kinetic energy of the ball.

Ans: Given,

Mass $m=0.5 \mathrm{~kg}$

Initial velocity $=5 \mathrm{~m} / \mathrm{s}$

Initial Kinetic energy $=1 / 2 \times \operatorname{mass} \times($ velocity $)$

$=\dfrac{1}{2} \times 0.5 \times(5)^{2}$ $=\dfrac{1}{2} \times 0.5 \times 25$ $=6.25 \mathrm{~J}$ 

Final velocity of the ball $3 \mathrm{~m} / \mathrm{s}$ 

Final kinetic energy of the ball $=\dfrac{1}{2} \times$ mass $\times(\text { velocity })^{2}$ 

$=\dfrac{1}{2} \times$ mass $\times(\text { velocity })^{2}$ 

$=\dfrac{1}{2} \times 0.5 \times(3)^{2}$ 

$=\dfrac{1}{2} \times 0.5 \times 9$ $=2.25 \mathrm{~J}$ 

Final kinetic energy of the ball $=\dfrac{1}{2} \times$ mass $\times(\text { velocity })^{2}$

The change in kinetic energy of the ball will be = 2.25J-6.25J

=-4J

Therefore, there will be a decrease in the kinetic energy of the ball.

16. A canon ball of mass $500 \mathrm{~g}$ is fired with a speed of $15 \mathrm{~m} / \mathrm{s}^{-1}$. Find:

(i) its kinetic energy and

Ans: Given,

Mass of canon ball $=500 \mathrm{~g}=0.5 \mathrm{~kg}$

Speed, $v=15 \mathrm{~m} / \mathrm{s}$

Kinetic energy of ball =

$=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$\dfrac{1}{2} \times 0.5 \times(15)^{2}$

$\dfrac{1}{2} \times 0.5 \times 225$

$56.25 \mathrm{~J}$

(ii) its momentum.

Ans: Momentum of the ball = mass × velocity 

= 0.5 × 15 = 7.5 kgm/s 

17. A body of mass $10 \mathrm{~kg}$ is moving with a velocity $20 \mathrm{~m} \mathrm{~s}^{-1}$. If the mass of the body is doubled and its velocity is halved,

find: (i) the initial kinetic energy, and

Ans: Given,

Initial mass $\mathrm{m}_{1}=10 \mathrm{~kg}$, Velocity $\mathrm{v}_{1}=20 \mathrm{~m} / \mathrm{s}$

Final mass $\mathrm{m}_{2}=2 \times 10=20$, Velocity $\mathrm{v}_{2}=20 / 2=10 \mathrm{~m} / \mathrm{s}$

The initial kinetic energy $\mathrm{K}_{1}$

$=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$\dfrac{1}{2} \times 10 \times(20)^{2}$

$\dfrac{1}{2} \times 10 \times 20 \times 20$

$2000 \mathrm{~J}$

(ii) the final kinetic energy.

Ans: Final kinetic energy K2

 $\mathrm{K}_{2}=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$\dfrac{1}{2} \times 20 \times(10)^{2}$

$\dfrac{1}{2} \times 20 \times 10 \times 10$

$1000 \mathrm{~J}$

Therefore, $\dfrac{\mathrm{K}_{1}}{\mathrm{~K}_{2}}=\dfrac{2000}{1000}=\dfrac{2}{1}=2: 1$

18. A truck weighing $1000 \mathrm{kgf}$ changes its speed from $36 \mathrm{~km} / \mathrm{h}^{-1}$ to $72 \mathrm{~km} / \mathrm{h}^{-1}$ in 2 minutes. Calculate:

(i) the work done by the engine and

Ans: Given,

$u=36 \mathrm{~km} / \mathrm{h} \quad \text { i.e. } 10 \mathrm{~m} / \mathrm{s}$

$\mathrm{v}=72 \mathrm{~km} / \mathrm{h} \quad \text { i.e. } 20 \mathrm{~m} / \mathrm{s}$

$\text { mass of truck }=1000 \mathrm{~kg}$

$\mathrm{~g}=10 \mathrm{~m} / \mathrm{s}^{-2}$

$\mathrm{W}=\dfrac{1}{2} \times 1000 \times\left(20^{2}-10^{2}\right)$

$\mathrm{W}=500 \times(400-100)$

$\mathrm{W}=500 \times 300$

$\mathrm{~W}=150000 \mathrm{~J}$

$\mathrm{~W}=1.5 \times 10^{5} \mathrm{~J}$

(ii) its power.

Ans: Power =work done /time taken

$=1.5 \times 10^{5} \mathrm{~J} / 120 \mathrm{~s}$

$=1.25 \times 10^{3} \mathrm{~W}$

19. A body of mass $60 \mathrm{~kg}$ has the momentum $3000 \mathrm{kgm} / \mathrm{s}^{-1}$. Calculate:

(i) the kinetic energy and

Ans: Given,

Mass $=60 \mathrm{~kg}$

Momentum $p=3000 \mathrm{kgm} / \mathrm{s}^{-1}$

Kinetic energy $=\dfrac{\mathrm{p}^{2}}{2 \mathrm{~m}}$ 

$=\dfrac{(3000)^{2}}{2 \times 60}$ 

$=\dfrac{(3000 \times 3000)}{120}$

$=75000 \mathrm{~J}$ 

$=7.5 \times 10^{4} \mathrm{~J}$

(ii) the speed of the body. Ans: Speed of the body Momentum $=$ mass $\times$ velocity 

$3000=60 \times$ velocity Velocity

$=\dfrac{3000}{60}$

Velocity = 50 m/s

20. How much work is needed to be done on a ball of mass 50g to give it a momentum of $5kgms^{-1}$ ?

Ans: Given,

Mass m = 50g = 0.05 kg

Momentum p =500 gcm = 0.005 kgm/s

Kinetic energy of the ball $\dfrac{\mathrm{p}^{2}}{2 \mathrm{~m}}$

$=\dfrac{(0.005)^{2}}{2 \times 0.05}$

$=250 \mathrm{~J}$

$=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$=\dfrac{1}{2} \times 20 \times(3)^{2}$

$=\dfrac{1}{2} \times 20 \times 9$

$=90 \mathrm{~J}$

21. How much energy is gained by a box of mass 20 kg when a man

(a) carrying the box waits for 5 minutes for a bus?

Ans: Given,

Mass m =20kg

Zero displacement hence zero work done

(b) runs carrying the box with a speed of $3ms^{-1}$ to catch the bus?

Ans: Work done, Kinetic energy of man 

(c) raises the box by $0.5 \mathrm{~m}$ in order to place it inside the bus?

Ans: Work done in raising the box, Potential

$\text { energy }=\mathrm{mgh}$

$=\mathrm{U}=20 \times 10 \times 0.5$

$=100 \mathrm{~J}$

22. A bullet of mass 50g is moving with a velocity of $500ms^{-1}$ . It penetrates 10 cm into a still target and comes to rest. Calculate:

(a) the kinetic energy possessed by the bullet, and

Ans: Given

Mass of bullet = 50 g= 0.05 kg

Velocity = 500 m / s

Distance penetrated by the bullet = 10 cm= 0.1 m

(a) The kinetic energy of the bullet=

$=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$=\dfrac{1}{2} \times 0.05 \times(500)^{2}$

$=\dfrac{1}{2} \times 0.05 \times 500 \times 500$

$=6250 \mathrm{~J}$

(b) the average retarding force offered by the target.

Ans: Work done by the bullet against the material of the target = resistance force × distance

6250 = resistance force × 0.1 m

Resistance force = 62500 N

23. A spring is kept compressed by a small trolley of mass 0.5 kg lying on a smooth horizontal surface as shown in the fig. When the trolley is released, it is found to move at a speed of $v = 2 m/s. What potential energy did the spring possess when compressed?

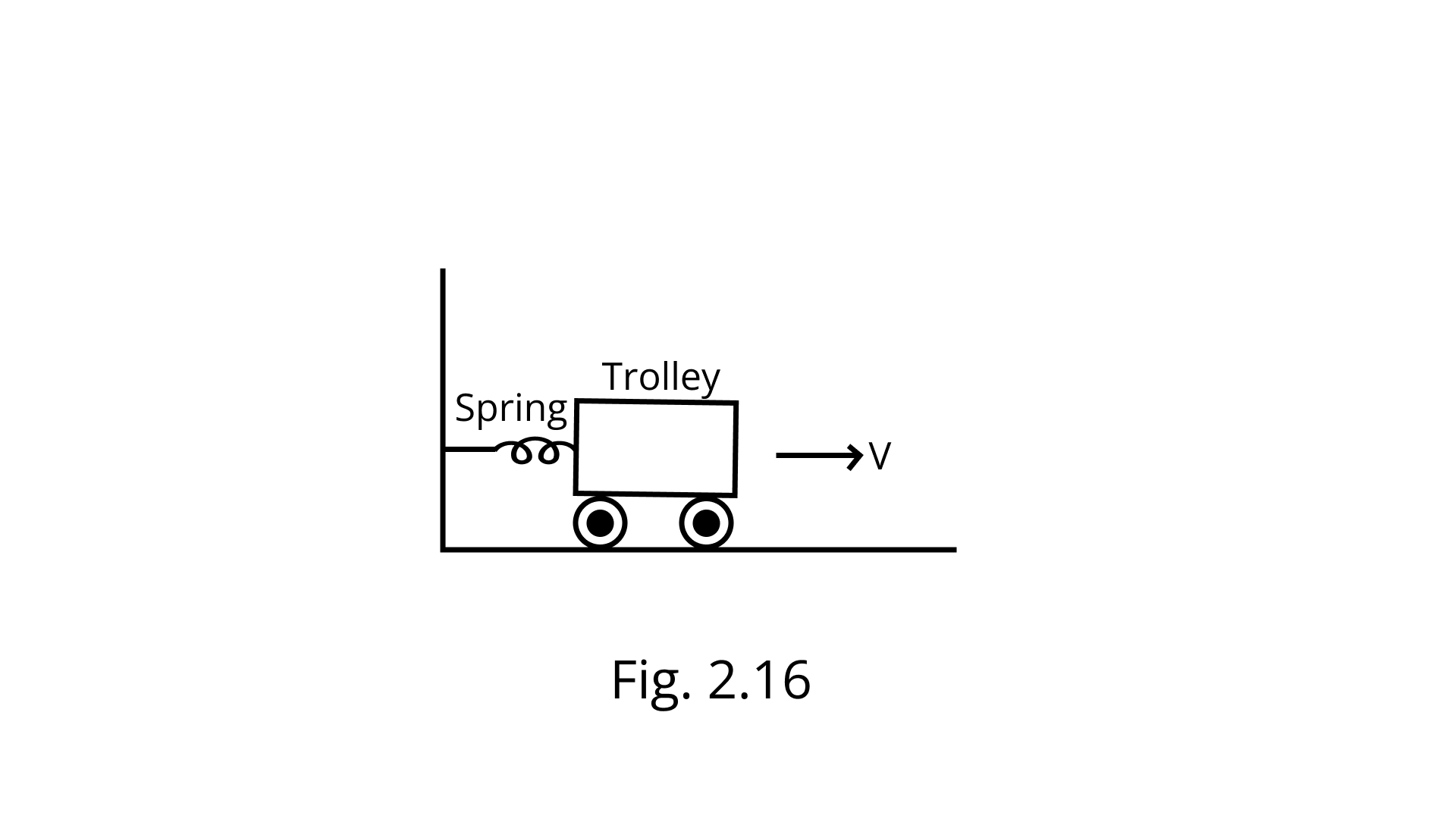
Ans: Given,

Mass of trolley M = 0.5 kg

Velocity = 2m/s

When the compressed spring is released, its potential energy is converted into kinetic energy completely.

Potential energy of compressed spring = kinetic energy of moving trolley

Kinetic energy of trolley = 

$=\dfrac{1}{2} \times \text { mass } \times(\text { velocity })^{2}$

$=\dfrac{1}{2} \times 0.5 \times(2)^{2}$

$=\dfrac{1}{2} \times 0.5 \times 2 \times 2$

$=1 \mathrm{~J}$

Therefore, potential energy of compressed spring = 1.0J

EXERCISE - 2(C)

1. State the Principle of conservation of energy.

Ans: The energy can neither be created nor destroyed; it can only be converted from one form to another form.

2. What do you understand by the conservation of mechanical energy? State the condition under which the mechanical energy is conserved.

Ans: The law of conservation of mechanical energy states that, whenever there is an interchange between the potential energy and kinetic energy, the total mechanical energy remains constant i.e. K + U = constant when there are no frictional forces. 

Mechanical energy is conserved only if there are no frictional forces i.e. between body and air

Thus, conservation of mechanical energy is valid only in vacuum, where friction due to air is absent.

3. Name two examples in which the mechanical energy of a system remains constant.

Ans: i) motion of a pendulum

ii) Motion of a freely falling body

4. A body is thrown vertically upwards. Its velocity keeps on decreasing. What happens to its kinetic energy as its velocity becomes zero?

Ans: When a body is thrown vertically upward, the kinetic energy is changed into potential energy and its velocity becomes zero. 

5. A body falls freely under gravity from rest. Name the kind of energy it will possess

(a)At the point from where it falls.

Ans: At the point from where it falls- Potential energy

(b)While falling

Ans: While falling-potential energy and kinetic energy

(c) On reaching the ground.

Ans: On reaching the ground-Kinetic energy

6. Show that the sum of kinetic energy and potential energy (i.e., total mechanical energy) is always conserved in the case of a freely falling body under gravity (with air resistance neglected) from a height h by finding it when

(i) the body is at the top,

Ans: Let a body of mass m be falling freely under gravity from a height h above the ground (i.e., from position A). 

Let us now calculate the sum of kinetic energy K and potential energy U at various positions, say at A (at height h above the ground), at B (when it has fallen through a distance x) and at C (on the ground). 

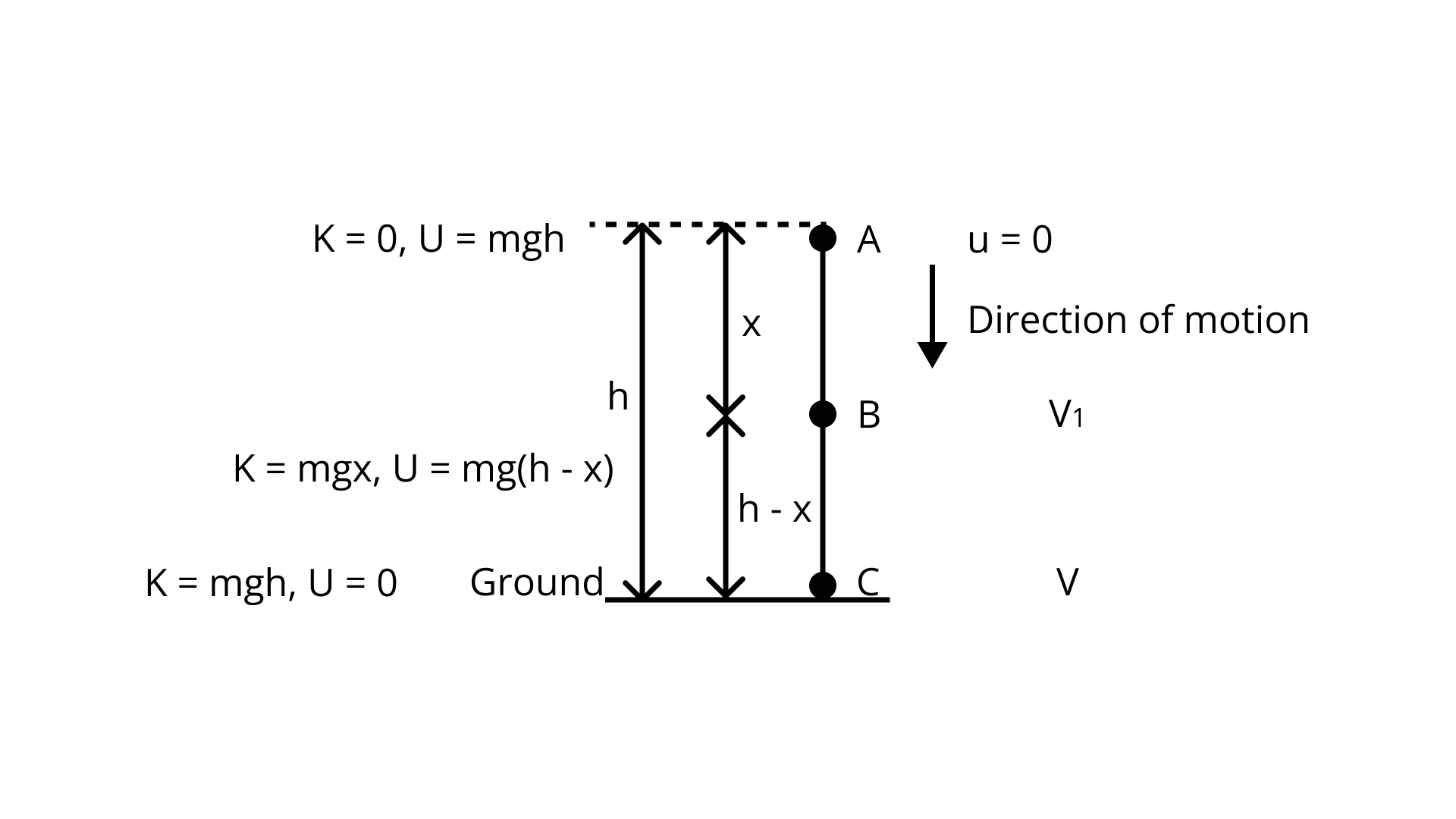
At the position A (at height h above the ground):

Initial velocity of body=0 (since body is at rest at A)

Hnce , kinetic energy K=0

Potential energy U=mgh

Hence total energy=K+U=0+mgh =mgh………….(1)

(ii) the body has fallen a distance x,

Ans: At the position B (when it has fallen at distance X):

Let v1 be the velocity acquired by the body at B after falling through a distance x. 

Then U = 0,

S = x,

a = g 

From the equation,

 $\mathrm{V}^{2}=\mathrm{u}^{2}+2 \mathrm{aS}$ 

$\mathrm{V}_{1}^{2}=0+2 \mathrm{gx}$ $=2 \mathrm{gx}$ 

Therefore, Kinetic energy 

$\mathrm{K}=\dfrac{1}{2} \mathrm{mv}_{1}{ }^{2}$ $=\dfrac{1}{2} \mathrm{~m}(2 \mathrm{gx})$ $=\mathrm{mgx}$

Kinetic energy 

$\mathrm{K}=\dfrac{1}{2} \mathrm{mv}_{1}{ }^{2}$

Now at B, height of body above the ground = h – x

Therefore Potential energy U = mg (h - x)

Therefore total energy=K+U

=mgx + mg (h-x) = mgh ………………..(2)

(iii) the body has reached the ground.

Ans: At the position C (on the ground)

Let the velocity acquired by the body on reaching the ground be v.

Then U = 0,

S = h,

a = g 

From equation;  

$\mathrm{v}^{2} =\mathrm{u}^{2}+2 \mathrm{aS}$

$\mathrm{v}^{2} =0^{2}+2 \mathrm{gh}$

$\mathrm{v}^{2} =2 \mathrm{gh}$

So, Kinetic energy $\mathrm{K}=\mathrm{K}=\dfrac{1}{2} \mathrm{mv}^{2}$ 

$=\dfrac{1}{2} \mathrm{~m}(2 \mathrm{gh})=\mathrm{mgh}$

And potential energy U = 0 (at the ground when h = 0)

Therefore total energy = K+U 

= mgh + 0 = mgh ……………….(3)

From equations (i), (ii), and (ii),

We can say that the total mechanical energy (sum of Kinetic energy and potential energy) always remains constant at each point of motion and is equal to the initial potential energy at height h.

7. A pendulum is oscillating on either side of its rest position. Explain the energy changes that take place in the oscillating pendulum. How does the mechanical energy remain constant in it? Draw necessary diagram.

Ans: 

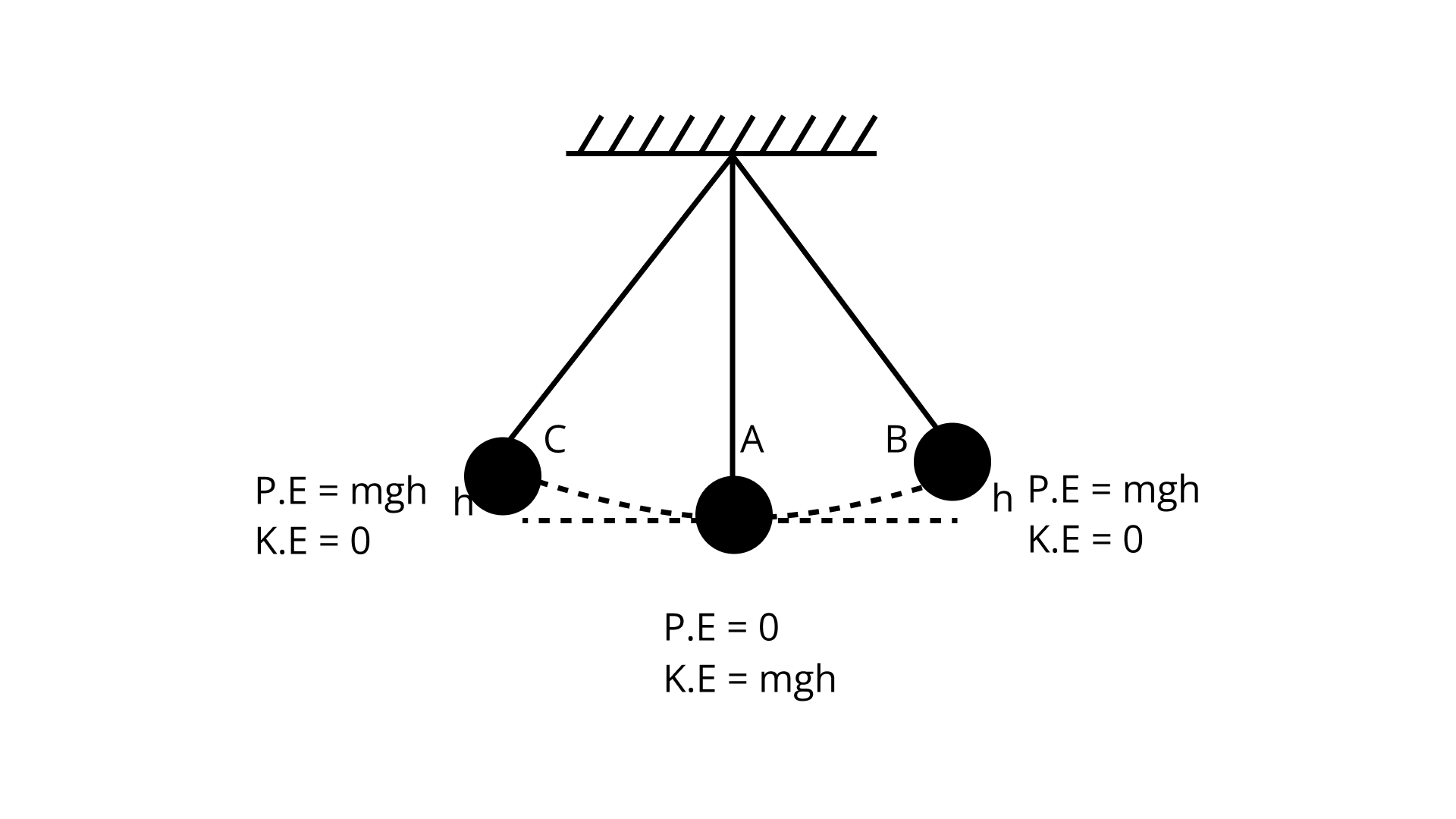
When bob swings from A to B the kinetic energy decreases and the potential energy becomes maximum at B where it rests temporarily. When the bob swings back from B to A, the potential energy again changes into kinetic energy and the process keeps repeating. Therefore, while swinging, the bob has potential energy at extreme positions B and C and kinetic energy at the resting position only. At intermediate positions i.e. between A and B or A and C, the bob has both the energies. And the sum of both the energies that is the total mechanical energy remains constant throughout the swing. 

8. A pendulum with a bob of mass m is oscillating on either side from its resting position A between the extremes B and C at a vertical height h above A. What is the kinetic energy K and potential energy U when the pendulum is at positions

(i) A,

(ii) B and

(iii) C?

Ans: (i) At position A : Pendulum has maximum kinetic energy and its potential energy is zero at resting position.

Therefore, K = mgh and U = 0

(b) At B : kinetic energy decreases and potential energy increases,

Therefore, K = 0 and U = mgh

(c) At C: kinetic energy K = 0 and potential energy U = mgh 

9. Name the type of energy possessed by the bob of a simple pendulum when it is at

(a) the extreme position,

Ans: Potential energy

(b) the mean position, and

Ans: Kinetic energy

(c) between the mean and extreme positions.

Ans: Both kinetic and potential energies.

MULTIPLE CHOICE TYPE

1. A ball of mass m is thrown vertically up with an initial velocity so as to reach a height h. The correct statement is:

(a) Potential energy of the ball at the ground is mgh.

(b) Kinetic energy to the ball at the ground is zero.

(c) Kinetic energy of the ball at the highest point is mgh.

(d) The potential energy of the ball at the highest point is mgh.

Ans: (d) The potential energy of the ball at the highest point is mgh.

2. A pendulum is oscillating on either side of its rest position. The correct statement is :

(a) It has only the kinetic energy at its each position.

(b) It has the maximum kinetic energy at its extreme position.

(c) It has the maximum potential energy at its mean position.

(d) The sum of its kinetic and potential energy remains constant throughout the motion.

Ans: (d) The sum of its kinetic and potential energy remains constant throughout the motion.

NUMERICAL

1. A ball of mass 0.20 kg is thrown vertically upwards with an initial velocity of 20m/s  Calculate the maximum potential energy it gains as it goes up.

Ans: Potential energy at the maximum height = initial kinetic energy

$=\dfrac{1}{2} \mathrm{mv}^{2}$

$=\dfrac{1}{2} \times 0.20 \times 20 \times 20$

$=40 \mathrm{~J}$

The maximum potential energy gain will be 40J

2. A stone of mass 500 g is thrown vertically upwards with a velocity of 15 ms-1. Calculate:

(a) the potential energy at the greatest height,

Ans: Given,

Potential energy at the greatest height= Initial kinetic energy=mgh

$=\dfrac{1}{2} \times 0.500 \times 15 \times 15$

$=56.25 \mathrm{~J}$

(b) the kinetic energy on reaching the ground

Ans: Kinetic energy on reaching ground = potential energy at the greatest height

=56.25 J

(c) the total energy at its half waypoint.

Ans: Total energy at its halfway point =

$=\dfrac{1}{2}(\mathrm{~K}+\mathrm{U})$

$=56.25 \mathrm{~J}$

3. A metal ball of mass 2kg is allowed to fall freely from rest from a height of 5m above the ground.   

(a) Taking $g = 10ms^{-2}$, calculate:

(i) the potential energy possessed by the ball when it is initially at rest.

Ans: Given,

the Potential energy of the ball = mgh

$=2 \times 10 \times 5$

$=100 \mathrm{~J}$

(ii) the kinetic energy of the ball just before it hits the ground?

Ans: Kinetic energy of the ball just before hitting the ground = potential initial energy

$=\mathrm{mgh}$

$=2 \times 10 \times 5$

$=100 \mathrm{~J}$

(b)What happens to the mechanical energy after the ball hits the ground and comes to rest?

Ans: Mechanical energy gets converted into heat and sound energy after the ball hits the ground and comes to rest.

4. The diagram given below shows a ski jump. A skier weighing 60kgf stands at A at the top of ski jump. He moves from A and takes off for his jump at B.

(a)Calculate the change in the gravitational potential energy of the skier between A and B.

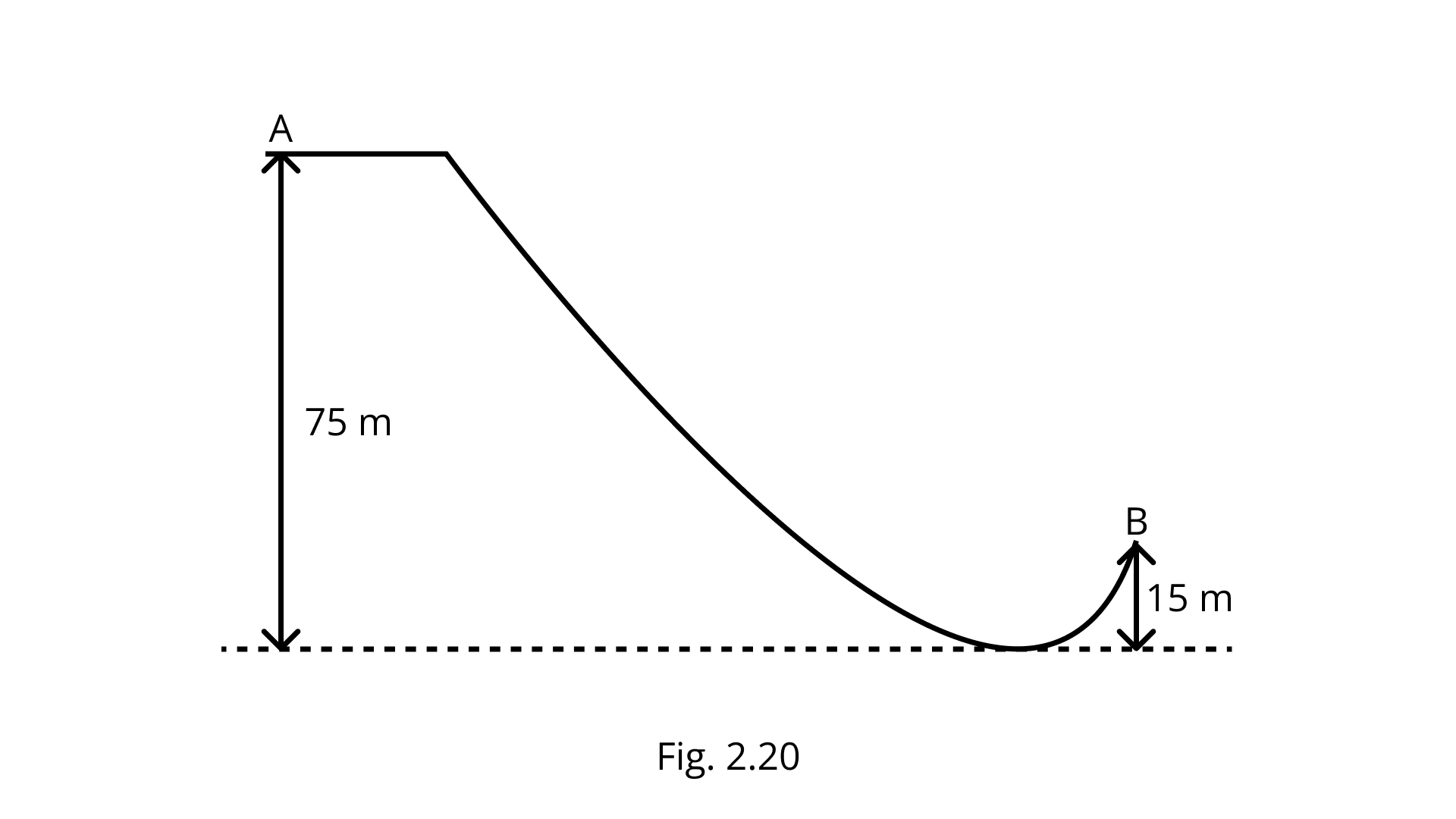
Ans: Given,

Mass of skier=60kg

The change in gravitational potential energy

Loss in potential energy =

$=\mathrm{mg}\left(\mathrm{h}_{1}-\mathrm{h}_{2}\right)$

$60 \times 10 \times(75-15)$

$=60 \times 10 \times 60$

$=3.6 \times 104 \mathrm{~J}$

(b) If $75 \%$ of the energy in part A becomes the inetic energy at B, calculate the speed at which the skier arrives at B.$(g=10 \mathrm{~ms}^{-2})$

Ans: Kinetic energy at B

$=\dfrac{75}{100} \times 3.6 \times 10^{4}$

$=27000 \mathrm{~J}$

$=2.7 \times 10^{4} \mathrm{~J}$

Kinetic energy=

$\dfrac{1}{2} \mathrm{mv}^{2}$

$27000=\dfrac{1}{2} \mathrm{mv}^{2}$

$27000=\dfrac{1}{2} \times 60 \times \mathrm{v}^{2}$

$\mathrm{v}^{2}=\dfrac{27000}{30}$

=900

$0 \mathrm{~m} / \mathrm{s}$

Therefore, the skier arrives at speed 30m/s 

5. A hydroelectric power station takes its water from a lake whose water level is 50 m above the turbine. Assuming an overall efficiency of 40%, calculate the mass of water which must flow through the turbine each second to produce power output of 1 MW. $(g = 10ms^{-2})$.

Ans: Given,

Potential energy = mgh

Efficiency = 40%

Useful work done = 40% of potential energy

$=\dfrac{40}{100}(\mathrm{mgh})$ $=200 \mathrm{~m}$ 

Power $=$ work done per second 

$1 \mathrm{MW}=200 \times$ mass of water flowing each second 

$1 \times 106 \mathrm{~W}=200 \times$ mass of water flowing per second 

Mass of water flowing each second $=\dfrac{\left(1 \times 10^{6}\right)}{200}$ 

Mass of water flowing each second $=5000 \mathrm{~kg}$

$1 \mathrm{MW}=200 \times$ mass of water flowing each second

$1 \times 106 \mathrm{~W}=200 \times$ mass of water flowing per second

Mass of water flowing each second $=\dfrac{\left(1 \times 10^{6}\right)}{200}$

Mass of water flowing each second $=5000 \mathrm{~kg}$

6. The bob of a simple pendulum is imparted a velocity of 5m s-1 when it is at its mean position. To what maximum vertical height will it rise on reaching at its extreme position if 60% of its energy is lost in overcoming the friction of air? (Take $g = 10ms^{-2}$).

Ans: Total kinetic energy 

$=\dfrac{1}{2} \mathrm{mv}^{2}=\dfrac{1}{2} \mathrm{~m} \times 5^{2}$

Energy lost $=\dfrac{1}{2} \mathrm{~m} \times 5^{2} \times \dfrac{60}{100}$

Energy available $=\dfrac{1}{2} \mathrm{~m} \times 5^{2} \times \dfrac{40}{100}$

As per energy conservation,

$\dfrac{1}{2} \mathrm{~m} \times 5^{2} \dfrac{40}{100}=\mathrm{mgh}$

$5=10 \times h$

$\mathrm{~h}=0.5 \mathrm{~m}$

ICSE SELINA for Work, Energy, and Power Solutions for Class 10 Physics ICSE Board covers all the important topics. The solutions consist of a detailed explanation of every question that is asked. This will help the students to clear any doubt they have and improve their application skills while preparing for board exams. The concepts will be understood better by the students with the help of a step-by-step guide of the chapter Work, Energy, And Power for class 10 physics ICSE Board. Class 10 is no easy nut to crack and the concepts are a bit complex and difficult to understand but with the help of the solutions from Selina Publishers, the students can grasp the concept better.

The solutions at Vedantu are such that the students can prepare for written exams. The textbook of Selina can be of more help for self-study and acts as perfect self-help guidance for the students studying physics.

The concepts that are covered in Selina Physics Class 10 in the chapter work, energy, and power are Introduction of work, measurement of work, the concept of power, concept of energy, types of mechanical energy like potential energy and its types, gravitational potential energy, mechanical energy and it's different forms, work done by the gravitational force (W = mgh), different forms of energy, the definition of work, the transformation of energy, conversion of potential energy into kinetic energy, theoretical verification of K+U = Constant for a freely falling body, types of mechanical energy - Kinetic energy, types of kinetic energy, application of the principle of conservation of energy to a simple pendulum, principle of conservation of energy.

Using the Selina book, chapter 2 Work, energy, and power can be easily studied by the students. The concepts will get very clear and they can prepare themselves for their board exams. All the questions in Selina are important and have a high probability of being asked in the final exams. To score a student should be thorough with everything in the chapter.