




A Brief Description about Ideal Gas
A hypothetical gas known as an ideal gas is made up of several randomly moving point particles with no interparticle interactions. Since it follows the ideal gas law, the ideal gas concept is of great significance. Many real gases behave qualitatively like an ideal gas under various temperature and pressure conditions. Many gases, including nitrogen, oxygen, hydrogen, noble gases, some heavier gases like carbon dioxide and mixtures like air, can be classified as ideal gases under some specific temperatures and pressure conditions.
Typically, at higher temperatures and lower pressures, a gas behaves more like an ideal gas. The ideal gas law is commonly expressed in an empirical form:
PV=nRT
where P, V and T stand for pressure, volume and temperature respectively, n denotes the number of moles of gas and R denotes the ideal gas constant.
What is the Compressibility Factor?
The compressibility factor Z, also known as the compression factor or the gas deviation factor, explains how a real gas differs from the behaviour of an ideal gas. The molar volume ratio of a real gas to an ideal gas at the same temperature and pressure is the simplest way to describe it. It is a practical thermodynamic property for modifying the ideal gas law, in order to take real gas behaviour into account. As Z is a ratio, it is dimensionless. Hence, there is no SI unit of compressibility factor. Deviations from ideal behaviour typically become more significant.
Compressibility factor formula is given by:
$\mathrm{Z}=\dfrac{\mathrm{V}_{\text {real }}}{\mathrm{V}_{\text {ideal }}}=\dfrac{\mathrm{PV}_{\text {real }}}{\mathrm{nRT}}$
The compressibility factor of an ideal gas is Z=1. In general, the value of Z increases with pressure and decreases with temperature. As attractive forces dominate the value of z<1 while repulsive forces dominate, the value of z becomes z>1.
Values for compressibility factors are typically calculated using equations of state (EOS), like the virial equation. Generalised compressibility charts that plot Z as a function of pressure at constant temperature can be used to determine the compressibility factor for particular gases.
Plot of Compressibility Factor Z vs Pressure
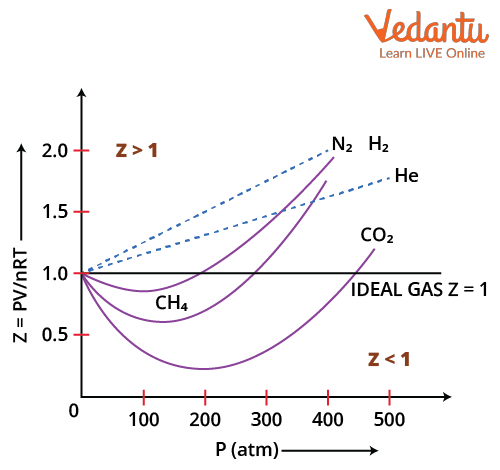
Graph of z vs p
In the plot of z vs p, the straight in centre is for ideal gas where z=1. If z>1, repulsion dominates in gases for gases like N2, He and H2. If z<1, then attraction dominates for all other gases. These gases at some temperature or pressure close to z=1 behave like ideal gases and show minimal interaction between molecules. For example, CO2 is a large molecule with a high degree of polarity. As a result, there is a steep drop in the curve at low pressure and there is a large intermolecular attraction there. N2 has the steepest increase in Z, however, straight from low pressure, since it is an inert molecule with the least intermolecular interaction.
The Van Der Waals Equation
In 1873, the Van Der Waals equation was developed. The forces of attraction between gas molecules is given by a. b is the volume occupied by 1 mole of gaseous particles. Although a and b are known as "the Van Der Waals constants“, they are actually variables that differ from gas to gas while being independent of P, V and T.
The compressibility factor z for a real gas is given by the equation:
$\mathrm{Z}=\dfrac{\mathrm{PV}_{\mathrm{m}}}{\mathrm{RT}}=\dfrac{\mathrm{V}_{\mathrm{m}}}{\mathrm{RT}}\left(\dfrac{\mathrm{RT}}{\mathrm{V}_{\mathrm{m}}-\mathrm{b}}-\dfrac{\mathrm{a}}{\mathrm{V}_{\mathrm{m}}^{2}}\right)$
Virial Equation
We may combine the ideal gas law and the compressibility factors of real gases to create an equation that describes the isotherms of a real gas, because the perfect gas law is an imperfect representation of a real gas. The Virial Equation of State is used to describe real gases.
The second, third and fourth virial coefficients, respectively, are denoted by the parameters B, C and D. The coefficients are not constants, because they change depending on the type of gas being considered as well as its temperature. The majority of the correction for a gas's less-than-ideal behaviour is provided by the second virial coefficient. Hence, sometimes this equation is represented till the second coefficient and virial equation is shortened.
If z is the compressibility factor virial equation is given as:
$\dfrac{P V_{\mathrm{m}}}{R T}=Z=1+\dfrac{B}{V_{\mathrm{m}}}+\dfrac{C}{V_{\mathrm{m}}^{2}}+\dfrac{D}{V_{\mathrm{m}}^{3}}+\ldots$
Conclusion
A hypothetical gas known as an ideal gas is made up of several randomly moving point particles with no interparticle interactions. Since it follows the ideal gas law, the ideal gas concept is of great significance. Many real gases behave qualitatively like an ideal gas under various temperature and pressure conditions. The compressibility factor, also known as the compression factor or the gas deviation factor, explains how a real gas differs from the behaviour of an ideal gas. The molar volume ratio of a real gas to an ideal gas at the same temperature and pressure is the simplest way to describe it. Compressibility is represented by Z and is dimensionless.
FAQs on Compressibility Factor Z | Plot of Compressibility Factor Z Vs Pressure for JEE
1. Define characteristics of ideal gas?
Ideal gases are composed of molecules and atoms, two types of tiny particles. The volume of the gas's particles is extremely small. The particles of ideal gases have negligible volume. The forces of attraction between the gas's particles are seen as being negligible. Individual gas molecule collisions are described as being perfectly elastic. The motion of the gas particles will always be random. Ideal gases undergo brownian motion. There are a lot of identical molecules in an ideal gas. The motion of the molecules follows Newton's laws of motion.
2. What is real gas?
A real gas is one that disobeys the gas laws under any conventional pressure and temperature conditions. As the size of gaseous particles increases and volume of gas increases, it deviates from its ideal behaviour. Real gases have mass, velocity and volume. When cooled to their boiling point, real gas liquifies. When compared to the total volume of gas, the space that the gas occupies is not little. Therefore, a real gas can be described as a non-ideal gas having molecules that occupy a specific amount of space and have the ability to interact with one another.
























