Graph of a Quadratic Polynomial for JEE

A polynomial is any expression in mathematics that has one or more algebraic terms along with numerical values. A polynomial function can comprise completely of variable terms as well. A few examples of polynomial functions will be the quadratic and cubic functions. Even a linear function in the form of y = mx+ x will be considered a linear polynomial whose graph is a straight line.

A quadratic polynomial is a mathematical expression where the highest power or degree of the variables is 2. Quadratic polynomials trace a different curve when plotted and graphed on the sheet. The graph of a quadratic polynomial is a parabola. Depending on the values of the coefficients in the expression and the discriminant, we get either a parabola opening upwards or downwards when x is the independent variable. Again, if the quadratic expression is in terms of y where the x is the dependable variable, the parabola will open either towards the right or towards the left. 

Graph of Quadratic Polynomial

As mentioned before, the quadratic polynomial graph is a parabola. If the polynomial function has x as the independent variable, then the roots of the quadratic expression give us the points where the graph cuts the x-axis. Similarly, if the quadratic function is in terms of y as the independent variable, then the roots of the function will give us the point of intersection of the y-axis and the parabolic curve.

To draw the graph of a quadratic polynomial we must follow the steps given below:

• Let the given polynomial by $f(x)=a x^{2}+b x+c$. This is a quadratic function with x as the independent variable and a, b, and c are the arbitrary constants of the expression. 

• Next, we write the given quadratic function as a y that is $y=f(x)=a x^{2}+b x+c$

• The third step is to calculate the zeroes of the quadratic polynomial by equation y to 0. This goes as follows: $y=f(x)=a x^{2}+b x+c=0$

• To get the points where the parabolic curve meets the y axis, we replace x with 0.

• Lastly we calculate the value of the discriminant of the quadratic function that is given by $D=b^2-4ac$. 

When D > 0, the graph intersects the x-axis at two distinct points which are given by the roots of the functions

When D = 0, the parabolic graph touches the x-axis at one single point.

When D < 0, the graph does not touch the axis and lies far away. 

• The last step is to find the turning point of the curve which has the coordinates    $\left(-\dfrac{b}{2a},~-\dfrac{D}{4a}\right)$. 

For drawing on the graph, we must plot the x values and their corresponding y values. By plotting these points on the graph we can easily draw the graph of a quadratic polynomial. If the value of the coefficient of the squared x term i.e. a > 0 then the parabola opens upwards. If a <0 the parabola will open downwards

To understand this further, we take the following quadratic polynomial example:

Example: Let the given quadratic polynomial function be $f(x)=x^{2}+2 x-3$. Draw the graph of the given quadratic function.

Solution:

To solve this example we will follow the exact steps mentioned above. 

Let $f(x)=x^{2}+2 x-3$. Comparing the given polynomial with the standard form we get that, a = 1, b = 2 and  c= -3.

Since a > 0, the parabola will open upwards. 

Next, we calculate the discriminant, $D=b^2-4ac=2^2-4(1)(-3)=16$

As we can see D > 0. Therefore the parabola will intersect the x-axis at two distinct points which will give us the roots of the quadratic polynomial.

Equation y and 0, we get $x^{2}+2 x-3=0$.

Simplifying we get, $x^{2}+2 x-3=0 \Rightarrow x(x+3)-1(x+3)=0 \Rightarrow(x-1)(x+3)=0$

From the above expression we can find out the roots of the given quadratic function to be 1 and -3. i.e. either x = 1 or x = (-3)

Thus the graph intersects the x-axis at points ( 1 ,0 ) and ( -3,0 ). 

The vertex of the parabola is given by $\left(-\dfrac{b}{2 a},-\dfrac{D}{4 a}\right)=\left(-\dfrac{2}{2},-\dfrac{16}{4}\right)=(-1,-4)$

The required polynomial chart to draw the graph will be given as follows:

Required Table

Image 1: Table showing the x and y values for $y=x^{2}+2 x-3$

Therefore the required graph that we obtain from plotting all the points is as follows: 

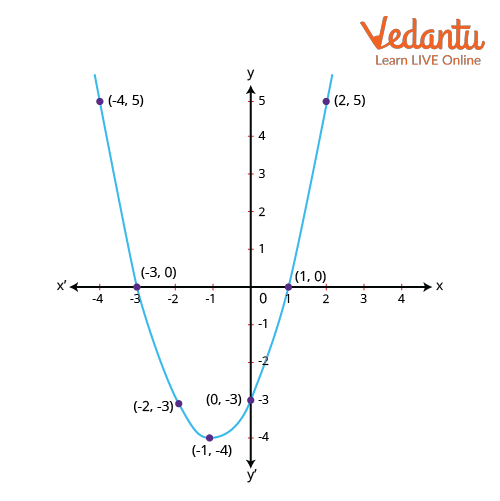
Parabolic Graph of $y=x^{2}+2 x-3$

Relationship Between Zeros of a Quadratic Polynomial and its Roots

Let us consider the quadratic polynomial, $y=f(x)=ax^2+bx+c$. Let α and β be the zeros of the polynomial. As per the factor theorem, we get $(x-\alpha)$ and $(x-\beta)$ are the factors of the given quadratic polynomial.

Therefore, $f ( x ) = k ( x – \alpha)  (x – \beta)$ 

$\Rightarrow a x^{2}+b x+c=k\left\{x^{2}-(\alpha+\beta) x+\alpha \beta\right\}=k x^{2}-(k \alpha+\beta) x+k \alpha \beta$

Thus comparing the coefficients  of the terms of the quadratic function we get, $\alpha + \beta = -\dfrac{b}{a}$ and $\alpha\beta = \dfrac{c}{a}$ 

Therefore the sum of the zeroes is given by $\left( -\dfrac{b}{a}\right)$ and the product of the zeroes is given by $\left(\dfrac{c}{a}\right)$. 

Conclusion

A quadratic polynomial is any mathematical expression constituting numerical coefficients and variables. The highest degree of power of the variable in this context is 2. The graph of a quadratic polynomial is a parabola. If the coefficient of the x2 term is positive then the parabola will open upwards. If the coefficient of the x² term is less than 0, then the parabola opens upwards. The value of the discriminant gives us the position of the curve on the graph. When D= 0 the graph intersects the x-axis and at D > 0 and D < 0, the curve touches the axis at a single point and lies above the axis, respectively. The relations between the roots of a quadratic polynomial and its roots are given as follows: $\alpha + \beta = -\dfrac{b}{a}$ and $\alpha\beta = \dfrac{c}{a}$