




What is RC Circuit?
The RC circuit is the contraction of the Resistor-Capacitor circuit. This circuit consists of components which are majorly resistors and capacitors. Along with these components, the RC circuit comprises a voltage source or current source which are responsible for the working of the circuit. The RC circuit can contain any number of resistors and capacitors. This number is independent and changes accordingly to the needs of electronics. An RC circuit comprises only one resistor and one capacitor and is called the first-order RC circuit as it is the simplest of all. Resistance is measured in ohms and the capacitance is measured in Farads.
Since there is a resistance in the circuit, there will be a power dissipation in the circuit. These circuits are used in various applications mainly in filters where they are used to block some range of frequency signals and allow only a certain range to pass through them. A few examples of those filters are low pass filters, high pass filters and band pass filters. Generally, a resistor is connected to a capacitor prominently to control the rate of charging and the discharging of the capacitor circuit. This happens as the capacitor stores energy when the current starts passing through it. Let us understand how the capacitor in an RC circuit gets charged and what are the applications of the RC circuit in detail.
Charging Characteristics of an RC Circuit
To know how the capacitor gets charged, let us consider a capacitor which was completely discharged initially and is connected in series with the resistor whose resistance is R. A voltage source is connected to the end of the resistor and the switch is kept open. Initially, when the switch is kept open, there is no charge generated in the circuit. When we close the switch, the current starts flowing from the circuit along with the capacitor and the resistor.
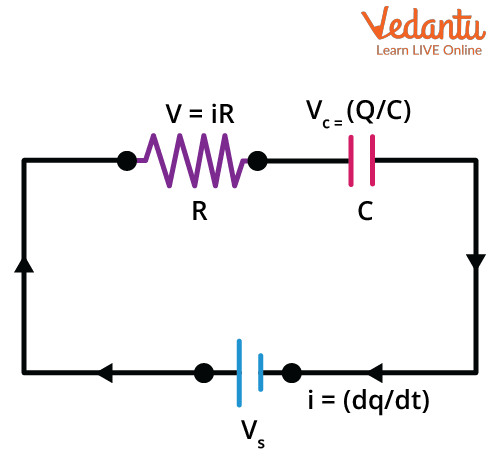
RC Circuit Diagram
As the capacitor takes too much time to get charged, in the initial moments when the switch is closed there is no potential difference across the capacitor. As the capacitor is getting charged at this moment, that is why we call the current flowing through the circuit the charging current. After some time, the capacitor gets charged up and a charge Q = CV develops on the plates of the capacitor.
Here, C is the capacitance of the capacitor and V is the voltage present on the plates of the capacitor. Until the capacitor gets completely charged, it doesn't let out any energy present within the plates of the capacitor. It gets charged until the voltage across the two plates is equal to the voltage we are supplying to the circuit.
Kirchhoff's Voltage Law in RC Circuit
When we apply Kirchhoff's voltage law in the RC circuit, which is shown above, we obtain the charging current
$l=\dfrac{V_s}{R}$
Where Vs is the supply voltage to the circuit and R is the resistance of the resistor that is present in the circuit.
When the capacitor starts charging and when the potential difference is created between the plates of the capacitor, then we apply Kirchhoff’s voltage law as follows,
Vs - Vc - iR = 0
Where Vc is the voltage difference across the capacitor.
iR = Vs - Vc
And we know $V_c=\dfrac{Q}{C}$ and let’s substitute the value of $V_c$ in the above equation.
Therefore, $\mathrm{i}=\dfrac{V_{s} C-Q}{R C}$
And we know that current is the rate of flow of charge per time, i.e., $i=\dfrac{dq}{dt}$
$\begin{align} &\dfrac{d q}{d t}=\dfrac{V_{s} C-Q}{R C} \\ &\dfrac{d q}{V_{s} C-Q}=\dfrac{d t}{R C} \end{align}$
Therefore, integrating on both sides from q = 0 to q = q and t = 0 to t = t, we get
$\begin{align} &\int_{0}^{Q} \dfrac{d q}{V_{s} C-Q}=\int_{o}^{t} \dfrac{1}{R C} d t\\ &\left[\dfrac{\ln \left(V_{s} C-Q\right)}{-1}\right]_{0}^{Q}=\dfrac{1}{R C}[t]_{0}^{t}\\ &-\left[\ln \left(V_{s} C\right)-\ln \left(V_{s} C-Q\right)\right]=\dfrac{-t}{R C}\\ &\left[\ln \left(V_{s} C-Q\right)-\ln \left(V_{s} C\right)\right]=\dfrac{-t}{R C}\end{align}$
$\begin{align} &\ln \dfrac{V_{s} C-Q}{V_{s} C}=\dfrac{-t}{R C}\\ &\dfrac{V_{s} C-Q}{V_{s} C}=e^{\dfrac{-t}{R C}}\\ &V_{s} C-Q=V_{s} C\left(e^{\dfrac{-t}{R C}}\right)\\ &Q=V_{s} C\left(1-e^{\dfrac{-t}{R C}}\right) \end{align}$
This is the RC circuit formula for charge obtained after the RC circuit analysis for the charge that flows in the circuit at some point in time t.
If we want to find the current flowing in the circuit at time t, then we need to differentiate the equation of charge obtained above.
$l=\dfrac{dq}{dt}$
$\begin{align}&\dfrac{d}{d t}\left(V_{s} C\left(1-e^{\left.\dfrac{-t}{R C}\right)}\right)=V_{s} C \dfrac{d}{d t}\left(1-e^{\dfrac{-t}{R C}}\right)\right. \\ &\dfrac{V_{s} C}{R C} e^{\dfrac{-t}{R C}}=\dfrac{V_{s}}{R} e^{\dfrac{-t}{R C}} \end{align}$
This is the RC circuit formula of current flowing at time t. Here RC is known as the time constant of the circuit which is defined as the time required for the capacitor to get charged until it attains 63.2% of the maximum voltage applied to the circuit. It is denoted by tau, $\tau=\mathrm{RC}$.
The reactance of the capacitor is given by, $X_{c}=\dfrac{1}{\omega C}$ which $\omega$ is the angular frequency of the current or voltage in the circuit.
$\omega=\dfrac{1}{2 \pi f}$
Where f is the frequency.
The total impedance of the RC circuit is given by $Z=\sqrt{R^{2}+X_{C}^{2}}$.
Numerical Examples of RC Circuit
Example 1: Find the impedance of the circuit and the time constant if angular frequency, $\omega$ = 200 rad-1sec and a resistor of R = 25$\Omega$ is connected in series with a capacitor of capacitance, C = 5 $\times$ 10-6F.
Solution:
We know that the time constant is given by the formula
$\tau=\mathrm{RC}$
= (25)(5 $\times$ 10-6) = 125 $\times$ 10-6 seconds
Therefore, Time constant = 125 $\times$ 10-6 seconds
The impedance of the circuit, $Z=\sqrt{R^{2}+X_{C}^{2}}$
Where $\mathrm{X}_{c}=\dfrac{1}{\omega C}=\dfrac{1}{200 \times 5 \times 10^{-6}}=10^{3} \Omega$
Therefore, $Z=\sqrt{(25)^{2}+\left(10^{3}\right)^{2}}=317.21 \Omega$
Therefore, the impedance of the circuit = 317.21$\Omega$
Example 2: Find the charge flowing in the capacitor at t = 5 sec when the supplied voltage to the circuit is Vs= 4.2 V and Resistance R = 25$\Omega$.
Solution:
The charge flowing through the capacitor is given by the formula
$q=V_{s} C\left(1-e^{\dfrac{-t}{R C}}\right)$
Here, Given
Vs = 4.2V ,C = 5 $\times$ 10-6 F, R = 25$\Omega$ and t = 5sec
Substituting the values in the above formula, we obtain
$q=4.2\left(5 \times 10^{-6}\right)\left(1-e^{\dfrac{-5}{125 \times 10^{-6}}}\right) \cong 21 \times 10^{-6}$
The charge flowing in the capacitor at t=5 sec is 21 $\times$ 10-6C.
Applications of RC Circuit
An RC circuit is frequently used in electronics and in our day-to-day life, some of the applications of the RC circuit are mentioned below:
These circuits are used in traffic lights and also in a wide variety of equipment that is used to control and receive audio signals.
In some cases, where there is a need for filtration of signals with high or low frequencies, these circuits make huge use of it.
These are also used as an integrator or differentiator circuits and camera flashes. These are the RC circuit applications that are used prominently in a human’s life.
Conclusion
In this article, we have learnt what RC circuits are and did an RC circuit analysis by providing all the required formulae along with the derivation of charge and current at time t. RC circuits are very useful in electronics and learning them is very essential. To learn from more of such Physics JEE articles or other JEE subjects, visit our website today.
FAQs on RC Circuit - JEE Important Topic
1. What is the impedance of an RC circuit?
The impedance of the RC circuit could be defined as the opposition to the flow of AC in the circuit and it is represented by the letter Z. We can describe this as the resistance for the whole circuit which consists of various components like resistors, capacitors and inductors. We can obtain the value of Z by the given formula, $Z=\dfrac{E}{I}$ where E is the terminal voltage of the circuit and I is the total current flowing through the circuit.
2. What is the weightage of this topic in JEE?
The topic RC circuit, capacitance and AC currents are related and many questions will be asked from these concepts, like finding the capacitance, finding the charge on the capacitor, finding the impedance of the circuit, finding the angular frequency, finding the current flowing at some given time through the capacitor, finding the reactance of the capacitor, deriving the expression for the voltage across the capacitor and so forth. A maximum of 3 to 4 questions can be asked on these topics and there is a weightage of 10% in JEE.
3. Why is the RC circuit used in low pass filters?
A low pass filter is a filter that allows only frequency signals which are below a cut-off frequency and blocks any signal with a frequency higher than the cut-off frequency. In an RC circuit, if the applied voltage is of low frequency then the reactance of C is very high than that of the resistor so it can be considered an open circuit where the current doesn’t flow. Therefore, only at low frequencies of voltage, the current flow and the output is obtained. This is why it is used as the low pass filter.












