




What is the Photoelectric Effect?
In 1905, Albert Einstein gave his Nobel prize-winning paper on the discrete quantisation of energy in electromagnetic radiation. The core premise of this work was based on the data obtained from the photoelectric effect experiments done by Aleksandr Stoletov. To explain the photoelectric effect, they found a correlation between the intensity of light and the number of electrons emitted. According to the photoelectric effect, when an electromagnetic wave of a specific frequency (energy) is bombarded on a metal, the electrons inside the metal are ejected and become known as photoelectrons through a process known as photoemission.
Work Function
The work function draws a barrier between emission and absorption. If the incident electromagnetic wave does not have energy equal to the work function of the material, electrons will not be ejected from the atoms of the metal. Quantised wave packets can activate emission if they have energies greater than the work function of the metal.
In solid-state physics, the work function is the minimum thermodynamic work needed to remove an electron from a solid to a point in the vacuum immediately outside the solid surface. Work function is a property of a material, which is defined as the minimum quantity of energy required to remove an electron to infinity from the surface of a given solid.
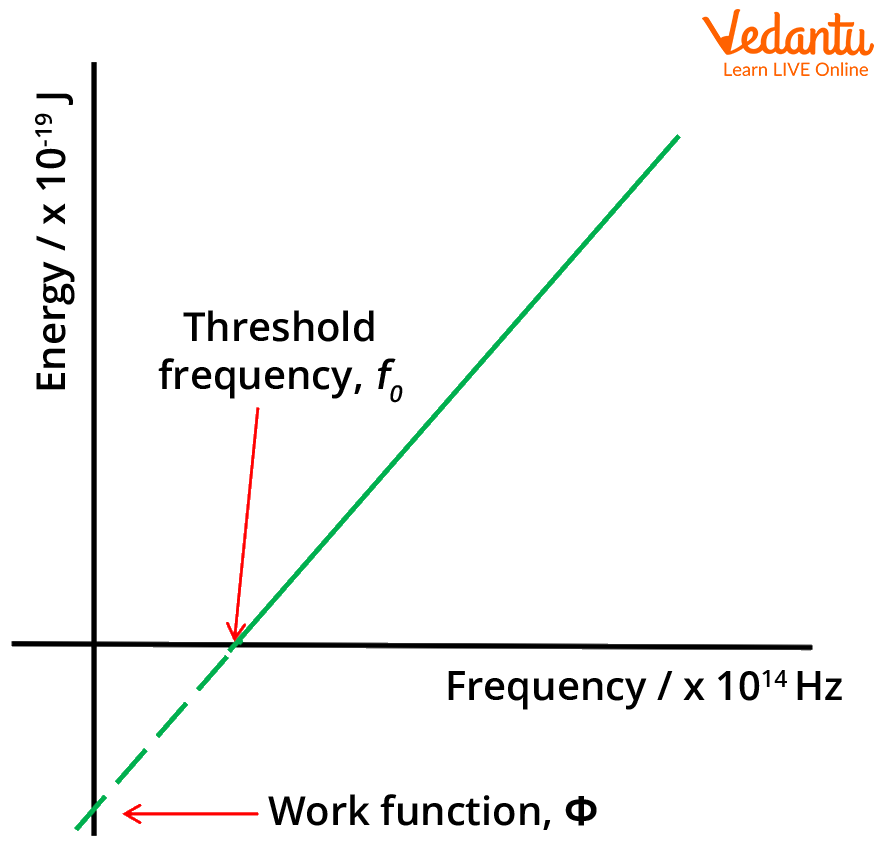
Energy versus frequency graph
What is Stopping Potential?
Stopping potential, or cut-off potential, is defined as the required potential for stopping the removal of an electron from a metal surface when the incident light energy is greater than the work potential of the metal on which the incident light is focused. The work function and stopping potential tend to mean the same thing in terms of the photoelectric effect.
Derivation of Maximum Kinetic Energy:
The concept of the derivation of Maximum Kinetic Energy using Einstein's explanation of the photoelectric effect in simpler terms:
Einstein figured out that light energy comes in small packets called photons. Each photon has a certain amount of energy, and when it hits a metal surface, an electron can absorb that energy. If the absorbed energy is enough to overcome the "stickiness" of the metal (called work function), the electron gains extra energy and gets kicked out from the metal.
Energy of the photon (hf) ≥ Work function (Φ) + Maximum Kinetic Energy (KEmax)
By rearranging the formula, we find the maximum kinetic energy:
KEmax = hf - Φ
Two important things to note:
Threshold Frequency: If the frequency of light is below a certain value (ν₀ = Φ/h), the absorbed energy is not enough to free the electron, so nothing happens.
Frequency Dependence: If the light frequency is above the threshold, the more the frequency increases, the more extra energy the electron gets. This is different from what classical physics would predict, where brighter light should just give more energy. In the photoelectric effect, it's the frequency that matters, not the brightness.
Derivation of Photoelectric Effect and Stopping Potential Formula
To derive Einstein’s photoelectric equation formula for stopping potential, we need to identify the required variables and parameters first. As we know
$ c=\nu \lambda$
where c is the speed of light, 𝛎 is the frequency of the electromagnetic wave, and $\lambda$ is the measure of the wavelength of the electromagnetic wave.
$\nu=\dfrac{c}{\lambda}$
$\Rightarrow E=h \nu$……..(1)
Here, E is the energy of the electromagnetic wave.
Therefore, we can use the known parameters to calculate the kinetic energy of the photoelectrons.
$K E_{\max }=\dfrac{h c}{\lambda}-\phi $……..(2)
The stopping potential $\phi$ can be given as
$\phi=\dfrac{h c}{\lambda}-K E_{\max }$
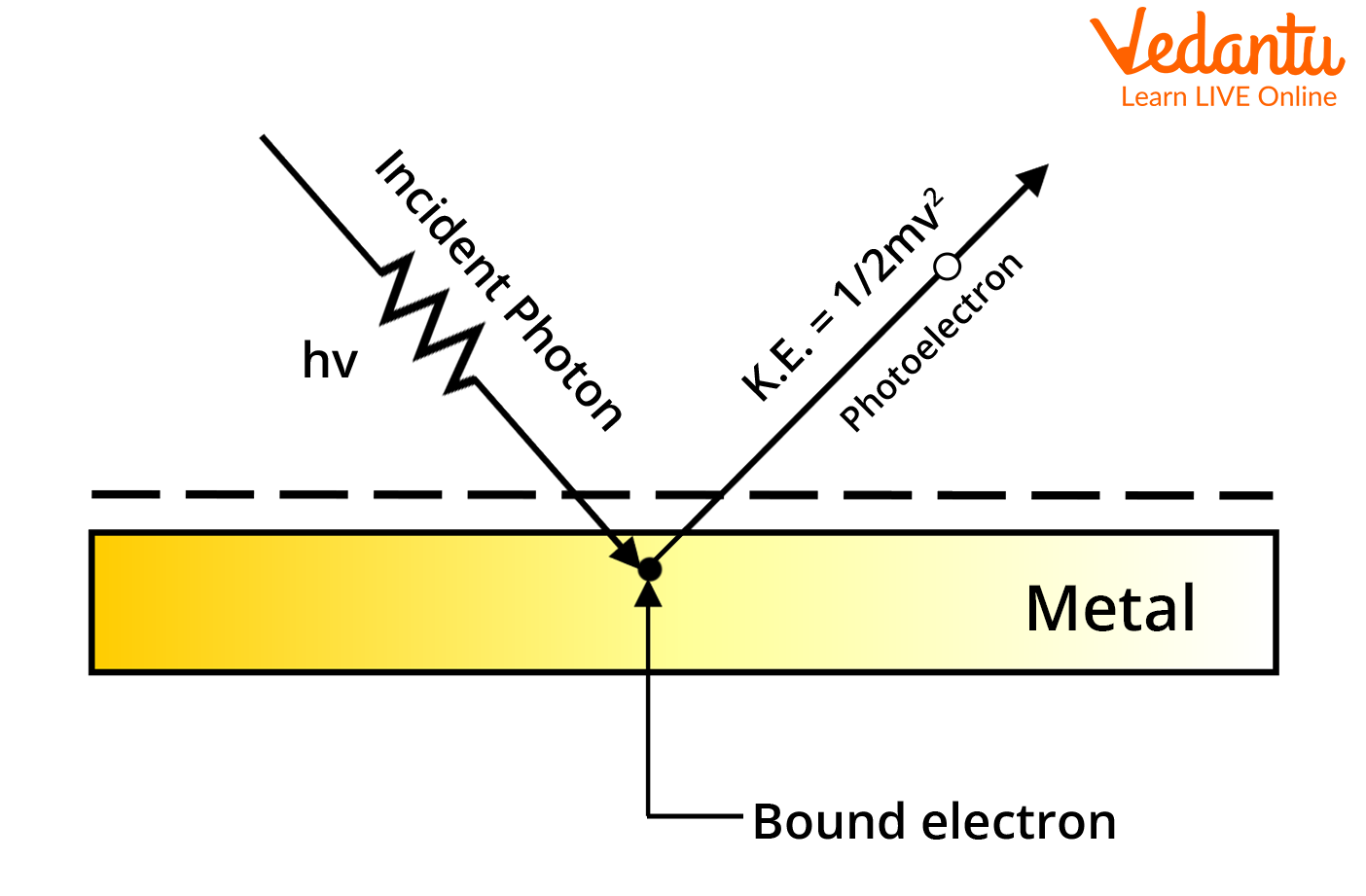
Process of Photoemission
Now the kinetic energy can be written as
$K E_{\max }=\dfrac{1}{2} m v_{\max}^{2} $
Also, the energy of a photo equal to the work function can be written as
$\begin{align} &\phi=\dfrac{h c}{\lambda_{0}} \\ &\phi=h \nu_{0} \end{align}$
So, the equation for the stopping potential can be written as
$\begin{align} &h \nu_{0}=\dfrac{h c}{\lambda}-\dfrac{1}{2} m v_{\max }^{2} \\ &h \nu_{0}=h \nu-\dfrac{1}{2} m v_{\max }^{2} \end{align}$
We can also write
$\begin{align} &\dfrac{1}{2} m v_{\max }^{2}=h \nu-h v_{0} \\ &\dfrac{1}{2} m v_{\max }^{2}=h\left(\nu-\nu_{0}\right) \end{align}$
The photoelectric effect can be derived from the concept of Planck's quantum theory. Photons travel in discrete energy packets, where the energy of one photon is given by the equation (1). $\nu$ is the frequency of the incident photon. ‘h’ is the Planck’s constant whose value is $h=6.6260715 \times 10^{-34} \mathrm{~J} . \mathrm{s}$ the units are Joule-Second.
To summarise the variables used in the derivation of the photoelectric equation, we have
h, which is the Planck constant.
$\nu$, which is the frequency of the radiation in question.
$\lambda$, which is the wavelength of the radiation.
c, which is the speed of light in a vacuum.
E, which is the energy of the photon.
There is a single interaction that sums up the photoelectric effect, the incidence of a photon on the metal surface, and its interaction with the electron bound to the surface of the metal within the interatomic interactions.
Derivation of Key Equations Governing the Photoelectric Effect
Let's delve into the derivation of the key equations governing the photoelectric effect. Starting with the conservation of energy, we equate the energy of the incident photon to the sum of the work function and the kinetic energy of the ejected electron:
hf=Φ+1/2 mv2
Simplifying and using the de Broglie wavelength (λ= h/mv), we arrive at the expression for kinetic energy:
KEmax=hf−Φ
This derivation provides a deeper insight into the relationship between incident light, work function, and the resulting kinetic energy of ejected electrons.
Dependence of Photoelectric Effect on Different Parameters
For a light of any given frequency, the photoelectric current is directly proportional to the intensity of light.
If the frequency of light is no greater than the threshold frequency, the emission of photoelectrons will be stopped, no matter how intense the light rays are.
The maximum kinetic energy of the photoelectrons emitted is independent of the intensity of light. As the frequency of light increases over the threshold frequency, the kinetic energy of the electrons also increases.
The process of photoemission is instantaneous, as it depends on the quanta of energy the radiation brings with it.
Applications of the Photoelectric Effect:
Solar Panels: They use special metals to turn light into electricity from various wavelengths.
Motion and Position Sensors: These sensors use a material that reacts to light (UV or IR) along with LEDs. When something blocks the light, a change in electricity is noted.
Lighting Sensors (e.g., in smartphones): Adjust screen brightness automatically based on the light intensity, thanks to the photoelectric effect generating current.
Digital Cameras: Detect and capture light by using sensors that respond to different colors.
X-Ray Photoelectron Spectroscopy (XPS): Uses X-rays to learn about surfaces, like elemental and chemical composition.
Burglar Alarms: Photoelectric cells help in detecting intruders.
Photomultipliers: Used to find very low levels of light.
Video Camera Tubes (early TV): Photoelectric effect was key in early television cameras.
Night Vision Devices: They work because of the photoelectric effect.
Chemical Analysis: Photoelectric effect aids in studying nuclear processes and analyzing materials by examining the energy of emitted electrons.
Conclusion
The phenomenon of the photoelectric effect was discovered by the works of Heinrich Hertz, Albert Einstein, Max Planck, and others during the late 19th to early 20th century. The photoelectric effect can be defined as a phenomenon during which electrons are emitted from the surface of the metal when the light of a suitable wavelength is incident on it. These electrons are termed photoelectrons.
This emission occurs due to the electrons absorbing energy from the electromagnetic radiation and using this agitation to break free from the intra-atomic forces of attraction in the metal. This spontaneous emission of electrons is called photoemission. The current generated due to this emission is referred to as photocurrent. This phenomenon converts light energy into electrical energy.
FAQs on Photoelectric Effect and Stopping Potential with Work Function and Derivation for JEE
1. How many questions are asked about the photoelectric effect in JEE?
Around 1-2 questions would be asked on this topic for the exam which makes around 4-8 marks in the total of the exam. Preparing this concept will help you gain these easy marks in the total. Preparing previous years’ questions will help you to understand how the questions are framed on related concepts and will give you an idea of how to tackle such problems with ease. Try to search for unusual problems to get insight into the relevance of the photoelectric effect in JEE.
2. What is the physical significance of the photoelectric effect?
The photoelectric effect proves the behaviour of light as a particle-type nature. This is in direct contrast with the theory of the wave nature of light, which is shown in experiments related to interference, diffraction, etc. For studying both the natures of light as a wave and as a particle, this phenomenon of the photoelectric effect is very crucial. It’s true that the energy of the radiation is uniformly distributed along the wavefront of the electromagnetic wave, however, Planck and Einstein proved that the light energy is quantised in the form of wave packets known as quanta.
3. What is the photoelectric effect?
The photoelectric effect is the phenomenon where electrons are ejected from a material's surface when light shines on it, provided the light has enough energy. This ejected electron is called a photoelectron.
4. What is work function?
The work function (Φ) of a material is the minimum energy required to eject an electron from its bound state in the material to the vacuum level. It is usually expressed in electron volts (eV).
5. What is stopping potential?
The stopping potential (V₀) is the minimum potential difference that needs to be applied to stop the most energetic photoelectrons emitted from a material. It is directly proportional to the maximum kinetic energy of the photoelectrons.
6. Relationship between work function and stopping potential:
Einstein's photoelectric equation relates the work function of a material to the stopping potential and the frequency (ν) of the incident light:
hf = Φ + ½ mv₀²
where h is Planck's constant, e is the electron's charge, and m is the electron's mass.
7. Derivation of Einstein's photoelectric equation:
Consider an electron bound in a material with an energy level E below the vacuum level.
When light with frequency ν hits the material, a photon with energy hf can be absorbed by the electron.
If the absorbed energy is greater than or equal to the work function (hf ≥ Φ), the electron can be ejected from the material with kinetic energy KE:
KE = hf - Φ
The stopping potential is applied to oppose the motion of the photoelectrons. At the stopping potential, the kinetic energy of the most energetic photoelectrons is converted into electrical potential energy:
eVo = ½ mv₀²
Combining equations 3 and 4, we get Einstein's photoelectric equation.
8. What is the significance of the photoelectric effect?
The photoelectric effect provided experimental evidence for the quantization of light, supporting the idea of photons.
It has numerous applications, including solar cells, photomultiplier tubes, and electron microscopes.


















