
The chord joining two points
Answer
140.4k+ views
Hint: In this question suppose two points
Complete step-by-step answer:
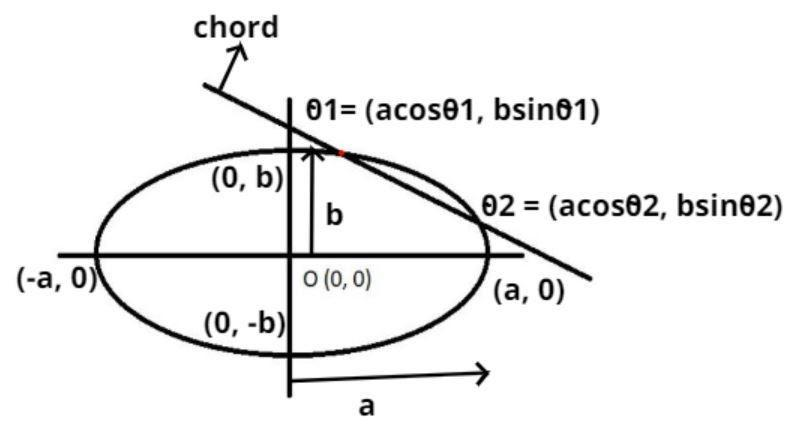
The chord joining two points
As we know that the ellipse is having a center (O) = (0, 0) is also shown in the figure.
Let us suppose the point
Now as we know that the slope between two points
Slope (m) =
So find out the slopes of
Let O =
So let the slope of
Now let the slope of
Now multiply the slopes we have
Now it is given that
Now substitute this value in equation (1) we have,
So multiplication of slopes is (-1) which is the condition of the right angle.
Therefore the chord joining two points
Hence option (B) is correct.
Note: The center of the given ellipse that is
Complete step-by-step answer:

The chord joining two points
As we know that the ellipse is having a center (O) = (0, 0) is also shown in the figure.
Let us suppose the point
Now as we know that the slope between two points
Slope (m) =
So find out the slopes of
Let O =
So let the slope of
Now let the slope of
Now multiply the slopes we have
Now it is given that
Now substitute this value in equation (1) we have,
So multiplication of slopes is (-1) which is the condition of the right angle.
Therefore the chord joining two points
Hence option (B) is correct.
Note: The center of the given ellipse that is
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
Difference Between Mutually Exclusive and Independent Events

Difference Between Area and Volume

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Maths Chapter 6 Permutations and Combinations

Degree of Dissociation and Its Formula With Solved Example for JEE

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Physics Average Value and RMS Value JEE Main 2025

JEE Main Chemistry Question Paper with Answer Keys and Solutions




