Question

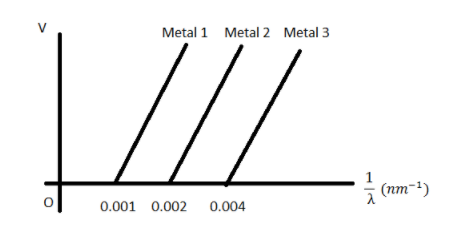
The graph $\dfrac{1}{\lambda }$ and stopping potential (V) of three metals having work function ${{\phi }_{1}}$ , ${{\phi }_{2}}$ and _{${{\phi }_{3}}$} in an experiment of photoelectric effect is plotted as shown in the figure. Which one of the following statements is/are correct? [Here $\lambda $ is the wavelength of the incident ray]
i. Ratio of work functions ${{\phi }_{1}}:{{\phi }_{2}}:{{\phi }_{3}}=1:2:4$
ii. Ratio of work functions ${{\phi }_{1}}:{{\phi }_{2}}:{{\phi }_{3}}=4:2:1$
iii. $\tan \theta \propto \dfrac{hc}{e}$ , where h=Plank’s constant, c =speed of light
iv. The violet colour light can eject photoelectrons from metals 2 and 3.

A. (i), (iii)
B. (i), (iv)
C. (ii), (iii)
D. (i), (ii) and (iv)

Answer 1

Hint: The photoelectric effect is the phenomenon of ejection of electrons from the surface of a metal when the light of a suitable frequency is irradiated on it.
This ejection can be stopped by applying a reverse bias (or stopping potential).

Complete step by step solution:
Formula used:
According to Einstein’s equation of photoelectric effect,
$V=\dfrac{hc}{e}\dfrac{1}{\lambda }-\dfrac{hc}{e{{\lambda }_{0}}}$ --(1)
or, when V = 0, that is the x-intercept $\dfrac{1}{\lambda }=\dfrac{1}{{{\lambda }_{0}}}$ --(2)
Where h is plank’s constant, c is speed of light, e is charge of electron, λ0 is the wavelength above which photoelectric effect does not take place.
$\dfrac{hc}{{{\lambda }_{0}}}=\phi \text{ }\left( \text{work function of the metal} \right)$ --(3)
Work function is the minimum energy of a photon that is required for photoelectric effect to take place. From the graph and (2) and (3), we can see that the work function depends on the x-intercept. The work function is greater for a greater x-intercept.
Thus, we can see in the graph that for metals 1, 2, and 3, the x-intercept is in the ratio of 1:2:4. So, the work functions of metals 1,2, and 3 are in the ratio of 1:2:4.
So, Ratio of work functions ${{\phi }_{1}}:{{\phi }_{2}}:{{\phi }_{3}}=1:2:4$.
So, (i) is correct.
The graph of a linear plot is of the form $y=mx+c$, where m is the slope and c is the y-intercept Comparing this with (1), we see that the slope (or tan θ) is $\dfrac{hc}{e}$. Hence $\tan \theta \propto \dfrac{hc}{e}$ , where h=Plank’s constant, c =speed of light.
So, (iii) is correct.
For ejecting photoelectrons from metal 2 using violet light, the energy of the light photon should be greater than the work function of the metal.
Or, from (2) and (3),
$\dfrac{1}{{{\lambda }_{violet}}}>0.002n{{m}^{-1}}$
${{\lambda }_{violet}}<500nm$ which is true since ${{\lambda }_{violet}}=380nm$.
So, violet light can eject photoelectrons from metal 2.
For ejecting photoelectrons from metal 3 using violet light, the energy of the light photon should be greater than the work function of the metal.
Or, from (2) and (3),
$\dfrac{1}{{{\lambda }_{violet}}}>0.004n{{m}^{-1}}$
${{\lambda }_{violet}}<250nm$ which is not true since ${{\lambda }_{violet}}=380nm$.
So, violet light cannot eject photoelectrons from metal 3.
So, (iv) is wrong.

Therefore (i) and (iii) are correct. So the correct answer is A) (i) and (iii).

Note: The photoelectric effect graphs are very important and many questions such as these can be based on this. All the different plots between various factors and their effects in the photoelectric effect should be properly understood.
By relating the concept, with the graphs, intuition can be developed by the student about such questions which ask for a comparison between metals based on the properties in the graph. This is especially helpful in competitive exams and saves a lot of time.
A student might think that option (iv) is correct after finding that violet light can eject photoelectrons from metal 2, however it does not for metal 3. The option asks for both metals 2 and 3. By solving for metal 2 and marking it as a correct option the student would have made a silly mistake. Students should proceed to complete the whole requirements of the question and not jump to the conclusion.