How to Add and Subtract Unlike Fractions?

Fractions are simply numbers in which the numerator is divided by the denominator. Fractions can get a little complicated when it comes to simple operations like addition and subtraction. When the denominators of given fractions are the same, we can add the numerators directly. However, when the denominators are different, such fractions need to be solved using a different method. Don’t worry, we’ve got you covered. In this chapter we’ll learn about adding and subtracting unlike fractions, addition and subtraction of fractions with unlike denominators, steps for addition and subtraction of unlike fractions, and addition and subtraction of like and unlike fractions.

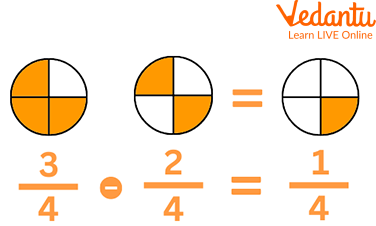
Additions and Subtraction of Unlike Fractions

What are Like Fractions?

Like fractions are fractions that have the same denominators. For example, $\dfrac{10}{19}, \dfrac{11}{19}, \dfrac{13}{19}, and \dfrac{15}{19}$ are like fractions.

What are Unlike Fractions?

Fractions that have different denominators are known as, unlike fractions. For example,

$\left\{\dfrac{2}{3}, \dfrac{5}{6}\right\},\left\{\dfrac{4}{9}, \dfrac{8}{7}\right\}$ are two sets of unlike fractions. The addition and subtraction operations of such fractions are different from those with the same denominator.

Let us see how to perform these operations on such fractions.

Adding and Subtracting Like Fractions

Let us add the fractions with like denominators in numerical terms. In this case, we need to add $\dfrac{1}{5} + \dfrac{2}{5}$. Let us use the following steps to understand the addition.

Step 1: Add the numerators of the given fractions. Here, the numerators are 1 and 2, so it will be 1 + 2 = 3

Step 2: Retain the same denominator. Here, the denominator is 5.

Step 3: Therefore, the sum of $\dfrac{1}{5} + \dfrac{2}{5} = \dfrac{1 + 2}{5}= \dfrac{3}{5}$.

Now, let us subtract the fractions with like denominators in numerical terms. In this case, we need to subtract $\dfrac{2}{5} - \dfrac{1}{5}$. Let us understand the procedure using the following steps.

Step 1: We will subtract the numerators of the given fractions. Here, the numerators are 2 and 1, so it will be 2 - 1 = 1

Step 2: Retain the same denominator. Here, the denominator is 5.

Step 3: Therefore, the difference of $\dfrac{2}{5} - \dfrac{1}{5} = \dfrac{2 - 1}{5} = \dfrac{1}{5}$

How to Add or Subtract Fractions with Unlike Denominators?

When the given fractions have a different denominator, we cannot add the numerators directly without considering the denominators. Below are the steps for the addition and subtraction of unlike fractions. Firstly we will see the steps for addition.

Steps for Addition of Unlike Fractions

Example: Add $\dfrac{1}{5}+ \dfrac{1}{3}$

Ans: For adding unlike fractions, we need to use the following steps

Step 1: Find the denominators Least Common Multiple (LCM).

Here, the LCM of 5 and 3 is 15.

Step 2: Convert the given fractions to like fractions by writing the equivalent fractions for the respective fractions such that their denominators remain the same. Here, it will be $\dfrac{1}{5} \times \dfrac{3}{3}=\dfrac{3}{15}$

Step 3: Similarly, an equivalent fraction of $\dfrac{1}{3}$ with denominator 15 is $\dfrac{1}{3} \times \dfrac{5}{5}=\dfrac{5}{15}$

Step 4: Now that we have converted the given fractions to like fractions, we can add the numerators and retain the same denominator. This will be $\dfrac{3}{15}+ \dfrac{5}{15}=\dfrac{8}{15}$

Steps to Subtract Fractions with Unlike Denominators

Let’s understand it, with an example.

Example: Subtract $\dfrac{5}{6}-\dfrac{1}{3}$

Solution: For subtracting unlike fractions, we need to use the following steps.

Step 1: Find the denominators Least Common Multiple (LCM). Here, the LCM of 6 and 3 is 6.

Step 2: Convert the given fractions to like fractions by writing the equivalent fractions for the respective fractions such that their denominators remain the same. Here, it will be $\dfrac{5}{6} \times \dfrac{1}{1}=\dfrac{5}{6}$

Step 3: Similarly, an equivalent fraction of $\dfrac{1}{3}$ with denominator 6 is $\dfrac{1}{3}$ $\times \dfrac{2}{2}=\dfrac{2}{6}$

Step 4: Now that we have converted the given fractions to like fractions, we can subtract the numerators and retain the same denominator. This will be $\dfrac{5}{6}-\dfrac{2}{6}=\dfrac{3}{6}$. This can be further reduced to $\dfrac{1}{2}$

Solved Examples

Below is an example of some of the problems based on the addition and subtraction of fractions with unlike denominators

Q 1. $\operatorname{Add} \dfrac{5}{9}+\dfrac{3}{2}$

Ans: The fractions $\dfrac{5}{9}$ and $\dfrac{3}{2}$ have different denominators.

LCM of 9 and $2=18$

Multiply $\dfrac{5}{9}$ by $\dfrac{2}{2}$

$\dfrac{5}{9} \times \dfrac{2}{2}=\dfrac{10}{18}$

Multiply $\dfrac{3}{2}$ by $\dfrac{9}{9}$

$\dfrac{3}{2} \times \dfrac{9}{9}=\dfrac{27}{18}$

Now,

$\dfrac{10}{18}+\dfrac{27}{18}=\dfrac{37}{18}$

Thus, upon adding $\dfrac{5}{9}$ and $\dfrac{3}{2}$ we get $\dfrac{37}{18}$ as the result.

Q 2. $\operatorname{Add} \dfrac{1}{9}$ and $\dfrac{7}{3}$

Ans: The fractions $\dfrac{1}{9}$ and $\dfrac{7}{3}$ have different denominators.

LCM of 9 and $3=27$

Multiply $\dfrac{1}{9}$ by $\dfrac{3}{3}$

$\dfrac{1}{9} \times \dfrac{3}{3}=\dfrac{3}{27}$

Multiply $\dfrac{7}{3}$ by $\dfrac{9}{9}$

$\dfrac{7}{3} \times \dfrac{9}{9}=\dfrac{63}{27}$

Now,

$\dfrac{3}{27}+\dfrac{63}{27}=\dfrac{66}{27}=\dfrac{22}{9}$

Thus, upon adding $\dfrac{1}{9}$ and $\dfrac{7}{3}$ we get $\dfrac{22}{9}$ as the result.

Q 3. Subtract $\dfrac{1}{3}$ from $\dfrac{5}{7}$

Ans: $\operatorname{LCM}(3,7)=21$

Multiply $\dfrac{1}{3}$ by $\dfrac{7}{7}$

$\dfrac{1}{3} \times \dfrac{7}{7}=\dfrac{7}{21}$

Multiply $\dfrac{5}{7}$ by $\dfrac{3}{3}$

$\dfrac{5}{7} \times \dfrac{3}{3}=\dfrac{15}{21}$

Hence,$\dfrac{15}{21}-\dfrac{7}{21}=\dfrac{8}{21}$

Thus, the required answer is $\dfrac{8}{21}$.

Worksheet for Addition and Subtraction of Unlike fractions

Below are some of the questions based on adding and subtracting unlike fractions and like fractions.

Q 1. $\dfrac{1}{3}+\dfrac{9}{3}$ (Ans. $\dfrac{10}{3}$)

Q 2. $\dfrac{2}{7}+\dfrac{1}{9}$ (Ans. $\dfrac{25}{63}$)

Q 3. $\dfrac{9}{7}-\dfrac{5}{3}$ (Ans. $\dfrac{-8}{21}$)

Q 4. $\dfrac{8}{3}-\dfrac{1}{3}$ (Ans. $\dfrac{7}{3}$)

Summary

Just like our counting numbers, fractions can also be easily subtracted and added. The rules for addition and subtraction of like and unlike fractions are quite simple and it involves three steps to do so: finding the same common denominator if it's an unlike fraction, then adding or subtracting the numerators. And if the answer is an improper form you have to reduce the fraction into a mixed number. Solving these unlike fractions using the given steps is easy once you grasp the important step for finding the LCM and rationalizing the denominators. You can also download the addition and subtraction of unlike fractions worksheets PDF.