Arzelà-Ascoli Theorem

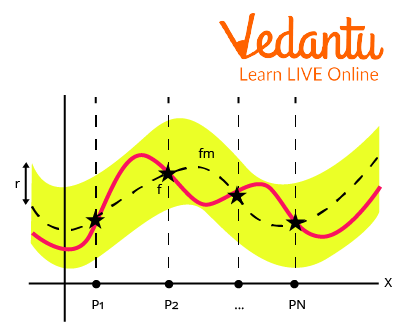
The Curve of Continuous function f

The above figure shows continuous functions f and subsequence $f_{m}$. So, we will be discussing the nature of the subsequence of $f_{m}$. In this article, we will also discuss the application of the theorem along with some of the limitations of the theorem. Some examples related to the theorem will also be solved here.

History of Mathematician

Two great mathematicians are associated with the Arzelà-Ascoli Theorem.

Cesare Arzelà

Name: Cesare Arzelà

Born: 6 March 1847

Died: 12 March 1912

Field: Mathematician

Nationality: Italian

Giulio Ascoli

Name: Giulio Ascoli

Born: 20 January 1843

Died: 12 July 1896

Field: Mathematics

Nationality: Italian

Statement of Arzelà-Ascoli Theorem

If a sequence, $\left\{f_{1}\right\}_{n=1}^{\infty}$ in $C(X)$ is bounded and equicontinuous, then it holds a uniformly convergent subsequence.

Mathematically it can be written as follows:

a) "F $\subset C(X)$ is bounded" means there exists a positive constant $M<\infty$ such that $|f(x)| \leq M$ for each $x \in X$ for all $f \in F$, and

(b) "F $\subset C(X)$ is equicontinuous" means for every $\varepsilon>0$ there exists $\delta>0$ such that for $x, y \in X:$

$d(x, y)<\delta \Rightarrow|f(x)-f(y)|<\varepsilon$ for all $f \in F$

Here, $d$ is the metric on $X$, and $\delta$ depends only on $\varepsilon$.

Proof of Arzelà-Ascoli Theorem

Firstly, we show that the compact metric space $X$ is separable, i.e., it has a finite dense subset $S$.

For a given positive integer $n$ and a point $x \in X$.

Let $B\left(x, \dfrac{1}{n}\right)=\{y \in X: d(x, y)<\dfrac{1}{n}\}$ be the open ball with radius $\dfrac{1}{n}$, centred at $x$.

For a given value of $n$, the set of all these balls as $x$ ranges through $X$ is an open cover of $x$ since $X$ is compact.

Thus, there is a countable subcollection that also covers $X$.

Let $S_{n}$ be the set of centres of the balls in the finite subcollection defined above.

Thus, $S_{n}$ is a finite subset of $X$, consequently " $\dfrac{1}{n}$-dense" in that each point of $X$ lies within $\dfrac{1}{n}$ of a point of the set $S n$.

Therefore, the union $S$ of all the sceneries $S_{n}$ is finite and dense in $X$.

Now, let us find a subsequence of $\left\{f_{n}\right\}$ that converges pointwise on $S$.

Let $\left\{x_{1}, x_{2}, \ldots\right\}$ be the elements of $S$.

As we know. then the numerical sequence $\left\{f_{n}\left(x_{1}\right)\right\}_{n=1}^{\infty}$ is bounded.

Thus, by Bolzano-Weierstrass, we can say that it has a convergent subsequence, and this can be written using double subscripts as:

$\left\{f_{1}, n\left(x_{1}\right)\right\}_{n=1}^{\infty}$

In the same way, we can show that the numerical sequence $\left\{f_{1}, n\left(x_{2}\right)\right\}_{n=1}^{\infty}$ is bounded, so it has a convergent subsequence $\left\{f_{2}, n\left(x_{2}\right)\right\}_{n=1^{\infty}}$.

From the above, we can say that the sequence of functions $\left\{f_{2}, n\right\}_{n=1^{\infty}}$, since it is a subsequence of $\left\{f_{1}, n\right\}_{n=1}^{\infty}$, converges at both $x_{1}$ and $x_{2}$.

Moving in this technique, we obtain a finite collection of sub-sequences of the initial sequence, as:

$f_{1,1} f_{1,2} f_{1,3} \cdots f_{2,1} f_{2,2} f_{2,3} \cdots f_{3,1} f_{3,2} f_{3,3} \cdots \quad f_{n, 1} f_{n, 2} f_{n, 3} \cdots$

Here, the sequence of the $n$-th row converges at $x_{1}, \ldots, x_{n^{\prime}}$ and every row is a subsequence of its previous row.

Therefore, the diagonal sequence in the above, i.e., $\left\{f_{n, n}\right\}$ is a subsequence of the initial sequence $\left\{f_{n}\right\}$ that converges at each point of $S$.

Let $\left\{g_{n}\right\}$ be the diagonal subsequence that is convergent at each point of the dense set $S$ that is created from the overhead step.

Let $\varepsilon>0$ and $\delta>0$ then by equicontinuity of the original sequence, so that $d(x, y)<\delta$ which implies $\left|g_{n}(x)-g_{n}(y)\right|<\dfrac{\varepsilon}{3}$ for each $x, y \in x$ and $n$ is a positive integer.

Specify $M>\dfrac{1}{\delta}$ so that the countable subset $S M \subset S$ that we produced in the initial steps is $\delta$-dense in $X$.

As $\left\{g_{n}\right\}$ converges at each point of $S M$, there exists $N>0$ such that; $n, m>N \Rightarrow\left|g_{n}(s)-g_{m}(s)\right|<\dfrac{\varepsilon}{3}$ for all $s \in S M$.

Consider $x \in X$ then $x$ lies within $\delta$ of some $s \in S M$, so if $n, m>M$ :

$\left|g_{n}(x)-g_{m}(x)\right| \leq\left|g_{n}(x)-g_{n}(s)\right|+\left|g_{n}(s)-g_{m}(s)\right|+\left|g_{m}(s)-g_{m}(x)\right|$

In $R H S$, the first and last terms are $<\dfrac{\varepsilon}{3}$ by our preference of $\delta$.

Thus, for a given $\varepsilon>0$ we can produce $N$ such that for each $x \in X, m, n>N \Rightarrow\left|g_{n}(x)-g_{m}(x)\right|<\dfrac{\varepsilon}{3}+\dfrac{\varepsilon}{3}+\dfrac{\varepsilon}{3}=\varepsilon$.

Therefore, on $X$ the subsequence $\left\{g_{n}\right\}$ of $\left\{f_{n}\right\}$ is uniformly Cauchy, and uniformly convergent.
Hence the proof of the theorem.

Limitations of Arzelà-Ascoli Theorem

• Arzelà-Ascoli Theorem is quite complex to understand as it requires in-depth knowledge of the subject to understand the theorem.

• Besides a wide range of applications, there are many proofs of the theorem for different branches of mathematics, such as metrics spaces, real analysis, topology, etc., which makes it confusing for readers to differentiate between them.

Applications of Arzelà-Ascoli Theorem

• Applications to Functional Analysis in proving compactness of equicontinuous family of functions.

• Applications to Ordinary Differential Equations in proving Peano’s Existence Theorem.

• Applications to Complex Analysis in Riemann’s Mapping Theory.

Solved Examples

1. What are equicontinuous functions as used in the Statement of Arzelà-Ascoli Theorem?

Ans: In mathematical analysis, a family of functions is said to be equicontinuous if all the functions are continuous and they have equal variation in the given neighbourhood of the family of functions. In particular, the concept applies to countable families and thus sequences of functions.

Important Points/Formulas to Remember

• For the subsequence of any function to be convergent, the sequence of uniform functions must be defined in closed and bounded intervals.

• An interval is said to be bounded if there exists an upper and lower bound for the interval.

• If the value of a function coincides with a common value at all values for a given interval, then the function is said to be convergent.\

Conclusion

The Arzelà-Ascoli theorem allows us to study compact sets in function spaces in Mathematical Analysis. This theorem shortens the checking of compactness for subsets of spaces of continuous function. Moreover, a lot of the topological spaces used in Real Analysis, Complex Analysis, and Functional Analysis are spaces of functions.