What is Bundling in Maths with Examples and Steps
For young elementary students, understanding place value is an important maths concept. They need to understand that a digit's value is determined by where it is in a number. A digit's place value in a number is its value in that specific place. For example- the place value of the digit 1 in the number 152 is the hundredth. The number 5 and the number 2 have five at tens and two at one place, respectively. Students can better understand number properties and how larger numbers can be divided into smaller numbers by learning how to bundle numbers. In this article, we will learn about bundling in maths by understanding place value.
Define Bundling
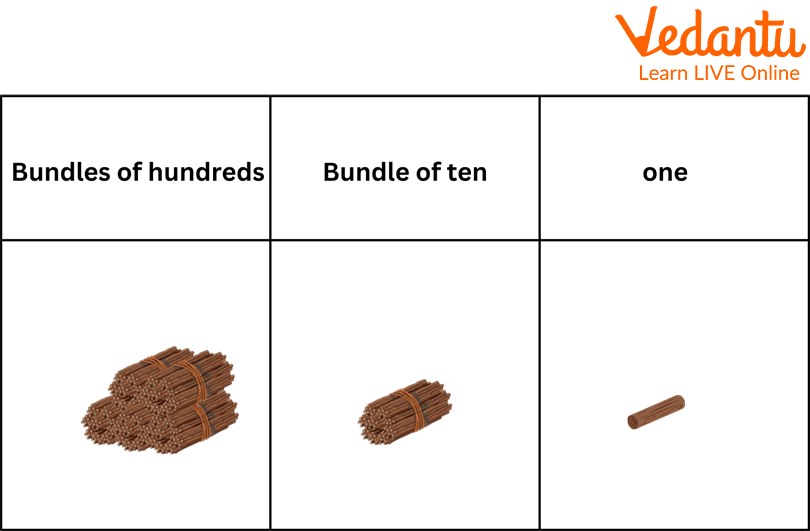
Bundling is also known as a grouping. This method of grouping numbers by combining smaller units to get a bigger one. For example, adding ten ones gives one ten. Adding 10 tens equals one hundred.
Place Value Using the Bundle of 10
We know that the hundreds, tens, and ones are represented by the three digits of a three-digit number.
Consider the following examples:
The numbers 100, 200, 300, 400, 500, 600, 700, 800, and 900 denote one, two, three, four, five, six, seven, eight, or nine hundred. A bundle of tens, or a hundred, can be considered a group of tens (with 0 tens and 0 ones).
3-Digit Number Using Bundling
Use bundles of one hundred with no tens or ones to represent numbers such as 100, 200, 300,... 900.
Let’s see an example of price bundling: Let us assume 1 stick costs 10 rupees. In one bundle, there are 100 sticks. Therefore, 200 is equal to 2 bundles of 10 tens. 3 bundles of 10 tens equal 300.
3-digit Number Bundling
Here we can see that there are 3 bundles of 100, which mean 300, 5 bundles of 10, that is 50, and 6 one.
Therefore the number is 356.
Hence, the cost will become 3560 rupees.
Let us see another example of bundling 451. We know that 4 is at a hundred places, 5 is at tens place, and 9 is at ones. So, 400 is equal to 4 bundles of 10 tens, 50 is equal to 5 bundles of tens, and 1 is equal to one single stick.
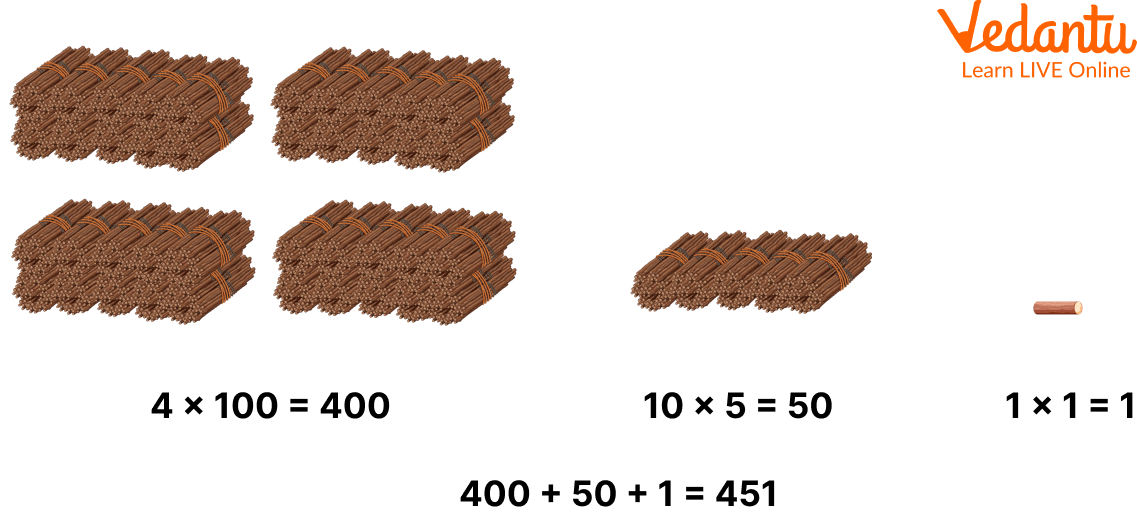
Bundling of 451
Solved Examples
Q 1. Represent number 279 using bundles.
Ans: We have given 279, of which 2 is at hundred places, 7 is at tens place, and 9 is at one’s place. Therefore, 100 is equal to 10 bundles of 10 tens, 70 is equal to 7 bundles of tens, and 9 is equal to nine single sticks.
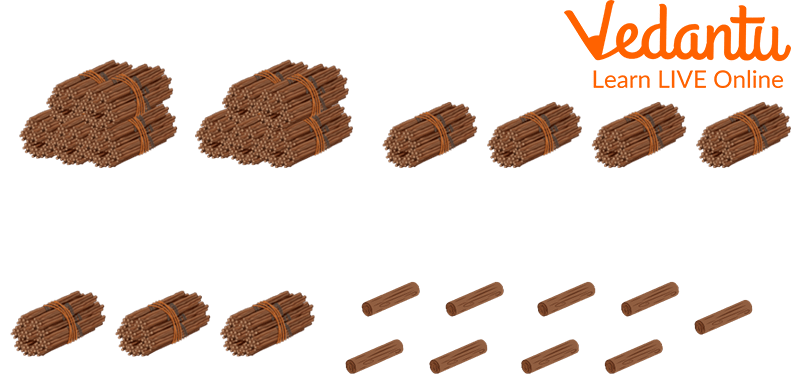
179 Using Bundles of Ten
Q 2. Represent number 93 using bundles of ten.
Ans: We have given a two-digit number 93, of which 9 is at tens place, and 2 is at one’s place. Therefore, 90 is equal to 9 bundles of tens, and 3 is equal to three single sticks.
93 Using Bundles of Ten
Practice Problem
Q 1. Count the number of sticks and write the number.
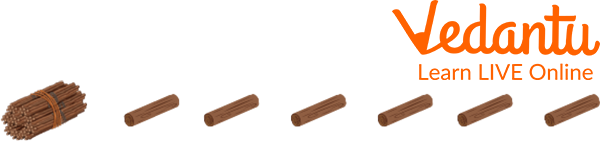
Bundling of Sticks
Ans: 16
Q 2. Count the number of sticks and write the number.
Bundling of Sticks
Ans: 57
Summary
In this article, we have learned about the place value of a number. With the help of bundling, we have learned how easily we can understand the place values of the numbers. And as we know, bundling in simple terms is the grouping of the numbers and finding the values using it. With the help of sticks, we have portrayed this article to make it easy to understand the concept of place value. Place value is the value of a digit according to its position in the number such as ones, tens, hundreds, and so on. Some solved examples and practice questions are given in this article to make your understanding of numbers easier.
FAQs on Understanding Bundling in Place Value
1. What is bundling in mathematics?
Bundling in mathematics is the process of grouping equal quantities together to make counting and calculations easier, especially in place value and early arithmetic. It is commonly used in topics like place value, addition, and base-ten system.
- In the base-ten system, 10 ones = 1 ten.
- 10 tens = 1 hundred.
- Bundling helps students understand how numbers are structured and regrouped.
2. How does bundling help in understanding place value?
Bundling helps in understanding place value by showing how smaller units combine to form larger units in the base-ten system. Each place represents a bundle of the previous place.
- 10 ones = 1 ten
- 10 tens = 1 hundred
- 10 hundreds = 1 thousand
3. What is an example of bundling in addition?
Bundling in addition occurs when we regroup 10 or more units into a larger place value. This is also called regrouping or carrying.
- Example: 27 + 15
- Add ones: 7 + 5 = 12
- Bundle 10 ones into 1 ten.
- Write 2 ones and carry 1 ten.
- Add tens: 2 + 1 + 1 (carried) = 4 tens
4. What is the difference between bundling and regrouping?
Bundling and regrouping describe the same mathematical idea, but bundling emphasizes grouping objects while regrouping refers to the written calculation process. Both involve combining smaller units into larger ones.
- Bundling: Physical or visual grouping (e.g., 10 sticks tied together).
- Regrouping: Numerical process in addition or subtraction.
5. How do you use bundling in subtraction?
Bundling in subtraction involves breaking a larger unit into smaller units, also called borrowing or decomposing. This happens when the top digit is smaller than the bottom digit.
- Example: 52 − 38
- Cannot subtract 8 from 2.
- Unbundle 1 ten from 5 tens → becomes 4 tens and 12 ones.
- 12 − 8 = 4
- 4 tens − 3 tens = 1 ten
6. Why is bundling important in early maths learning?
Bundling is important in early maths because it builds a strong understanding of number sense and place value. It helps learners see how numbers are constructed.
- Improves counting accuracy.
- Supports addition and subtraction skills.
- Prepares students for multiplication and division.
7. What is bundling in the base-ten number system?
Bundling in the base-ten number system means grouping quantities in sets of 10 to move to the next place value. Each place is ten times the value of the place to its right.
- 10 ones = 1 ten
- 10 tens = 1 hundred
- 10 hundreds = 1 thousand
8. Can you give a real-life example of bundling?
A real-life example of bundling is packing pencils into boxes of 10 to make counting easier. Instead of counting 37 pencils one by one, we bundle them.
- 37 pencils = 3 bundles of 10
- Plus 7 single pencils
9. How does bundling relate to multiplication?
Bundling relates to multiplication because multiplication represents repeated grouping of equal quantities. Each group can be seen as a bundle.
- Example: 4 × 10 means 4 bundles of 10.
- 4 × 10 = 40
10. What are common mistakes students make with bundling?
Common mistakes in bundling include forgetting to regroup correctly or misunderstanding place value. These errors often occur in addition and subtraction.
- Not carrying when the sum exceeds 9.
- Borrowing incorrectly in subtraction.
- Confusing tens and ones places.
