Decimal Number System

We have learnt that decimals are the extension of our number system. We also know that decimal numbers can be considered fractions with denominators such as 10, 100, 1000, etc. The numbers expressed in decimal form are called decimal numbers or decimals. This article discusses parts of a decimal number, along with decimal number examples. Some practice problems are given underneath for a better understanding of the topic.

For example, 5.2, 4.19, 11.83, etc.

Definition of Decimal Numbers

A decimal number is a number that has a decimal point between the whole number and the fractional part. The point is used to segregate these two parts of the decimal. Thus, it is called a decimal point. The numbers after the decimal point are always smaller than 1.

For example, in the decimal numbers 11.128 and 2.656, 11 and 2 are whole numbers, whereas 128 and 656, following the decimal point, are fractional parts of the number. The fractional part of the decimal number is smaller than 1. In the above examples, the fractional parts of the numbers are 0.128 and 0.656.

Parts of a Decimal Number

Generally, there are two parts of a decimal number:

1. Whole Number Part

The digits lying to the left of the decimal point form the whole number part. The places begin with ones, then tens, then hundreds, then thousands and so on.

For example, if we have to write the numbers two hundred one and 7 tenths numerically, then we will write it as 201.7. Here, 201 is considered a whole number.

2. Decimal Part

The decimal point, together with the digits lying on the right of the decimal point, form the fractional part of the decimal part; hence, it is always smaller than 1. The places begin with tenths, then hundredths, then thousandths and so on………

For example, 12.34 has .34 present in its decimal part.

Types of Decimal Numbers

There are two types of decimal numbers, namely:

• Terminating

The decimal numbers have only a finite number of digits after the point or in the decimal part. These are also called exact decimal numbers. For example, 12.38, 2.39, 7.3, etc.

• Non-terminating

In this type, decimal numbers have an infinite number of digits after the decimal point. Further, non-terminating decimal numbers can be classified into two categories, namely,

• Repeating or Recurring Decimal Numbers: A repeating decimal or recurring decimal is a decimal representation of a number whose digits are periodic (repeating its values at regular intervals) and the infinitely repeated portion is not zero.

For example, 123.24242424…….

• Non-repeating or non-recurring decimal numbers: These decimals are decimal fractions that will never end and, after the decimal point, even predictably repeat one or more numbers. Non-terminating repeating decimals are rational numbers, and we can represent them as p/q, where q will not equal 0.

For example, 124.1612014……

Decimal Number Examples

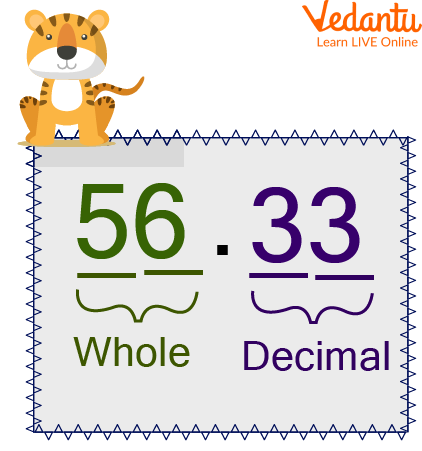
Q 1. Identify the whole part and the decimal part:

1. 56.33

Ans: Whole part= 56

Decimal part= 0.33

Decimal Number Example

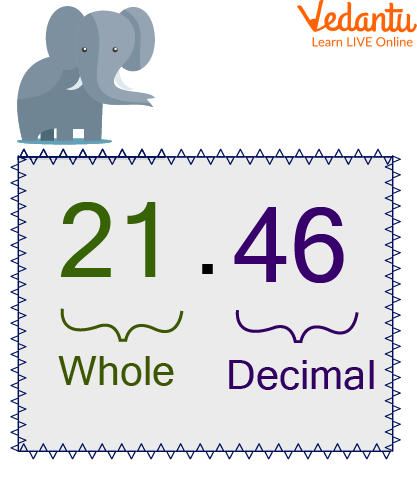
2. 21.46

Ans: Whole part= 21

Decimal part= 0.46

Decimal Number Example

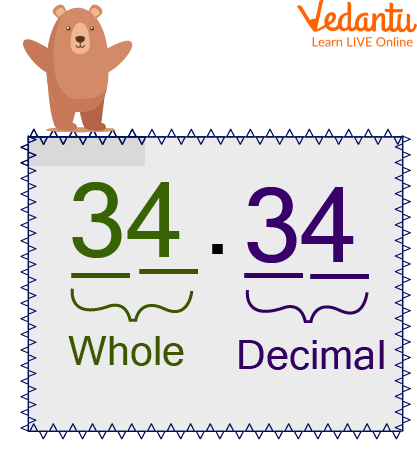
3. 34.34

Ans: Whole part= 34

Decimal part= 0.34

Decimal Number Example

Practice Questions

Q1. Write the place and place value of the underlined digits:

(a) 8.13

(b) 53.31

(c) 100.92

(d) 11.381

(e) 0.004

Ans.

1. Hundredths

2. Tens

3. Tenths

4. Hundredths

5. Tenths

Q2. Express each of the following as decimals.

(a) $\dfrac{37}{100}$

(b) $\dfrac{11}{1000}$

(c) $\dfrac{9}{100}$

(d) $\dfrac{739}{10}$

(e) $\dfrac{1234}{1000}$

(f) $\dfrac{495}{10}$

Ans.

1. 0.37

2. 0.011

3. 0.09

4. 73.9

5. 1.234

6. 49.5

Summary

In this article, we have learned about the definition of decimal numbers, the parts of a decimal number, the types of decimal numbers, and how the decimal point is used to separate the whole part from the fractional or decimal part. Some decimal number examples are discussed to explain to the student the difference that arises in the placement of decimal points at different places. We also learned about the place value of the numbers in the whole number part and the decimal part of a number.