Division Factor

Any integer that divides a number into another number equally is considered a factor. In the division problem, for instance, \[10 \div 5 = 2\],10 has the factors 1, 2, 5, and 10. These factors can all be divided into equal groups, such as two groups of five, two groups of two, and one group of ten, which is the same as two groups of five, two groups of two, and one group of ten, and one group of ten, respectively.

Division Factor

The numbers that can divide a number exactly are called factors. There is, therefore, no remainder after division. The numbers you multiply together to obtain another number are called factors. A factor is therefore another number's divisor.

Divisor vs. Factor

Any number that divides another number is known as the divisor. However, a factor is a divisor that completely divides the integer and leaves no remainder. Therefore, any factor of a number is also its divisor. But not all factors are divisors; a number's divisors are all of its factors. In the previous illustration, the components of 20 are 4 and 5. However, dividing 20 by 3 does not result in a perfect division of the number.

How to Divide Factors?

What are the Factors of 18 (Division Method)?

Steps to find division factors of 18:

STEP 1: Using division laws, we determine the number's smallest exact prime divisor (factor). Here, the number 18 is even. It can be divided by 2. Therefore, 2 divides 18 without leaving a remainder. So, the least prime factor of 18 is 2.

STEP 2: Is to divide the supplied number (18) by its smallest prime factor, which equals 9.

STEP 3: Next, we identify the derived quotient's prime factors. Repeat steps 1 and 2 until the quotient is a prime number. Here, the quotient is 9, therefore \[9 = 3 \times 3\].

We stop the operation here because 3 is the quotient. Consequently \[18 = 2 \times 3 \times 3\].Thus, the factors of 18 are 1,2, 3, 6, 9,18.

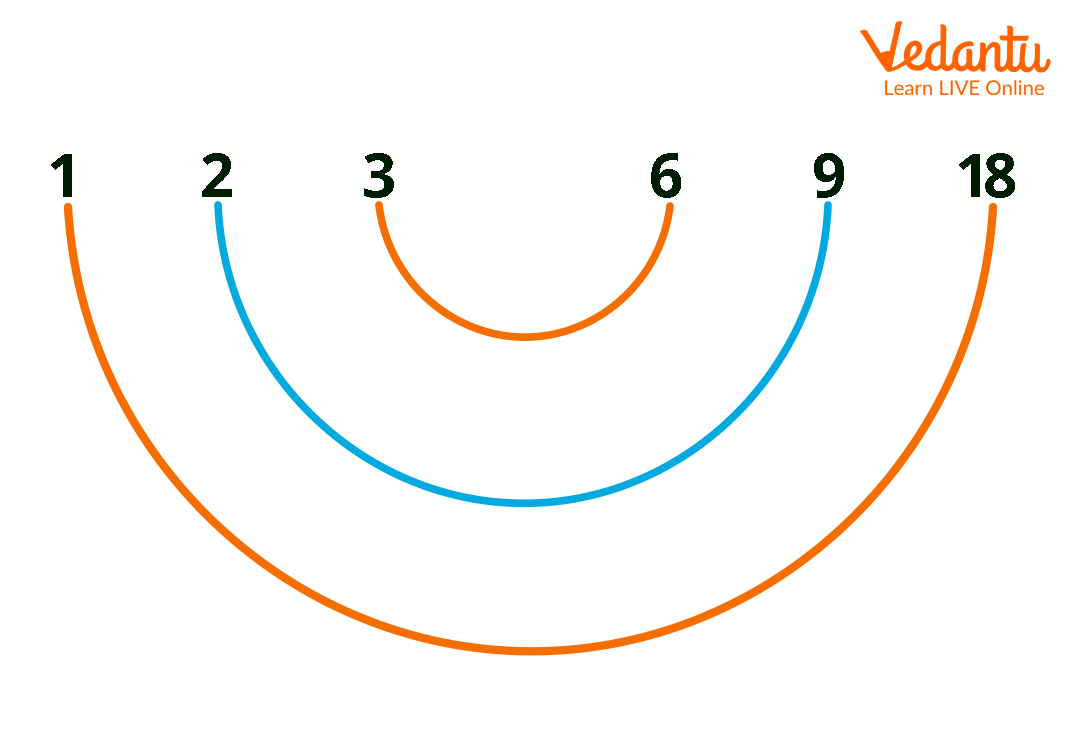
Pairs for Factors of 18

Solved Examples

Example 1: List the factors of 18 and their corresponding factor pairs in Example 1.

Ans:

\[\begin{array}{l}1 \times 18 = 18\\2 \times 9 = 18\\3 \times 6 = 18\end{array}\]

Therefore, the factors of 18 are 1, 2, 3, 6, 9, and 18.

Pairs of the factors of 18 are (1,18), (2,9), and (3,6)

Example 2: Find the common factors of 25 and 24.

Ans: The factors of 25 are as follows: 1, 5, and 25.

The factors of 24 are as follows: 1, 2, 3, 4, 6, 8, 12, and 24.

Thus, the common factor of 25 and 24 is 1.

Example 3: Find the factors of 72.

Ans: 72 is represented as the product of the following additional numbers:

\[\begin{array}{l}1 \times 72 = 72\\2 \times 36 = 72\\3 \times 24 = 72\\4 \times 18 = 72\\6 \times 12 = 72\\8 \times 9 = 72\\9 \times 8 = 72\end{array}\]

Since multiplication is currently being repeated, it must be stopped.

Conclusion

Factors and multiples by using division facts, for instance, are employed while handling money, sorting objects into boxes, looking for patterns in numbers, resolving ratios, or expanding or contracting fractions. In Mathematics, a factor is an integer that divides another number equally by itself while producing no remainder. We frequently come across factors and multiples.