




An Introduction to Estimated Sum in Maths
Would you like to know more about the estimated sum for kids? We've got what you're looking for! This article will go over some information on what is estimated sum and how to estimate the sum. When attempting to solve a problem, estimation entails putting these conversion factors to use. Estimating can reduce some of the stress associated with needing to accurately compute the total of two numbers while solving problems with sums. Let’s learn more about it ahead!
How to Estimate the Sum?
There are many estimation methods in Maths. Our method for estimating an amount to the tenth consists of two steps:
STEP 1: Round the numbers to the nearest tenth.
If the number ends in 1, 2, 3 or 4, we round down.
If the number ends in 5, 6, 7, 8 or 9, we round up.
STEP 2: In the next step, add the rounded numbers to get a final estimated sum.
The same procedure can be used to estimate the sum for hundreds. However, instead of rounding off to the nearest tens, here we will be rounding off to the nearest hundreds.

Estimating Sums

Child Estimating the Sum of Candies
Ensure that your child is aware of the distinction between an actual sum and estimated sum! Actual sum is the sum of two numbers without rounding off, whereas in estimated sums, the numbers are rounded off to their nearest tens or hundreds. In the case of two-digit numbers, we can only estimate the sum to the nearest tens. Let us understand this concept better with a few examples.
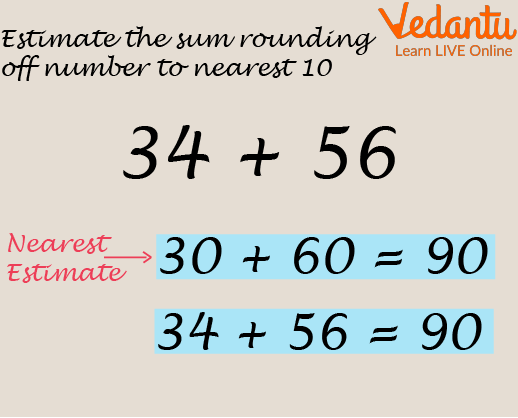
Estimate the Sum to the Nearest Tens
For Example-
Let us estimate 38 + 23.
Solution: 38 is nearer to 40 than 30.
So, 38 is rounded up to 40.
23 is closer to 20 than 30.
So, 23 is rounded down to 20.
Hence, the result is 60.
Let us round off the numbers 389 and 535 to the nearest 100s and find the estimated and the actual sum.
Solution:
535 is closer to 500 than 600
and 389 is closer to 400 than 300
535 $\Rightarrow$ 500
389 $\Rightarrow$ 400
500 + 400 = 900
Hence, 400 + 500 = 900 is the estimated sum.
To find the actual sum:
389 + 535 = 924
924 is the actual sum.
Do remember these estimation methods in Maths so that if you see any sort of problem in your homework, there's no way you'll forget!
Solved Examples
Q 1: Calculate the estimated sum of 72 and 48.
Ans: 72 is rounded off to 70 as it is closer to 72.
48 is rounded off to 50 as it is closer than 40.
72 $\Rightarrow$ 70
48 $\Rightarrow$ 50
So, 72 is nearest to 70 and 48 is nearest to 50
70 + 50 = 120
Thus, the estimated sum = 120.
Q 2: Determine the estimated sum and the actual sum of 87 and 79.
Ans: 87 is rounded off to 90 as it is closer than 80.
79 is rounded off to 80 as 80 is closer than 70.
87 $\Rightarrow$ 90
79 $\Rightarrow$ 80
So, 87 is nearest to 90 and 79 is nearest to 80.
90 + 80 = 170
Thus, the estimated sum = 170
The actual sum of 87 and 79
87 + 79 = 166
Thus, the actual sum = 166.
Q 3: Find estimated sum of 586 and 120 to the nearest hundred.
Ans: 586 is rounded off as 600 as it is the nearest 100.
120 is rounded off as 100 is 100 is nearer to 120 than 200.
586 $\Rightarrow$ 600
120 $\Rightarrow$ 100
The nearest number to 600 is 586, and the nearest number to 100 is 120
600 + 100 = 700
Thus, the estimated sum to the nearest hundred = 700
Q 4 Tia and John collect marbles. Tia has 335 and John has 442 marbles. Decide which estimate is better for determining the number of marbles they own jointly.
Ans: Here, we’ll round off the digits to the nearest tens.
Tia's marble count is 335 rounded up to 340 as it is the nearest 10
John’s Marble count is 442 rounded up to 440 as 440 is the nearest 10
Total number of marbles = 340 + 440 = 780
∴The estimated answer is 780.
Q 5 A train terminal has 12 platforms. Approximately 115 trains depart each day from each platform. Calculate how many trains leave the terminal on a daily basis and give an approximation.
Ans: The total number of trains can be calculated by multiplying the number of platforms and the Number of trains leaving each platform
Total number of trains=12×115
12 is rounded off to 10 as it is the closest, and 115 is rounded as 120 as it is the nearest 10
Therefore, the estimated value=10×120= 1200.
Practice Questions
Q 1 Give the estimated sum of 27 + 44 to the nearest 10.
Ans: 70.
Q 2 Give the estimated sum of 54 + 38 to the nearest 10.
Ans: 90.
Q 3 Give the estimated sum of 41 + 38 to the nearest 10.
Ans: 80.
Summary
Now, your child has learnt what is estimated sum and the difference between actual sum and estimated sum. The examples provided in this article will encourage children to have a good understanding of the magnitude of numbers rather than overly relying on mathematical procedures to achieve an answer. Instead of memorising estimation methods in Maths, make sure your ward practises the problems.
FAQs on Estimated Sum
1. How do you estimate total sum and differences?
To estimate the sum, round off all the integers to the same place value and then add them. To calculate the difference, round all of the numbers to the same place value and then subtract them.
2. What is the benefit of estimating?
Estimation saves time and money before investing in anything. We can gain from estimating since we can save money and time on large projects. Estimation assists in performing a reality check on any project.
3. How can I make my child an expert in estimated sums?
To make your child an expert in estimating sums, he or she has to understand concepts like what is estimated sum, the difference between actual sum and estimated sum and our estimation method in Maths. The child should also practice lots of sums from estimated Maths examples to become an expert in solving these sums.











