Stepwise Guide to Calculating the HCF of 8 and 12
The Highest Common Factor (HCF) of two numbers is the highest possible number that divides both numbers completely. The Highest Common Factor (HCF) is also called the Greatest Common Divisor (GCD). The HCF of two numbers can be determined by a variety of methods. Using the prime factorisation method is one of the easiest ways to get the HCF of two or more numbers. In this article, we will learn about HCF and how to find it. Also, we will see the HCF chart.
Factors
A number completely divides the given number without leaving any remainder is said to be the factor of that number. A number can have either positive or negative factors. For example, let's find the factors of 8. Since the number 8 is completely divisible by 1, 2, 4, and 8, we can list these as the positive factors of 8. The product of two negative numbers is a positive number, hence (-1) (-8) = 8 and (-2) (-4) = 8. In addition, the number 8 has negative factors, which can be written as -1, -2, -4, and -8.
Common Factors
When two or more numbers are exactly divided by the same number(s), those common divisors are known as common factors of the given numbers. Or we can say a common factor is a number that divides a pair of two or more numbers exactly without leaving any remainder. For example, the factors of 18 are 1, 2, 3, 6, 9, and 18.
Let's find out the common factors of the given numbers: 8, 12, 15, and 18.
Step 1: First of all we will find out the factors of the given numbers.
Factors of 8 = 1, 2, 4, and 8.
Factors of 12 = 1, 2, 3, 4, 6, and 12.
Factors of 15 = 1, 3, 5, and 15.
Factors of 18 = 1, 2, 3, 6, 9, and 18.
Step 2: Now, we will check the common factors (8, 12, 15, 18) = 1
So, 1 is the common factor of 8,12,15, and 18.
What is HCF?
The HCF (Highest Common Factor) of two or more numbers is the highest number among all the common factors of the given numbers. In simple words, the HCF (Highest Common Factor) of two natural numbers x and y is the largest possible number that divides both x and y.
Using the above definition, the HCF of a few sets of numbers can be listed as follows:
HCF of 60 and 40 is 20, i.e., HCF (60, 40) = 20
HCF of 100 and 150 is 50, i.e., HCF (150, 50) = 50
HCF of 144 and 24 is 24, i.e., HCF (144, 24) = 24
HCF of 17 and 89 is 1, i.e., HCF (17, 89) = 1
Steps to Find HCF
Let us understand the steps to find the HCF using two numbers by using the prime factorisation method:
What is the HCF of 8 and 12?
Step 1: First of all, find factors of 8 and 12 separately.
Factors of 8 = 1, 2, 4, and 8.
Factors of 12 = 1, 2, 3, 4, 6, and 12
Step 2: Now, find the common factors of 8 and 12.
The common factors of 8 and 12 are 1, 2, and 4.
Step 3: Now check the highest number among the common factors.
Among these numbers, 4 is the highest (largest) number.
So, the HCF of 8 and 12 is 4.
This is written as HCF (8, 12) = 4.
Observe the following HCF chart to understand this concept.
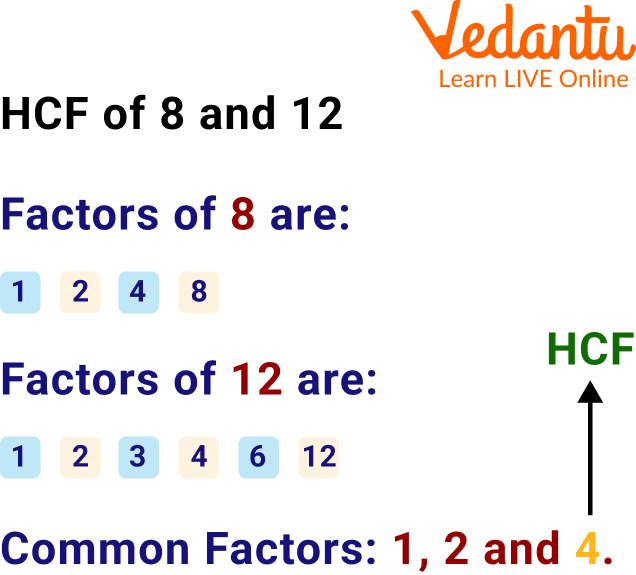
HCF of 8 and 12
Solved Example
Q1. Find the HCF of 24 and 32 by prime factorisation.
Ans: Prime factorisation of 24 and 32 is $(2 \times 2 \times 2 \times 3)$ and $(2 \times 2 \times 2 \times 2 \times 2)$ respectively.
As visible, 24 and 32 have common prime factors.
Hence, the HCF of 24 and 32 is $2 \times 2 \times 2=8$.
Q2. What is the HCF of 20 and 30?
Ans: Factors of 20: 1, 2, 4, 5, 10, 20
Factors of $30: 1,2,3,5,6,10,15,30$
There are 4 common factors of 20 and 30, which are $1,2,10$, and 5.
Therefore, the highest common factor of 20 and 30 is 10.
Practice Problem
Q1. What is HCF of 135 and 225?
Ans: 45
Q2. What is HCF of 65 and 117?
Ans: 13
Conclusion
From the HCF of two numbers examples, we have learned to find the factors and then take the common factors. Then after that multiplication of the common factors will give us the HCF. We can use the division method to find the HCF. Hence, after going through this article we have made our concepts of HCF very clear in both methods and now we know how to find the HCF of two numbers. With the examples and practice problems, we will get more clarity on the topic. So after reading the article, go through the practice problem to have a better understanding.
FAQs on HCF of 8 and 12 Explained
1. What is the HCF of 8 and 12?
The HCF (Highest Common Factor) of 8 and 12 is 4. To find this, you can list the factors of each number. The factors of 8 are 1, 2, 4, and 8. The factors of 12 are 1, 2, 3, 4, 6, and 12. The highest factor that appears in both lists is 4.
2. How do you find the HCF of 8 and 12 using the prime factorisation method?
To find the HCF of 8 and 12 using the prime factorisation method, you break down each number into its prime factors:
The prime factors of 8 are: 2 × 2 × 2.
The prime factors of 12 are: 2 × 2 × 3.
Next, you identify the common prime factors in both lists, which are two '2's. Multiplying these common factors gives you the HCF: 2 × 2 = 4.
3. What is the step-by-step process to find the HCF of 8 and 12 using the division method?
To find the HCF of 8 and 12 using the division method, follow these steps:
Divide the larger number (12) by the smaller number (8). This gives a quotient of 1 and a remainder of 4.
Now, make the remainder (4) the new divisor and the previous divisor (8) the new dividend.
Divide 8 by 4. This gives a quotient of 2 and a remainder of 0.
The last non-zero divisor is the HCF. Therefore, the HCF of 8 and 12 is 4.
4. What is the difference between the HCF and LCM of 8 and 12?
The primary difference is what they measure. The Highest Common Factor (HCF) of 8 and 12 is the largest number that can divide both numbers without a remainder, which is 4. In contrast, the Least Common Multiple (LCM) is the smallest number that is a multiple of both 8 and 12, which is 24. Essentially, the HCF is a divisor, while the LCM is a multiple.
5. Where can the concept of HCF be used in a real-life situation?
The concept of HCF is useful for problems involving equal distribution or grouping. For example, if you have 8 chocolate bars and 12 biscuit packets and want to create the maximum number of identical gift bags, you would use the HCF. The HCF of 8 and 12 is 4. This means you can create a maximum of 4 identical gift bags, each containing 2 chocolate bars (8 ÷ 4) and 3 biscuit packets (12 ÷ 4).
6. What is the relationship between the HCF and LCM of 8 and 12?
For any two numbers, there is a fundamental relationship: the product of the two numbers is equal to the product of their HCF and LCM. For 8 and 12:
Product of the numbers = 8 × 12 = 96.
HCF(8, 12) = 4 and LCM(8, 12) = 24.
Product of their HCF and LCM = 4 × 24 = 96.
This confirms the rule: HCF × LCM = Product of the Numbers, which is a key concept in the CBSE syllabus.
7. If the HCF of 8 and 12 is 4, what does this number fundamentally represent?
An HCF of 4 fundamentally represents the largest possible unit size that can measure or divide both 8 and 12 perfectly. Imagine you have two ribbons, one 8 inches long and the other 12 inches long. The HCF, 4, is the length of the longest possible piece you can cut both ribbons into without having any leftover material. It signifies the greatest common scale between the two quantities.
8. What are all the common factors of 8 and 12?
To find the common factors, we first list all factors for each number:
Factors of 8 are: 1, 2, 4, 8.
Factors of 12 are: 1, 2, 3, 4, 6, 12.
The numbers that appear in both lists are the common factors. Therefore, the common factors of 8 and 12 are 1, 2, and 4. The 'highest' of these is the HCF.
