Step-by-Step Guide: How to Identify Number Patterns
Introduction to Patterns
The study of mathematics includes numbers and the different patterns in which they are listed. There are different patterns in mathematics, such as number patterns, image patterns, logic patterns, word patterns, and so on. The number pattern is the most commonly used one since students are aware of even numbers, odd numbers, skip counting, etc., which helps in understanding these patterns easily. In this article, we will learn about number patterns, fill in the missing numbers and ask some questions based on them.
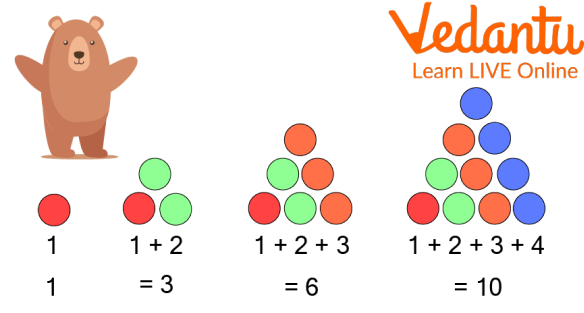
Patterns example
Definition of Patterns
Patterns include a series or sequence that generally repeats itself. The patterns we observe in our daily lives are those of colours, actions, shapes, numbers, etc. They can be related to any event or object and are finite or infinite. In mathematics, patterns are numbers arranged in a sequence related to each other in a specific rule. These rules define a way to calculate or solve problems. For example, in a sequence of 3,6,9,12,_, each number is increasing by 3. So, according to the pattern, the last number will be 12 + 3 = 15.
Numbers Patterns in Math
A number pattern is the most common type in mathematics, where a list of numbers follows a certain sequence based on a rule. The types of number patterns are algebraic or arithmetic patterns, geometric patterns, and the Fibonacci pattern.
For example, let us find the missing numbers in the series: 4, 8, ___, 16, 20, ___ .
Ans: In the above pattern, we can see that each number increases by 4. Hence, the rule followed for this pattern is adding 4 to the last term to get the next term. We can find the missing numbers using this pattern. Therefore, the missing numbers are 8 + 4 = 12 and 20 + 4 = 24.
Rules for Patterns
To create a complete pattern, a set of rules must be considered. To apply the rule, we need to understand the nature of the sequence and the difference between the two consecutive numbers. It takes some guesswork and checking to see whether the rule works throughout the whole series.
There are two basic divisions to finding out the rules in number patterns:
When the numbers in the given pattern get larger, they are said to be in ascending order. These patterns usually involve addition or multiplication.
When the numbers in the given pattern get smaller, they are said to be in descending order. These patterns usually involve subtraction or division.
Solved Examples
Find the missing numbers.
Q1. 1, 2, 3, 4, 5, 6, ____, ____, ____, ____.
Ans: 7, 8, 9, 10.
Pattern rule: Add one to each number to find the next number.
6 + 1 = 7
7 + 1 = 8
8 + 1 = 9
9 + 1 = 10
Q2. 4, 7, 10, 13, 16, ____, ____, ____, ____.
Ans: 19, 22, 25, 28.
Pattern rule: Add 3 to each number to find the next number.
16 + 3 = 19
19 + 3 = 22
22 + 3 = 25
25 + 3 = 28
Q3. 27, 25, 23, 21, 19, ____, ____, ____, ____.
Ans: 17, 15, 13, 11.
Pattern rule: Subtract 2 from each number to find the next number.
19 - 2 = 17
17 - 2 = 15
15 - 2 = 13
13 - 2 = 11
Q4. 1050, 1060, 1070, 1080, ____, ____, ____, ____.
Ans: 1090, 2000, 2010, 2020.
Pattern rule: Add 10 to each number to get the next number.
1080 + 10 = 1090
1090 + 10 = 2000
2000 + 10 = 2010
2010 + 10 = 2020.
Q5. 31, 28, 25, 22, ____, ____, ____, ____.
Ans: 19, 16, 13, 10.
Pattern rule: Subtract 3 from each number to get the next number.
22 - 3 = 19
19 - 3 = 16
16 - 3 = 13
13 - 3 = 10.
Practice Questions
Q 1. Fill in the missing numbers.
101 __ 103
Ans: 102
104 __ 106
Ans: 105
107 __ 109
Ans: 106
110 __ 112
Ans: 111
113 __ 115
Ans: 114
Q 2. Identify the number pattern and fill in the missing numbers.
113, 122, 131, 140, ____, ____, ____, ____.
Ans: 149, 158, 167, 176.
890, 880, 870, 860, ____, ____, ____, ____.
Ans: 850, 840, 830, 820.
7, 14, 21, 28, ____, ____, ____, ____.
Ans: 35, 42, 49, 56.
5, 10, 15, 20, ____, ____, ____, ____.
Ans: 25, 30, 35, 40.
127, 125, 123, 121, ____, ____, ____, ____.
Ans: 119, 117, 115, 113.
Summary
First of all, we have seen the pattern. Then moving further, I learned about the number pattern. i.e. It can be defined as a pattern or sequence in a series of numbers. This pattern generally establishes a common relationship between all numbers present among them. For example, $0,5,10,15,20,25$, 30 (differs by a value of 5 ). There are different ways to find the missing numbers in a pattern. In the end, we have seen some solved examples and practice problems to have a better command of the topic.
FAQs on Discover and Solve Number Patterns in Maths
1. What are patterns in mathematics?
A pattern in mathematics is a repeated arrangement of numbers, shapes, or other mathematical objects that follow a specific rule or a set of rules. For instance, the sequence 2, 4, 6, 8... is a pattern where the underlying rule is to add 2 to the previous number to get the next one.
2. What are the common types of number patterns in mathematics?
The most common types of number patterns taught in the CBSE syllabus include:
Arithmetic Patterns: Where a constant number is added or subtracted each time (e.g., 5, 10, 15, 20...).
Geometric Patterns: Where the previous number is multiplied or divided by the same value each time (e.g., 3, 9, 27, 81...).
Fibonacci Sequence: Where each number is the sum of the two preceding ones (e.g., 0, 1, 1, 2, 3, 5...).
Growing and Shrinking Patterns: Patterns that increase or decrease in a predictable way, which can involve shapes or numbers.
3. How can you identify the rule in a simple number pattern?
To identify the rule in a pattern, first observe the relationship between consecutive numbers. If the difference between them is constant, it is an arithmetic pattern (rule is addition/subtraction). If the ratio is constant, it is a geometric pattern (rule is multiplication/division). For example, in the pattern 40, 35, 30, 25..., the difference is always -5, so the rule is "subtract 5".
4. What is the difference between an arithmetic pattern and a geometric pattern?
The key difference lies in the operation used to generate the sequence. An arithmetic pattern is formed by adding or subtracting a fixed number (the common difference) to get the next term. In contrast, a geometric pattern is formed by multiplying or dividing by a fixed number (the common ratio) to get the next term.
5. Can patterns be made with shapes and not just numbers?
Yes, absolutely. Patterns made with shapes, colours, or objects are often called visual or geometric patterns. They follow a rule based on a sequence of changes in attributes like shape, size, colour, or orientation. For example, a repeating pattern of Red Triangle, Blue Square, Red Triangle, Blue Square... is a visual pattern that helps develop spatial reasoning skills.
6. What is the importance of the Fibonacci pattern in nature?
The Fibonacci sequence is important because it appears frequently in natural structures, demonstrating how mathematics governs the world around us. This pattern can be observed in the arrangement of petals on a flower, the spiral growth of pinecones and seashells, the branching of trees, and even the shape of spiral galaxies. It represents a highly efficient model for growth and packing in nature.
7. Where else, besides nature, do we see mathematical patterns in daily life?
We constantly interact with mathematical patterns in our daily routines. Some common examples include:
Music: Melodies and rhythms are built on repeating sequences of notes and beats.
Architecture: The design of buildings often uses repeating shapes for windows, floor tiles, and support structures.
Calendars: The days of the week and months of the year follow a predictable, repeating cycle.
Traffic Signals: The sequence of red, yellow, and green lights is a simple, predictable pattern.
8. Why is learning to recognise patterns important for a student's mathematical development?
Recognising patterns is a foundational skill in mathematics because it develops logical reasoning and critical thinking. This ability allows students to make predictions, understand relationships between numbers, and identify rules. Mastering patterns at an early stage is crucial as it forms the basis for understanding more complex topics like algebra and functions in higher classes.
