




Linear Equation in One Variable - Introduction
The general form for the linear equation is jx + k = 0. In this equation, j and k are integers and the solution of x can only be 1. For instance, 8x + 7 = 10 is the equation, which is linear, and it only has a single variable in it. The only type of solution for this equation is x = ⅜ . In the case of the linear equation with two variables, there will be two solutions as it has got two variables. The formula list for linear equations reduces the time spent in searching for these formulas. The list provides great help to the students in ensuring that they don’t miss any of the formula while preparing for the board exams or the competitive exams.
In the worksheet, we get to work on the linear equations of one variable definition, real life instances of the linear equations in one variable, formulas, solution, word problems, and the worksheet.
What is a Linear Equation in One Variable?
When we have the variable of one of a max of one order in the equation, then it is called the linear equation in one variable. The linear equation is generally expressed as jx + k = 0. In this equation j and k are the two integers and the solution for x can only be one. For instance, 5x + 6 = 10 is the equation which is linear and it only has a single variable within it. The only solution that you get for this equation is x = ⅘. Here are a few more samples of these equations: 13x - 92 = 139, 27x - 9 = 81.
A linear equation is an equation in which the highest degree of the equation is one. To put it simply, it is an equation that contains no exponents. A linear equation with one variable's standard form is
ax+b = 0 wherein x is the variable, a is the coefficient and b is the constant.
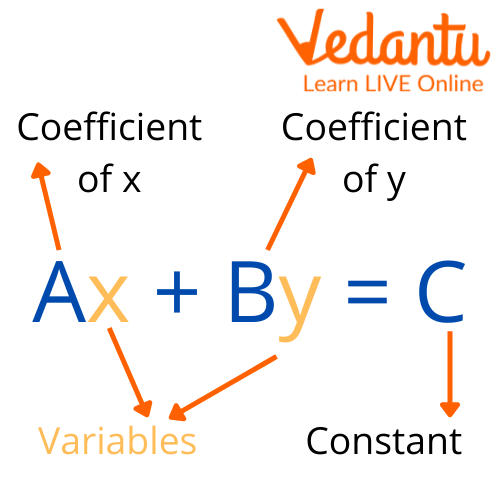
Standard Form of Linear Equation
Different Types of The Linear Equations
There are fundamentally three types of linear equations. They are as follows:
linear equations in one variable
linear equations in two variables
linear equations in three variables.
Linear Equation Formula
The standard form of the linear equation is generally expressed in the following form: jx + k =0. In this equation, j and k are the two integers, the solution for x can only be one, and the value for j and k can never be zero.
The Process of Solving the Linear Equations in Variable
When you are solving an equation that only has one solution, then you should follow these steps:
First find the LCM. If there are any fractions that exist, then you should clear them.
The next step involves simplification of both sides of the equation as it happens.
Next, you should isolate the variable on one side.
Finally you need to verify the result that you have obtained.
Things to Remember When Solving Linear Equation Exercises
To simplify an equation we can multiply or divide the equation on both sides of the equation
For example, let's take 3x+6= 18
If the same number is added, subtracted, multiplied, or divided into both sides of a linear equation, the solution remains unchanged.
When a number is taken from one side of the equal sign to the other, the sign associated with it (-, +, x, ÷) is changed to its opposite sign.
If the sign (- or + ) is equal on both sides, they can be canceled out.
Example : -2x = -4
= 2x = 4 (the minus side on both sides is canceled)
Knowing the rules of adding, subtracting, dividing, and multiplying integers is very useful in solving equations.
Solved Examples
Q 1: Solve the following equations:
Ans:
So, first of all we are going to add the above equation. For that, we have to take the LCM of the denominator, and here it is 60.
Now taking the denominator of LHS to the RHS
Now solving the bracket by expanding the multiplication.
Sequencing the like term together to do the further calculation.
After solving, we get
Taking the constant term to the RHS
On further solving, we get
Solving for the value of x, we get
Q 2: Solve the following equations:
Ans:
First of all, we will expand the bracket by doing the multiplication.
Now adding the constant term together in LHS, we get
Keepimg the variable at one side and constant on the another side, so we get
Now on solving, we get
Since both side have negative sign, so it will cancel out each other, our equation will become;
Solving for the value of x, we get
Q 3: Solve the following equations:
2x – 3 = 7
Ans: Step 1: Add 3 to both sides.
The next step is dividing both sides by 2.
Therefore x = 5
Q 4: Solve the following equations:
2x – 3 = x + 2
Ans: 2x = x + 2 + 3
Therefore x = 5
Q 5: Solve the following equations
72x – 7 = 28x – 5
Ans: Taking variable at one side and constant at other side, we get
72x – 28x = 7 – 5
Solving the LHS and RHS
Solving for the value of x, we get
Therefore x =
Linear Equation Practice Problems
Q 1: Solve the following equation
3x - 2 = 4
Ans: 2
Q 2: Solve the following equation
Ans:
Q 3: Solve the following equation
17x - 5 = -
Ans:
Q 4: Solve the following equation
49x + 3 = 10
Ans:
Summary
A linear equation, which can be written as the combination of variables (or unknowns) and the coefficients, which are frequently real values and it has equal sign. The coefficients, which can be any expressions as long as they don't contain any of the variables, can be thought of as the equation's parameters.
The coefficients must not all be 0 in order for the equation to have any sense. An alternative method for creating a linear equation is to equalize a linear polynomial over a field, from which the coefficients are drawn, to zero. The numbers that, when used to replace the unknowns in such an equation, result in equality, are the solutions.
FAQs on Linear Equations in One Variable
1. What is Linear Equation Definition of One Variable?
When you have a variable of a maximum of one order in an equation, then it is known as a linear equation in one variable. The linear equation is usually expressed in the form of jx + k = 0. Here j and k are two integers and the solution of x can be only one. For Example, 5x + 6 = 10 is an equation that is linear and has only a single variable in it. The only solution to this equation would be x = ⅘.
2. How to Solve Linear Equations?
When you have to solve an equation that has always only one solution, then the steps given below are followed.
Step 1: Find the LCM. In case any fractions exist, clear them.
Step 2: In this step simplification of both the sides of the equation happens.
Step 3: Here, you will be isolating the variable on one side.
Step 4: You will verify the obtained result.
3. How can I define the linear equation of one variable?
When we have the variable of max one order in the equation, then it is called the linear equation in one variable. Usually, the linear equation is expressed or denoted by jx + k = 0. In this equation, j and k represent the integers and there can only be one solution for x. For instance, in the linear equation 5x + 6 = 10, there is a single variable in it. The only solution that would be possible for this equation is x = ⅘.
4. What are the steps involved in solving the linear equations?
When you have to solve a linear equation which only has one solution then you must follow these steps for solving the problems.
Step 1: First and foremost, you need to find LCM (least common multiple). If there are any fractions present, then you must clear them.
Step 2: Next you must simplify both sides of the equation.
Step 3: Now the variable should be isolated on one side.
Step 4: Finally the obtained result should be verified.
5. What are some of the important formulas associated with algebraic function in linear equations?
There are various important algebraic formulas that are relevant for class 11. Some of these formulas include Commutative property of addition, Distributive property, Commutative property of multiplication, Associative property of multiplication, Associative property of addition, Multiplicative identity property, Additive identity property, Additive inverse property, Zero property of multiplication, and Multiplicative inverse property. Learning each of these formulae for algebraic function is important and you study about these formulae by referring to the Vedanut notes.
6. What are the features of the Vedantu notes on “Linear equations in one variable”?
The Vedantu notes on “Linear equations in one variable” provide an excellent guide and study reference to the students on the topic. The notes include detailed explanation, documentation of various sub-topics, depiction of important formulae, and solved problems. These comprehensive features presented in a structured way and embedded in the content help the students to understand the complex concepts easily. This helps in the preparation for the exams.
7. How can I refer to the Vedantu notes on “Linear equations in one variable”?
If you want to refer to the Vedantu notes on “Linear equations in one variable” then it is available for free download on the website and the app. These Notes are offered in the PDF file format. For downloading the file to your device for offline use, you need to navigate to the specific link and then click on the “Download PDF” option. Now the file containing the notes will be downloaded at your specified location.











