Courses

Courses for Kids

Free study material

Store

Talk to our experts

1800-120-456-456

Maths

Numbers Divisible by 4: Definition, Rules, and Examples

Numbers Divisible by 4: Definition, Rules, and Examples

Q: 1. What are the numbers divisible by 4?

A number is divisible by 4 if, when divided by 4, the result is a whole number with no remainder. In other words, any integer $n$ such that $4 \mid n$ (4 divides $n$ evenly) is considered divisible by 4. Typically, All multiples of 4 (e.g., 4, 8, 12, 16, 20, ...)A number whose last two digits form a number that is itself divisible by 4are numbers divisible by 4. For example, 124 is divisible by 4 since 24 is divisible by 4.

Q: 2. Is 61380 divisible by 4?

To determine if 61380 is divisible by 4, examine the last two digits (80). Since 80 is divisible by 4 ($80 \div 4 = 20$), the entire number 61380 is also divisible by 4. Therefore, 61380 is divisible by 4.

Q: 3. How many numbers are divisible by 4 from 1 to 140?

To find how many numbers from 1 to 140 are divisible by 4, we solve for the number of multiples of 4 in this range: The sequence of numbers divisible by 4 is: 4, 8, 12, ..., 140This is an arithmetic progression with first term $a_1 = 4$, common difference $d = 4$, and last term $a_n = 140$.Number of terms $n$ is given by: $a_n = a_1 + (n-1)d \Rightarrow 140 = 4 + (n-1)\times4 \Rightarrow n = (140-4)/4 + 1 = 35$So, there are 35 numbers between 1 and 140 that are divisible by 4.

Q: 4. What is the divisibility rule of 2, 3, 4, 5, 6, 7, 8, 9, 10?

Divisibility rules help determine if a number is divisible by another without full division. Summary:2: If the last digit is even (0, 2, 4, 6, 8).3: If the sum of digits is a multiple of 3.4: If the last two digits form a number divisible by 4.5: If the last digit is 0 or 5.6: If the number is divisible by both 2 and 3.7: Double the last digit, subtract it from the rest. If result is 0 or multiple of 7, it's divisible by 7.8: If the last three digits form a number divisible by 8.9: If the sum of digits is a multiple of 9.10: If the last digit is 0.

Q: 5. What is the easiest way to check if a number is divisible by 4?

The quickest way to check if a number is divisible by 4 is to examine its last two digits. If these two digits, as a standalone number, are divisible by 4, then the whole number is also divisible by 4. For example, in the number 1,236, the last two digits (36) are divisible by 4 ($36 \div 4 = 9$), so 1,236 is divisible by 4.

Q: 6. What is the general formula for finding the nth number divisible by 4?

To find the nth number divisible by 4, use the formula: \[ a_n = 4 \times n \]Here, $a_n$ is the nth multiple of 4, and $n$ is the position in the sequence. For example, the 10th number divisible by 4 is $4 \times 10 = 40$.

Q: 7. Why do numbers divisible by 4 also qualify as divisible by 2?

Any number divisible by 4 can be expressed as $4k$ for some integer $k$. Because 4 is itself a multiple of 2, $4k = 2 \times 2k$, so all multiples of 4 are also multiples of 2. Thus, divisibility by 4 always implies divisibility by 2, but not vice versa.

Q: 8. What is the smallest 3-digit number divisible by 4?

The smallest three-digit number is 100. Since $100 \div 4 = 25$, and it yields no remainder, 100 is the smallest three-digit number divisible by 4.

Q: 9. How can students use Vedantu resources to master divisibility concepts?

Students can use Vedantu's interactive lessons, practice worksheets, and live doubt-solving sessions to master divisibility rules, including numbers divisible by 4. With experienced educators providing step-by-step explanations and tailored quizzes, understanding and applying divisibility concepts becomes easier and more engaging.

Q: 10. How is the divisibility rule for 4 different from that for 8?

The divisibility rule for 4 is based on the last two digits: if they are divisible by 4, so is the number. For 8, you must consider the last three digits—if these are divisible by 8, the entire number is divisible by 8. This distinction is important for quickly checking large numbers.

Reviewed by:

Rama Sharma

How to Check if a Number Is Divisible by 4 Easily

You must have heard the word “divisibility” in Maths. The meaning of divisibility is the capacity to be completely divided without remainder. Some divisibility rules must be followed to check this divisibility. Without doing actual division, how can we guess numbers divisible by 4 or not? In this article, you will be learning the divisibles of 4 or the divisibility rules of 4. This article will explore the divisibility rule of 4 with examples and how it can be used to test whether a number is divisible by 4.

Divisibility Rule of 4

What Do You Mean by Divisibility?

Divisibility is defined as the rule or way to determine whether a given large or small number is divisible by a given fixed number (divisor).

A number is divisible by another number if it can be divided by that other number equally or if doing so results in a whole number. For instance, because 6 divided by 3 gives 2 and 2 is a whole number, then 6 is divisible by 3 (we say "3 divides 6").

A set of broad guidelines known as the "divisibility rules" is frequently used to determine whether or not a number is evenly divisible by another number. For e.g., if we have to check whether the number 432 is divisible by 4 or not.

Given number = 432

Fix number or divisor = 4

In detail, we will learn in the next heading about the numbers divisible by 4.

So for some numbers, we have some divisibility rule to find out whether the number is divisible by a divisor or not.

Division Into 4 Parts

Divisibility Rule of 4

The divisibility rule of 4 is defined as the given number being divisible by 4 if the last two digit numbers of the given number are zeros or they are the multiples of 4 (4, 8,12,16,20,24,.....).

This rule helps students to find out if the given number is divisible by 4 or not.

Some of the whole numbers which are divided by 4 completely are 0,4,8,12,16. We all know a table of 4. Hence these multiples of 4 are completely divisible by 4.

How to Check Divisibility by 4?

In this trick, we have to follow two conditions to check the divisibility test of 4 given as follows:

Check whether the last two digits of a given number are zeros. If yes, then the given number will be divisible by 4. If no, then check for the second condition given below. Zeros should be in the ones and tens place.

For e.g., 700 is a number that has zeros at ones and tens places. Hence, we can say that 700 is divided by 4 without doing any calculation.

Check if the last two digits of a given number are exactly the multiple of 4 or come in the table of 4, then we can say that the given number is divisible by 4.

For e.g., 736 checks two digit numbers are bold and underlined.

36 comes in the table of 4, or it is a multiple of 4. Hence we can say that 736 is completely divided by 4.

Divisibility Rule of 4 with Example

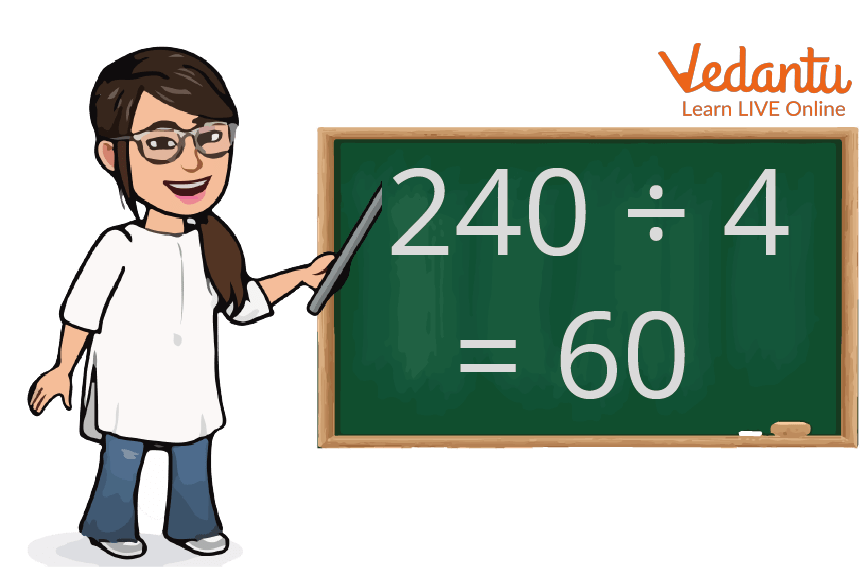
Some Examples

Here are some questions that show the numbers divisible by 4

1. Check whether the given numbers are divisible by 4 or not.

1700
6500

Ans:

1700

Given the number- 1700

The last two digit numbers are -00

Hence Divisibility Rule 1 is followed, and 1700 is divisible by 4.

1700 ÷ 4 = 425

6500

Given the number- 6500

The last two digit numbers are -00

Hence Divisibility Rule 1 is followed, and 6500 is divisible by 4.

6500 ÷ 4 = 1625

Practice Questions

Q 1. Which one of the following is completely divisible by 4? Tick the correct option.

Ans: 36

Q 2. Which one of the following is completely divisible by 4? Tick the correct option.

Ans: 840

Summary

Divisibility is defined as the rule or way to determine whether a given large or small number is divisible by a given fixed number (divisor). The divisibility rule of 4 is defined as the given number being divisible by 4 if the last two digit numbers of the given number are zeros or multiples of 4.

FAQs on Numbers Divisible by 4: Definition, Rules, and Examples

1. What are the numbers divisible by 4?

A number is divisible by 4 if, when divided by 4, the result is a whole number with no remainder. In other words, any integer $n$ such that $4 ∣ n$ (4 divides $n$ evenly) is considered divisible by 4. Typically,

All multiples of 4 (e.g., 4, 8, 12, 16, 20, ...)
A number whose last two digits form a number that is itself divisible by 4

are numbers divisible by 4. For example, 124 is divisible by 4 since 24 is divisible by 4.

2. Is 61380 divisible by 4?

To determine if 61380 is divisible by 4, examine the last two digits (80). Since 80 is divisible by 4 ( $80 \div 4 = 20$ ), the entire number 61380 is also divisible by 4. Therefore, 61380 is divisible by 4.

3. How many numbers are divisible by 4 from 1 to 140?

To find how many numbers from 1 to 140 are divisible by 4, we solve for the number of multiples of 4 in this range:

The sequence of numbers divisible by 4 is: 4, 8, 12, ..., 140
This is an arithmetic progression with first term $a_{1} = 4$ , common difference $d = 4$ , and last term $a_{n} = 140$ .
Number of terms $n$ is given by: $a_{n} = a_{1} + (n - 1) d \Rightarrow 140 = 4 + (n - 1) \times 4 \Rightarrow n = (140 - 4) / 4 + 1 = 35$

So, there are 35 numbers between 1 and 140 that are divisible by 4.

4. What is the divisibility rule of 2, 3, 4, 5, 6, 7, 8, 9, 10?

Divisibility rules help determine if a number is divisible by another without full division. Summary:

2: If the last digit is even (0, 2, 4, 6, 8).
3: If the sum of digits is a multiple of 3.
4: If the last two digits form a number divisible by 4.
5: If the last digit is 0 or 5.
6: If the number is divisible by both 2 and 3.
7: Double the last digit, subtract it from the rest. If result is 0 or multiple of 7, it's divisible by 7.
8: If the last three digits form a number divisible by 8.
9: If the sum of digits is a multiple of 9.
10: If the last digit is 0.

5. What is the easiest way to check if a number is divisible by 4?

The quickest way to check if a number is divisible by 4 is to examine its last two digits. If these two digits, as a standalone number, are divisible by 4, then the whole number is also divisible by 4. For example, in the number 1,236, the last two digits (36) are divisible by 4 ( $36 \div 4 = 9$ ), so 1,236 is divisible by 4.

6. What is the general formula for finding the nth number divisible by 4?

To find the nth number divisible by 4, use the formula:
$a_{n} = 4 \times n$
Here, $a_{n}$ is the nth multiple of 4, and $n$ is the position in the sequence. For example, the 10th number divisible by 4 is $4 \times 10 = 40$ .

7. Why do numbers divisible by 4 also qualify as divisible by 2?

Any number divisible by 4 can be expressed as $4 k$ for some integer $k$ . Because 4 is itself a multiple of 2, $4 k = 2 \times 2 k$ , so all multiples of 4 are also multiples of 2. Thus, divisibility by 4 always implies divisibility by 2, but not vice versa.

8. What is the smallest 3-digit number divisible by 4?

The smallest three-digit number is 100. Since $100 \div 4 = 25$ , and it yields no remainder, 100 is the smallest three-digit number divisible by 4.

9. How can students use Vedantu resources to master divisibility concepts?

Students can use Vedantu's interactive lessons, practice worksheets, and live doubt-solving sessions to master divisibility rules, including numbers divisible by 4. With experienced educators providing step-by-step explanations and tailored quizzes, understanding and applying divisibility concepts becomes easier and more engaging.

10. How is the divisibility rule for 4 different from that for 8?

The divisibility rule for 4 is based on the last two digits: if they are divisible by 4, so is the number. For 8, you must consider the last three digits—if these are divisible by 8, the entire number is divisible by 8. This distinction is important for quickly checking large numbers.