What are Patterns in Mathematics?

Mathematics is based on numbers. It entails the investigation of various patterns. There are many distinct kinds of patterns, including word patterns, logic patterns, image patterns and number patterns. In Mathematics, number patterns are quite prevalent. All number patterns are very interesting. This article will cover what a pattern is, as well as several patterns, including arithmetic patterns, geometric patterns and many instances with solutions.

Pattern in Mathematics

A recurring arrangement of numbers, forms, colours and other elements constitutes a pattern in Mathematics. When a group of numbers is arranged in a particular way, the arrangement is referred to as a pattern. Patterns can also be sometimes referred to as a series. The number of patterns can be limitless or finite. Several examples of numerical patterns include the following:

• The pattern of even numbers: 2, 4, 6, 8, 10, 1, 14, 16, 18...

• The pattern of odd numbers: 3, 5, 7, 9, 11, 13, 15,...

• The pattern of the Fibonacci series: 1, 1, 2, 3, 5, 8, 13, 21, and so forth.

Number Patterns

Number patterns are a collection of numbers that are arranged in a particular order. There are many distinct kinds of number patterns, including geometric, Fibonacci and algebraic or arithmetic patterns. Let's now examine the three distinct patterns that are there.

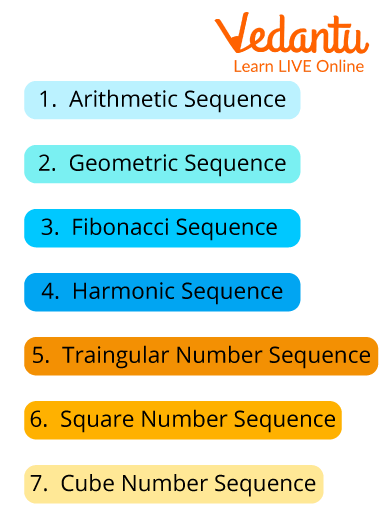
Different Types of Math Patterns

Pattern Rules in Mathematics

We need to be aware of some rules in order to create patterns. Understanding the nature of the sequence and the distinction between the two succeeding phrases is necessary in order to grasp the rule for any pattern.

• Identifying Missing Term: Take a look at the patterns 1, 4, 9, 16 and 25. It is obvious from this pattern that each number is the square of the number corresponding to its location.

Example: What will be the value of x in the pattern: 1, 3, 5, x?

The answer will be 7 as there are all odd numbers in the sequence.

• Difference Rule: In some cases, it is simple to determine the difference between two phrases that follow one another.

Example: 1, 5, 9, 13, 17, 21, and so on.

Solved Examples of Number Pattern Problems

1. Find the values of A and B in the following pattern.

15, 22, 29, 36, 43, C, 57, 64, 71, 78, 85, D.

Solution: Following is the given order: 15, 22, 29, 36, 43, C, 57, 64, 71, 78, 85, D.

In this case, the number is rising by \[ + 7\].

As the difference between the two numbers is 7 in this series, C will be equal to 50 by adding 43 and 7.

D's previous digit is 85. D will therefore be \[85 + 7 = 92\].

C, therefore, has a value of 50, and D has a value of 92.

2. Find the rule for the following hard number pattern: 81, 27, and 9.

Solution: It is a falling pattern, which is the first thing to notice.

Therefore, let's begin with the lowest number 9.

As can be seen, \[9 \times 3 = 27\].

Let's check the following number to see if this trend holds true.

\[27 \times 3\] Equals 81.

As a result, if we comprehend the series in the order provided, we can see that the pattern rule used in this situation is "Divide by 3".

This indicates that 81 divided by 3 gives us 27, and 27 divided by 3 gives us 9.

3. What are the next 2, 7, 8, 3, 12, and 9?

Solution: We have to find the pattern of numbers following the sequence:

2, 7, 8, 3, 12, 9

Assume that the sequence's final three terms are x, y, and z, making it 2, 7, 8, 3, 12, and x, y, z. The sequence should be divided into three groups. Let the first group consist of the numbers 2, 7, 8, 3, 12, 9, and the third group consists of [x, y, z].

The first element of the first and second group differs by 1, making \[3 - 2 = 1\], the second element of the first and second group differs by 5, making \[12 - 7 = 5\], and the third element of the first and second group differs by 1, making \[9 - 8 = 1\]. The second and third groups' components follow the same pattern: \[{\rm{x}} - 3 = 1\], \[{\rm{y}} - 12 = 5\], and \[z - 9 = 1\]; \[{\rm{x}} = 4\], \[{\rm{y}} = 17\], and \[{\rm{z}} = 10\].

Thus, 4 will be the following number.

Conclusion

Numbers are the foundation of Mathematics. It describes looking into various patterns. Every number pattern is fascinating. An arrangement of numbers is referred to as a pattern when it is done in a certain way. Children can practise Maths pattern problems with the help of worksheets available online.