




Introduction
In mathematics refers to a specific pattern in which we may draw a centre dividing line on a form or object and it offers two perfectly identical sides termed symmetry, and the shape or thing is referred to as symmetrical when this occurs. Examples of symmetrical figures are butterflies, and honeycombs made by bees. There are different types of symmetry, one of them being point symmetry. Let us now learn about it.
What Is Point Symmetry?
Point symmetry occurs when every part has a mirror portion. It is the same distance as the centre point but in the opposite direction. It is sometimes referred to as "Order 2 rotational symmetry." However, the two conditions listed below can be used to see if a shape possesses symmetry around a point.
Every portion of the defined form must be the same distance from the centre as the others.
The part of the form and its equivalent part must be in opposing directions.
What Is Line Of Symmetry?
The line of symmetry is an imaginary line that divides a form into two precise or identical halves. This line of symmetry in math shows the symmetry in form along only one axis. Many forms can have many lines of symmetry because they can be split into half by more than one line. A location within an object or figure through which any straight line passes, as well as two points on the figure's edge at the same distance from the centre but on opposing sides, is the centre of symmetry.
The following are the attributes of the line of symmetry:
It always travels through the shape's centre.
The halves formed by creating the symmetry line reflect each other. As a result, this sort of symmetry is also known as reflection symmetry.
Because the halves formed by drawing the line of symmetry math are mirror copies of each other, the symmetry is also known as mirror symmetry.
Point Symmetry Is Seen In Alphabet
When you draw a line across the point of symmetry, it will intersect the figure such that one side of the point would be at the same distance from the point. Point symmetry exists when every piece of an item has an equal part and can be seen in the English alphabet. The alphabet O has the centre point, and the equivalent parts are oriented in opposite directions. The uppercase letters H, I, N, O, X and Z all have point symmetry; when you draw a line from the middle of H, I, O and X you will get both upper and lower parts of the same length. The letters H, I, O, and X all have point and line symmetry.
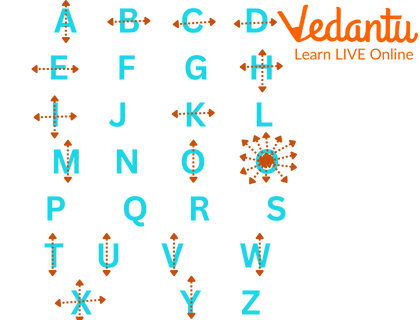
Centre of Symmetry Examples For Alphabets
Point Symmetry In Shapes
If two equal forms are made by putting a point on an object or shape, but they face different directions, the object or shape shows point symmetry. Or in simple words, we can say that the point of origin should be equally distant from the two opposite sides of a shape. Squares and rectangles, for example, have a point of symmetry. We can restore the original form by rotating the shapes square and rectangle 180 degrees.
Conclusion
In conclusion, we can say that every object may or may not be symmetrical depending upon the line of symmetry that passes through it. An object has a centre of symmetry if an imaginary line can be stretched from any point on its surface to its centre and a corresponding point exists along the line equidistant from the centre.
Sample Questions
1. Which alphabet has a point of symmetry?
a. Q
b. O
c. G
d. L
Ans. O
Explanation: A point of symmetry is a point of origin through which a line of symmetry passes. O can be divided into two equal-looking halves making it an alphabet not having a line of symmetry with two parts being placed equally distant from each other.
2. Which shapes do not have a point of symmetry?
a. Equilateral Triangle
b. Circle
c. Square
d. None of the above
Ans. None of the above
Explanation: All the shapes have points of symmetry as they have two opposite parts that are equally placed from the point of origin.
3. A line of symmetry divides the object into two or more parts.
a. True
b. False
Ans. False
Explanation: A line of symmetry divides the object into two equal halves which when placed over one another cover themselves fully.
FAQs on Point Symmetry
1. Define point symmetry?
Point symmetry is the situation in which every portion is the same distance from a certain centre point.
2. How do you determine whether a figure has point symmetry?
To understand if the given figure possesses point symmetry, we must first understand the following criteria:
The point symmetry requirements that a form or figure must meet, i.e., each piece must have a matching part.
The distance from the central point must be equal.
The direction, however, should be reversed.
3. Does the English alphabet have a point of symmetry?
An item is said to have point symmetry if every section of it has a corresponding part. Many letters in the English alphabet have point symmetry. O is the centre point, and the equivalent parts are pointed in opposite directions.
4. Does point symmetry fall within the group of symmetry?
An item or shape has point symmetry if two equal forms are generated by adding a point to the object or shape, but they face different directions. It's as if you divided the thing in half such that all sides are the same but facing opposite directions.
5. What is meant by a symmetrical shape?
If a line can be traced across a 2D form and either side is a mirror of the other, it is said to be symmetrical. A square, for example, has a symmetrical form.











