




What Do You Mean by Remainder in Maths?
According to the Merriam-Webster Dictionary, the remainder is, “a remaining group, part, or trace/ the number left after a subtraction/ the final undivided part after the division that is less or of lower degree than the divisor”. In simple words, the term "remainder" refers to the part that remains after the division process has been completed. If we divide 8 pencils equally among 7 children, we are left with 1 pencil. In this example, the remaining 1 pencil represents the remainder. Also, if we divide 33 by 2, the quotient is 16 and the remainder is 1. The divisor is always greater than the remainder.
Understanding Remainder in Maths in Detail
The remainder refers to the portion of the dividend that the divisor cannot divide fairly. You may end up with a fraction of the dividend left over after dividing whole numbers to determine the quotient; this is the remainder. It is a decimal or a fraction that represents a portion of the dividend. Let us consider one example.
Suppose you have 23 chocolates and you want to divide it equally among 4 of your friends. So, how many will each friend get? How many chocolates will remain after distributing the chocolates equally? The answer is: Each friend will get 5 chocolates, so 20 chocolates can be distributed equally among 4 friends and 3 chocolates will remain which cannot be distributed. So, here, 23 is the dividend, 4 is the divisor, 5 is the quotient and 3 is the remainder.
Remainder Formula
As we know,
Dividend = Divisor × Quotient + Remainder
Accordingly, the remainder formula is given as:
In the above remainder formula,
The dividend is the number or value that is being divided.
The divisor is the number that divides another number.
The quotient is the result that is obtained after the division of two numbers.
The remainder is the value that is left after the division.
How to Find the Remainder After a Division?
We can't always show in pictures how we divide the number of things equally among the groups to find the remainder. Instead, we can use the long division method to find the remainder. Here is a memory trick to always remember the steps of long division:
Does McDonald’s Sell Cheeseburgers Daily?
Step I: Does - Divide the dividend by the divisor.
Step II: McDonald’s - Multiply the partial quotient times the divisor.
Step III: Sell - Subtract the product from the first digits of the dividend.
Step IV: Cheese - Compare the difference with the divisor; the difference must be smaller.
Step V: Burger’s Daily - Bring down the next digit of your dividend and begin again.
Let us understand in simple terms. Consider the following image:
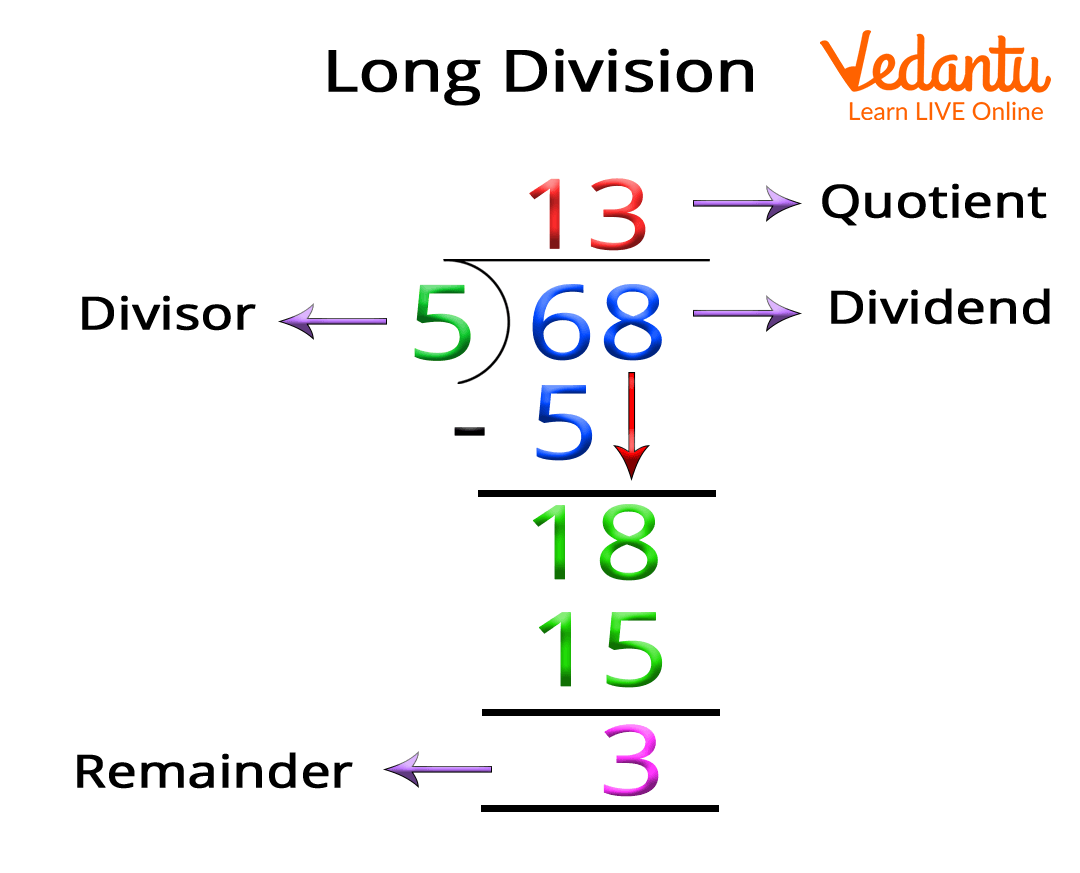
Here, 68 is the dividend, 5 is the divisor, 13 is the quotient and 3 is the remainder.
At first, 6 is divided by 5 and we got the first digit of the quotient 1. We multiplied 1 and 5 and wrote the result below 5. Then, we subtracted 5 (5 x 1 = 5) from 6 and got 1. Then, we brought down 8 and got the number 18. In the next step, we divided 18 with 5 and got the second digit of the quotient i,e 3. So, after multiplying, the number came to 15. On subtracting 15 from 18, we got 3. So, 3 is the remainder.
How to Write Remainders?
There are several ways to express the remainder of a division problem. The remainder can be either a whole number or a faction. One way to write the remainder is to separate the quotient and the remainder with a "R." 7/2 = Q=3 and R=1 is the formula for dividing 7 by 2. In this case, Q=3 is a quotient, and R=1 is a remainder.
Another way to represent the remainder is as a component of a mixed fraction. 7/2 = 3 12 is the formula for dividing 7 by 2.
Properties of Remainders
The following are the properties of the remainder:
The divisor is always less than the remainder. The division is wrong if the remainder is either more than or equal to the divisor.
When one number (divisor) entirely divides the other number (dividend), the remainder is 0.
The remainder can be more than, equal to, or less than the quotient.
Remainder Examples
Here are a few examples of remainders that will help you understand the term remainder in Maths in a better way.
1. A teacher had 315 chocolates. She divides all chocolate evenly among 30 students. Find
How many chocolates did the teacher give to each of the students?
How many chocolates are left with the teacher after distributing them among the student?
Solution:
Total number of students in class = 30
Total number of chocolates teacher has = 315
Now, we will divide 315 by 30
(Image will be uploaded soon)
i) The quotient value of the above division represents the number of chocolate each student gets is 10.
Therefore,
Chocolates each students gets = 10
ii) The remainder value of the above division represents the number of chocolate left with the teacher.
Therefore,
Chocolates left with the teacher after distribution = 15
2. What is the remainder when 53 is divided by 8?
Solution: To find the value of the remainder, when 53 is divided by 8, we consider the multiples of 8.
8 × 1 = 8
8 × 2 = 16
8 × 3 = 24
8 × 4 = 32
8 × 5 = 40
8 × 6 = 48
8 × 7 = 56
As 8 × 6 = 48 and 8 × 7 = 56, only six 8’s can go into 53. Then the value left over is 53 - 48 = 5. Hence, the value of the remainder when 53 is divided by 8 is 5.
Do You Know?
FAQs on Remainder - Explanation, Formula, Method of Finding Remainder and Examples
Q1. What are the Different Properties of the Remainder in Maths?
Answer: The different properties of the remainder in Maths are as follows:
The value of the remainder is always greater than the divisor. If the value of the remainder is less than or equal to the divisor, it implies that division is incomplete.
The value of the remainder can be either lesser, equals to, or greater than the value of the quotient.
If a number (divisor) completely divides another number ( dividend), the value of the remainder is 0.
Q2. Can the Number 0 can be a Remainder?
Answer: Yes, the number 0 can be a remainder when the divisor divides the dividend completely. For example, the value of the remainder when 30 is divided by 6 is 0.
Q3. What is the Remainder of 19 Divided by 6?
Answer: 19 divided 6 is 3 R 1
Where 3 is the quotient and 1 is the remainder.
Hence, the remainder of 19 divided by 6 is 1.
Q4. What is an Example of the Remainder?
Answer: When 29 chocolates are distributed equally among 9 children, each child gets 3 chocolates and 2 chocolates are left undistributed. Here, 2 is the remainder.
5. What is the formula of the remainder?
The basic formula is:
Dividend = Divisor x Quotient + Remainder
From the above formula, we can derive the following formula:
Remainder = Dividend – (Divisor x Quotient)
6. What is a remainder?
Remainder is a subset of division. We get it as a left-over digit when we divide. We get a remainder as a result of an incomplete division after certain steps. It is what remains after dividing a few things into groups of an equal number of things.






































