




An Introduction to Rounding off Significant Figures
Significant figures are used to establish the number, which is displayed as digits. These digits represent numbers in a meaningful way. In addition to figures, the term significant digits is frequently used. We can determine the number of significant digits by counting all of the values beginning with the first non-zero digit on the left. 12.45, for example, has four significant digits. In this article, you will learn more about Significant figures. Let’s start with its proper definition.
Definition: What are Significant Figures?
The significant figures of a given number are the digits that convey the meaning according to their accuracy. 4.0123, for example, has four significant digits. These significant figures add precision to the numbers. Significant digits are another term for them.
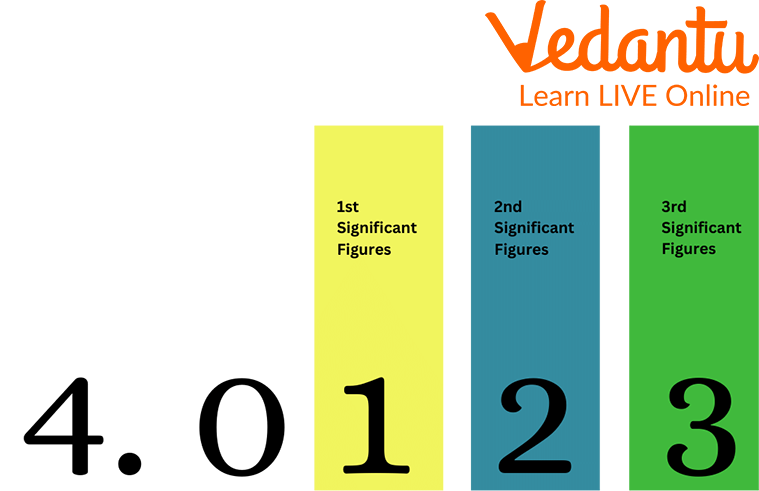
Significant Figures
What Do Significant Figures Mean?
Significant figures in mathematics define the number of significant single digits that contribute to the degree of accuracy of a given number.
Rules for Significant Figures
There are a few rules for significant figures given below:
Non-zero digits are all significant.
All zeros between any two non-zero digits are significant. For example, the number 108.0097 has seven significant digits.
All zeros to the right of a decimal point, as well as those to the left of a non-zero digit, are never significant. 0.00798, for example, had three significant digits.
All zeros to the right of a decimal point are significant if they are not followed by a non-zero digit. 20.00, for example, has four significant digits.
All of the zeros after the decimal point to the right of the last non-zero digit are significant. 0.0079800, for example, has five significant digits.
If the zeros to the right of the last non-zero digit are from a measurement, they are all significant. 1090 m, for example, has four significant digits.
Rounding Significant Figures
The method of rounding to a significant figure is often used as it can be applied to any kind of number, regardless of how big or small it is.
To round to a significant figure:
Look at the first non-zero digit if rounding to one significant figure.
Look at the digit after the first non-zero digit if rounding to two significant figures.
Draw a vertical line after the place value digit that is required.
Look at the next digit.
If it's 5 or more, increase the previous digit by one.
If it's 4 or less, keep the previous digit the same.
Fill any spaces to the right of the line with zeros, stopping at the decimal point if there is one.
Example: Round 53,879 to 1 significant figure, then 2 significant figures.
Ans: 5|3879 to 1 significant figure is 50,000
53|879 to 2 significant figures is 54,000
Notice that the number of significant figures in the question is the maximum number of non-zero digits in your answer.
Rules for Finding the Number of Significant Figures:
All non-zero numbers are always significant (1, 2, 3, 4, 5).
For example; 13245 has five significant figures.
For example; 25.14 has four significant figures.
All zeros in the interval between non-zero numbers are always significant.
For example; 204.003 has six significant figures.
51.02010 has seven significant figures.
In a decimal number between 0 and 1, all zeros to the right of the decimal point should be considered but to the left of a non-zero number are ignored.
For example; 0.00247 has only three significant figures.
0.002030 has four significant figures.
When a decimal is rounded to a specified number of decimal places, all of the final zeros in the decimal are removed.
Rounding off Decimals to the Required Number of Significant Figures:
Correctly rounding off the number to three significant figures
(1) 53.214 → It contains five significant figures.
We needed to round it off to one place after the decimal to get it to three significant digits.
As a result, 53.214 = 53.2, rounded to three significant figures.
The hundredth digit is 1, which is less than 5. As a result, the digit in the tenth place remains 2 while the digits 1 and 4 vanish.
(2) It has 5 significant figures (4.3062). To get to three significant figures, we round to the second place after the decimal point.
So, 4.3062 = 4.31 is correct to three decimal places.
(3) 30.002 → It contains five significant figures. To get to three significant figures, we needed to round it up to one decimal place after the decimal point.
To three significant figures, 30.002 = 30.0.
(4) 0.0001378 → It contains four significant figures. To round it to three significant figures, we must round it to six decimal places after the decimal point 0.0001378 = 0.000138 is correct to three significant figures.
(5) 0.0001366 → It contains four significant figures. To get to three significant figures, we need to round it to six decimal places after the decimal point.
0.0001366 = 0.000137 is the correct answer to three significant figures.
Solved Examples on Significant Figures
Examples to Rounding off to a Specified Unit
Q 1: Identify the number of significant digits/figures in the following given numbers.
45, 0.046, 7.4220, 5002, 3800
Ans:
Q 2: Give the number of significant figures in each measurement.
36.7 m
0.006606 s
2,002 kg
306,490,000 people
Ans:
1. This measurement has three significant numbers since all nonzero digits are significant.
2. The first three zeros are insignificant, but the zero between the sixes is, hence this number has four significant figures.
3. This measurement includes four significant figures because the two zeros between the two are significant.
4. The four trailing zeros in the number aren’t significant, but the other five are, making this a five-figure number.
Solved Examples to Rounding off to a Specific Unit
Q 1. The result of an experimental calculation corrected up to seven significant figures is 7.363573. Round it off to six, five, four, three and two significant figures.
Ans: The given quantity P = 7.363573
When rounding off to 6 significant figures, P6 = 7.36357 ( ∵ the dropped digit is less than 5, 6th significant figure remains the same).
When rounding off to 5 significant figures, P5 = 7.3636 (5th significant figure is added by 1, as the dropped digit is greater than 5).
Similarly, When rounding off to 4 significant figures, P4 = 7.364
When rounding off to 3 significant figures, P3 = 7.36
When rounding off to 2 significant figures, P2= 7.4
Q 2: Round off 18.066 cm to the nearest mm.
Ans: Since, 1 mm = 0.1 cm, so to round it off to the nearest mm, we have to round it off to one decimal place.
18.066 cm = 18.1 cm rounded off to the nearest mm or 181 mm.
Q 3: Round off 53.4278 m to the nearest cm.
Ans: Since, 1 cm = 0.01 m, we round off 53.4278 m to the nearest cm. We have to round it off to two places of decimal.
53.427 m = 53.43 m rounded off to the nearest cm or 5343 cm
Q 4: Rounding to the correct number of significant figures exercises with a 5 as the first non-significant figure:
Round 4.7475 to 4 significant figures
Round 4.7465 to 4 significant figures
Ans:
4.7475 becomes 4.748 because the first non-significant digit is 5 and the last significant figure is rounded up to 6.
4.7465 becomes 4.746 because the first non-significant figure is 5 and the last significant figure is even.
Practice Questions
Q 1. Write 12.378162 correct to 4 significant digits.
Ans: 12.38
Q 2. Round off 53.4278 m to the nearest cm.
Ans: Since, 1 cm = 0.01 m, we round off 53.4278 m to the nearest cm. We have to round it off to two places of decimal.
Summary
In this article we have learnt that the correct use of significant figures requires nothing more than common sense in mathematical computation. As we have seen, the significant figures refers to the number of important single digits (0 to 9 inclusive) in the coefficient of expression in the scientific notation. Then we have learned about the rounding off numbers upto the significant figures. In the end of the article, we have included some solved examples and practice problems to have better experience on the topic.
FAQs on Significant Figure
1. How is rounding used in everyday life?
Rounding numbers makes them 'easier' to use or understand whilst also keeping the number close to its original value. Instead of using exact numbers, simpler values can be used. For example, 189.2 could be rounded to 189, 190 or 200, depending on the degree of accuracy required.
2. How do you find the significant number?
We can use the following three rules to determine how many significant figures are present in a number:
Digits that are not zero are always important.
Any zeros in the range of two significant digits are noteworthy.
Only in the decimal section are a final zero or following zeros important.
3. What is the purpose of significant figures?
The number of digits in a value, often a measurement, that contributes to the degree of accuracy of the value is referred to as significant figures. At the first non-zero digit, we begin counting significant figures. Determine the number of significant figures for a set of numbers.











