Simplest Form of Fraction

Simplifying a fraction means reducing a fraction to its simplest form. A fraction is in its simplest form if its numerator and denominator have no common factors other than 1. An important step in solving fraction problems is reducing them to the simplest form. Though we simplify them, the fraction's value will remain unchanged. This means the simplified fraction and the actual fraction form a pair of equivalent fractions. In this article, you will learn about the simplest form of a fraction and how it can be converted.

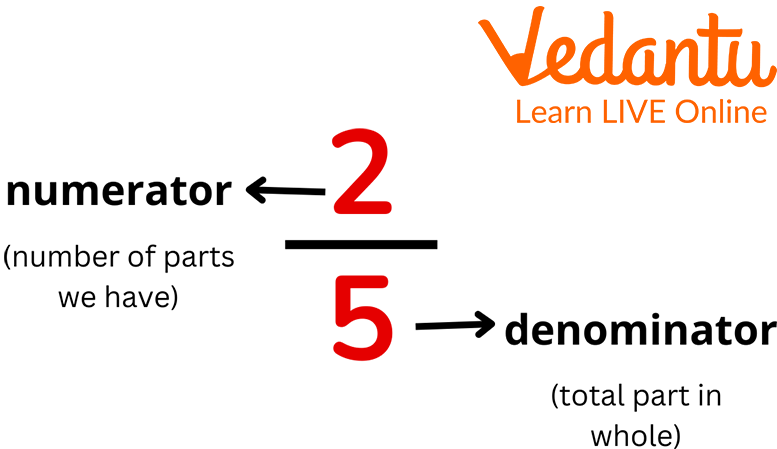
Numerator and Denominator

The Simplest Form of a Fraction

A fraction is in its simplest form if its numerator and denominator are co-prime or have no common factors except 1. The simplest form of a fraction is equivalent to the given fraction. For example, the fraction $\dfrac{3}{4}$ is in the simplest form because 3 and 4 have no common factor except 1.

Let's try simplifying the fraction $\dfrac{3}{6}$ step by step.

Simplification of Fraction

In the example, the simplified form is obtained by dividing the numerator and denominator by 3, the greatest number that divides both numbers exactly.

Fun Facts: The simplest form is the number's smallest possible equivalent fraction.

Simplification of Fractions in Step-by-Step Method

There are different ways to simplify fractions, and all these methods are explained below.

Method 1:

To understand how to simplify fractions, adhere to the detailed steps below.

Step 1: List the factors of the numerator and denominator numbers.

Step 2: Determine the common factors of the numerator and denominator.

Step 3: Divide the numerator and denominator by the common factors until they have no common factor except 1. The fraction so obtained is in the simplest form.

Consider the fraction, $\dfrac{8}{24}$ and follow the steps mentioned below to understand how to simplify the fraction $\dfrac{8}{24}$.

Step 1: Write the factors of the numerator and denominator.

The factors 8 and 24 are

Factors of 8: 1, 2, 4, and 8

Factors of 24: 1, 2, 3, 4, 6, 8, 12, and 24

Step 2: Determine the common factors of the numerator and denominator. The common factors of 8 and 24 are 1, 2, 4, and 8.

Step 3: Divide the numerator and denominator by the common factors until they have no common factor except 1. The fraction so obtained is in the simplest form. Let's start dividing by 2, then $\dfrac{8}{24} = \dfrac{\dfrac{8}{2}}{\dfrac{24}{2}} = \dfrac{4}{12}$.

We will divide by 2 until we can't go any further. So, we have

$\dfrac{\dfrac{4}{2}}{\dfrac{12}{2}}$

=$\dfrac{2}{6}$

$\dfrac{\dfrac{2}{2}}{\dfrac{6}{2}}$

= $\dfrac{1}{3}$

Conversion of a Fraction

A fraction can be converted into a decimal, a fraction can be converted into a percentage, improper fractions can be converted to mixed fractions, and vice versa.

$\dfrac{3}{5}, \dfrac{2}{3}, \dfrac{4}{7}, \dfrac{7}{11},$ etc., are the simplest forms of $\dfrac{6}{10}, \dfrac{8}{12}, \dfrac{20}{35},$ and $\dfrac{21}{33}$ respectively.

The simplest form examples are given below:

Percentage: 30%

Fraction: $\dfrac{3}{10}$

Decimal: 0.3

To go from a fraction to a percentage, we can convert to decimal first.

$\dfrac{3}{5} \rightarrow 0.6 \rightarrow 60 \%$

Solved Examples

Q 1. Find the simplest form of the fraction $\dfrac{11}{33}$.

Ans: The highest common factor of 11 and 33 is 11.

So, divide both the numerator and denominator by 11,

i.e $\dfrac{11}{33} = \dfrac{(11 \div 11)}{(33 \div 11)} = \dfrac{1}{3}$.

Therefore, the simplest form of $\dfrac{11}{33}$ is $\dfrac{1}{3}$.

Q 2. Convert $\dfrac{350}{175}$ into simplest form.

Ans: Here, $\dfrac{350}{175}=\dfrac{50}{25}=\dfrac{2}{1}$

Here, equivalent fractions of $\dfrac{350}{175}$ are $\dfrac{50}{25}$ and $\dfrac{2}{1}$. Also, these fractions have a numerator greater than the numerator, as you can see that $350>175,50>25$, and $2>1$. Besides this, the simplest form of the above fraction is $\dfrac{2}{1}$.

Q 2. Convert $\dfrac{200}{550}$ into simplest form.

Ans: Here, $\dfrac{200}{550}=\dfrac{20}{55}=\dfrac{4}{11}$

Here, equivalent fractions of $\dfrac{200}{550}$ are $\dfrac{20}{55}$ and $\dfrac{4}{11}$. Besides this, the simplest form of the above fraction is $\dfrac{4}{11}$.

Practice Problems

Q 1. Change $\dfrac{45}{60}$ to its simplest form.

Ans: $\dfrac{3}{4}$

Q 2. Change $\dfrac{15}{75}$ to its simplest form.

Ans: $\dfrac{1}{5}$

Summary

In this article, we have learned about the simplest fraction. It is just like simplifying a fraction. We have seen how we can convert the fraction into its simplest form by solving it. So to reduce the fractions, we just have to divide the numerator and denominator by their highest common factor. At the end of the article, we have added some solved examples and practice problem to have clear concepts regarding the simplest form.