How to Use the Speed Formula in Real-Life Maths Problems
A speed formula represents the distance covered at a specific rate. Distance travelled in a given amount of time is the measure of speed. By knowing the distance travelled by an object and the time it took, you can calculate its average speed. The speed formula and its applications will be discussed in this section.
The formula for speed is distance divided by time. The units of speed are meters per second (m/s) or kilometres per hour (km/hr).
Deriving the Formula of Speed
Like any other equation, the speed equation = distance /time can be rearranged.
There are three ways to rearrange the formula:
Distance/time = speed
Speed* time = distance
Distance/speed = time
Two variables are needed to compute one (speed, distance, time).
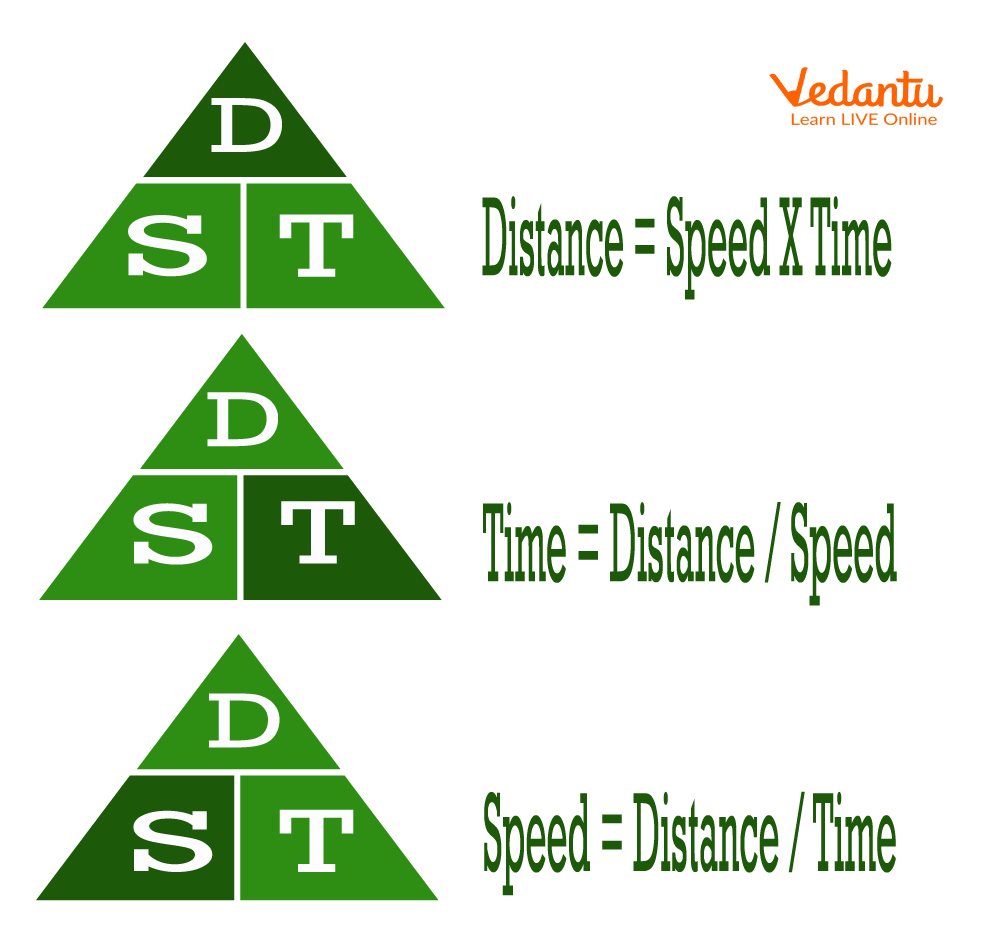
Rearrangement of Formula
In the above-given picture, three different triangles are derived from the formula of speed. In the first triangle, the distance (D) is marked as dark green colour, which means that the formula for distance is given. In the second triangle, the time (T) is marked as dark green, which means that the formula for time is given. In the third triangle, the speed (S) is marked as dark green colour, which means the speed calculation formula can be used to find the speed.
Method for Calculating Average Speed Formula
There is usually a simple formula for calculating the average speed formula.
Speed = $\dfrac{\mathrm{Distance}}{\mathrm{Time}}$
It is possible, however, to use two different speeds for different distances or for different periods of time. It is possible to calculate the average speed using other formulas in these situations. Real-life problems and standardised tests often contain these problems, so learning these formulas and methods is beneficial.
How Does Speed Affect Time and Distance?
Speed involves both distance and time. "Faster" means either "farther" (greater distance) or "sooner" (less time). Doubling one's speed would mean doubling one's distance travelled in a given amount of time. Doubling one's speed would also mean halving the time required to travel a given distance.
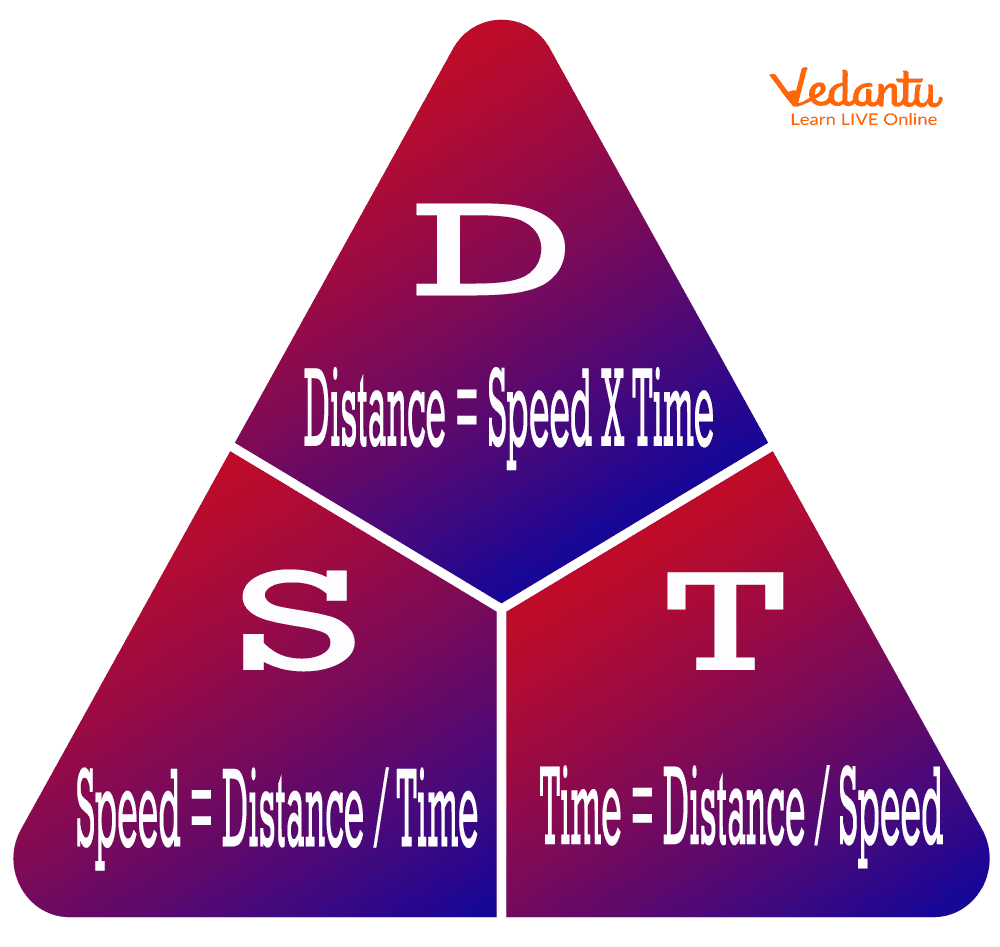
Formula in Triangles
Solved Examples
Example 1: What's your speed if you travel 3600 m in 30 minutes?
Ans: Using the speed calculation formula,
Speed = Distance/ Time
$\dfrac{3600}{30 \times 60}$ = 2m/s = speed
Method for Measuring Speed
Most Americans measure speed in miles per hour or mph. In most cases, cars are measured by this method. In Physics, meters per second (m/s) is usually used to calculate speed.
Speed Can be Measured in Two Different Ways:
Instantaneous Speed - The speed of a particular moment. Depending on how quickly the car is driving at this moment, it might slow down or speed up over the next hour.
Distance covered by an object over a given time interval is the basis for calculating the average speed. 50 miles travelled in an hour will result in 50 mph. While the car may have been travelling at instantaneous speeds of 40 mph and 60 mph during that period, its average speed was 50 mph.
Summary
Here we have learnt about the mathematical relation between speed, distance and time. The speed of a moving body is the distance it travels in unit time. A body moving for the same time will travel the same distance.
Distance = speed x time
Speed = distance/ time
To find time, apply the formula for time, t = d/ s, which means time equals distance divided by speed.
If the distance is in km and time is in hours, then the speed is km/hr.
If the distance is in m and the time is in seconds, then the speed is m/sec.
FAQs on Speed Formula: Definition, Examples & Easy Calculations
1. What are the three formulas for speed?
Speed can be calculated using three main formulas:
- Speed = Distance ÷ Time ($v = \frac{d}{t}$)
- Average Speed = Total Distance ÷ Total Time
- Speed = Initial Velocity + Acceleration × Time ($v = u + at$) for uniform acceleration. Each formula applies to different situations.
2. How to calculate speed?
To calculate speed, divide the distance traveled by the time taken. The formula is $\text{Speed} = \frac{\text{Distance}}{\text{Time}}$. Use this when an object moves at a constant rate, ensuring both distance and time use compatible units.
3. How to find speed in km/h?
To find speed in km/h, measure the distance in kilometers and the time in hours. Divide the kilometers by hours: $\text{Speed (km/h)} = \frac{\text{Distance (km)}}{\text{Time (h)}}$. This ensures your result will be in kilometers per hour.
4. How to find speed and velocity?
Speed is found by dividing total distance by time taken. Velocity is the rate of change of displacement with time. Both use $v = \frac{d}{t}$, but velocity includes direction while speed does not.
5. What is the average speed formula?
The average speed formula calculates how fast an object moves over the whole journey. It is:
- Average Speed = Total Distance Travelled ÷ Total Time Taken.
6. Can speed be negative?
Speed measures how fast something moves, but it is always a positive value or zero, since distance and time cannot be negative. Unlike velocity, speed does not include direction and therefore cannot be negative.
7. Why is unit conversion important in the speed formula?
In the speed formula, it is important to use compatible units for distance and time. For example, if distance is in meters and time in seconds, speed is in meters per second (m/s). Mixing units may give incorrect results.
8. What is the SI unit for speed?
The SI unit for speed is meters per second (m/s). In calculations using the speed formula, keeping distance in meters and time in seconds ensures you use the correct unit for scientific accuracy.
9. How do you use the speed formula in real life?
People use the speed formula to find how fast a car travels, the pace of a runner, or the speed of a train. By dividing distance by travel time, anyone can calculate speed in real-world activities for safety and planning.
10. How is speed different from velocity in the speed formula?
Speed is the rate of motion without regard to direction, while velocity includes the direction of movement. Both use $v = \frac{d}{t}$, but velocity is a vector and can be positive or negative based on direction.
11. What does a speed of 0 mean?
A speed of 0 means that an object is not changing its position over time, so it is at rest. In the speed formula, if distance does not increase while time passes, the calculated speed is zero.
