




Square Root of 11: An Introduction
One can easily find the square of a number by multiplying the number by itself but finding the square root of a number is not always straightforward especially, when the given number is either not a perfect square or a prime number or a decimal number. There are very few methods of finding the square roots of such numbers.
What is the value of root 11? 11 is a non-perfect-square as well as a prime number. So, the square root of 11 must be a decimal number and not an integer. Moreover, the square root of 11 can be expressed as a never-ending and non-recurring decimal number $3.316624790355...$. So, the square root of 11 can’t be found exactly; it is always given approximately by rounding up or down, and the square root up to 7 decimal places is 3.3166248. In radical form, it can be expressed as $\sqrt{11}$ and in exponent form as ${{11}^{\dfrac{1}{2}}}$ or ${{(11)}^{0.5}}$.
Definition of Square Root of a Number
It is well known that the square of a number is the number obtained after multiplying the number by itself. The inverse operation of the square is the square root. Consider a number N whose square root is a number SR, which, when multiplied by itself, produces the result N., i.e., $SR\times SR=N$ or $S{{R}^{2}}=N$. It is represented as $\sqrt{N}=SR$. For example, the square root of 4 is 2 as ${{2}^{2}}=4$; $\sqrt{1.69}=1.3$; $\sqrt{5}=2.236068$ (approx.).
What is the Square Root of 11?
The square root of 11 is a number that gives the product 11 after being multiplied by itself. It is represented as $\sqrt{11}$ in radical form and ${{11}^{\dfrac{1}{2}}}$ in exponent form. It is a non-recurring decimal number $3.316624790355...$. The square root 11 value rounded up to 7 decimal places is 3.3166248.
Did you ever notice that if s and n are numbers such that ${{s}^{2}}=n$, then ${{(-s)}^{2}}=n$? So, it is obvious that the square root of $n$ is not only $s$ but also $-s$ i.e., $\sqrt{n}=\pm s$. Hence, the square root of 11 rounded up to 7 decimal places is $\sqrt{11}=\pm 3.3166248$.
Methods of Finding the Square Root of 11
The square root of a number can be found by the following methods:
Repeated Subtraction
Estimation
Only the square root of a perfect square number can be found by the prime factorization method by simplifying the radical of the number. As 11 is not a perfect square and also a prime number, it cannot be factored further, and its square root cannot be found by the prime factorisation method. To find the square root of a number, one number from each pair of the same numbers from its prime factorisation is taken, and then they are multiplied, and the obtained product will be the desired square root of the given number.
As 11 is a prime number, its prime factorization is $11=1\times 11$, i.e., none of the factors of 11 is repeated. Therefore, $\sqrt{11}$ is the simplest radical form. The repeated Subtraction method is also used to find the square root of a perfect square and cannot be applied here to find the square root of 11.
Finding the Square Root of 11 by Long Division Method
The method of finding the square root of 11 by Long Division Method involves the following few square root method steps:
Step-1: Beginning from the rightmost digit of the given number, the digits are paired up by putting a bar above them. As 11 is a two-digit number, the whole number lies under one bar. Rather, we write 11 as $\overline{11}.\overline{00}\overline{00}\overline{00}\overline{00}\overline{00}$.
Step-2: At the next step, the largest possible number is to be found such that the square of that number is less than or equal to the leftmost single digit or the left-most pair of the given number. Here, for $\overline{11}.\overline{00}\overline{00}\overline{00}\overline{00}\overline{00}$, the left-most pair is $\overline{11}$, the largest possible number whose square is less than 11 is 3. So, the divisor is 3, the quotient is 3, and the remainder becomes 2.
Step-3: At the next step, the divisor should be double of the present quotient and it is written with a blank on its right. Then the largest possible digit should be guessed to fill in the blank that will also become a new digit to the right of the quotient such that the divisor, when multiplied to the new quotient, gives the product less than or equal to the dividend. Repeat this process to which decimal places you want to find the square root.
The whole process can be given as follows:
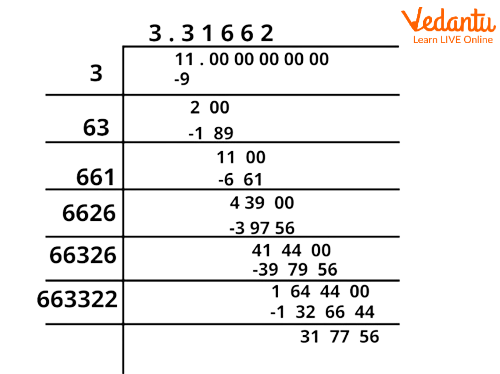
Square Root of 11 by Long Division Method
Is the Square Root of 11 a Rational Number or an Irrational Number?
A rational number is a number that can be expressed as $\dfrac{p}{q}$, where
$p$ and $q$ are integers and
$q \neq 0$
For example, \[2,\text{ }4,\text{ }\dfrac{1}{2},\text{ }-\dfrac{4}{5}\] etc. All positive and negative integers, all fractions are rational numbers. Non-terminating recurring decimal numbers that have repeated numbers after the decimal points are also rational numbers.
On the other side, an irrational number is a number that cannot be expressed as \[\dfrac{p}{q}\] i.e., the ratio of two integers. Non-terminating non-recurring decimal numbers are irrational numbers. For example, $\pi ,\text{ }e,\text{ }\sqrt{2},\text{ }\sqrt{3}$ etc. As we have seen before, that the square root of 11 is a non-terminating non-recurring decimal number $3.316624790355...$, therefore, the square root of 11 is an irrational number.
Interesting Facts
$-\sqrt{11}$ is also a square root of 11.
The square roots of 11 are $\pm 3.31662$.
The square root of 11 is the solution of the quadratic equation ${{x}^{2}}=11$.
Solved Example
Example 1: If the square root of 11 is 3.317, find the value of the square root of 0.11.
Solution: First, let us write the given decimal number 0.11 in fractions; then we get $0.11=\dfrac{11}{100}$.
So,$\sqrt{0.11}=\sqrt{\dfrac{11}{100}}=\dfrac{\sqrt{11}}{\sqrt{100}}=\dfrac{3.317}{10}=0.3317$.
Example 2: Steve is making a square cake. Steve decides to serve each guest a 1-inch square piece of cake. The party will be attended by $121$ people. How long should the sides of his square cake be so that he can serve each guest one piece?
Solution: There should be $121$ cake pieces since there are $121$ guests.
Area of the square cake $=121$ sq in.
Therefore, the length of the sides of the cake $=\sqrt{121}=11$
Hence, the length of the sides of the square cake $=11$ inches.
Conclusion
11 is not a perfect square number and also, and the square root of 11 is not only a decimal number but also a never-ending non-recurring decimal number $3.316624790355...$ that can be found only by the method of long division. It is an example of an irrational number and the square roots of 11 rounded up to 7 decimal places are $\pm 3.3166248$.
Practice Questions
1. If a circle has an area of $\pi \times 11 cm^2$, then. Find the circle's radius.
2. What is the square root of 11 in the simplest radical form?
3. Find the square root of 13.
Answers
1. $3.317 cm$
2. $\sqrt{11}$ or $-\sqrt{11}$
3. 3.6055513
FAQs on Square Root of 11
1. Describe the simplified radical form of the square root of 11.
To make the square root of 11 simpler, represent it as the product of its prime factors. 11 can be divided into prime factors using the formula 11 x 1. A digit's square root can be found by multiplying any number from each pair of the numbers that make up its prime factorization. There are no pairs of the same numbers in the prime factorization of the number 11, which is 11 × 1. As a result, the square root of 11 has reached its simplest form($\sqrt{11 × 1}$) and cannot be further simplified.
2. What is the square root of -11?
We know that the square of any real number must be positive even if it is true for a negative number also. Because if $-n$ is a negative number, then its square will be ${{(-n)}^{2}}=(-n)\times (-n)=+{{n}^{2}}$. Hence, the square of any real number cannot be negative. So, the square root of any negative number cannot be a real number. This involves the concept of an imaginary number or complex number i.e., the square root of -11 is an imaginary number and it can be written as $\sqrt{-11}=\sqrt{-1}\times \sqrt{11}=i\sqrt{11}=3.31662i$ , where $i=\sqrt{-1}$ is called the imaginary unit.
3. How do you calculate the square root of $-11$?
Negative $11$'s square root is not a real number. The square root of negative $11$ can be calculated using a complex or imaginary square root number. Making the imaginary square root of -1 is the first step. This allows us to combine real and imaginary square roots to prove the following: $\sqrt{-11} = \sqrt{11} \times \sqrt{-1}$. The square root of $11$ is $3.317$. In addition, the imaginary (iota), which can be written as $\iota$ is the square root of the negative one. We now have a solution $\sqrt{-11} = 3.317 \iota$.























