An Introduction to Fractions
In Mathematics, fractions are frequently used terms. As children go through the grades, they are introduced to a variety of words, such as fractions, 1 halves, thirds, and fourths. It is now common to be confused about how much 1 half is or what half means. In simpler terms, halves can be defined as the two equal parts of a whole object.
To better comprehend these phrases, however, we must first comprehend the concept of fractions. A fraction is defined as a piece of a larger collection. The numerator and denominator are the two halves of the fraction. The illustration below can help you understand the notion of fractions.
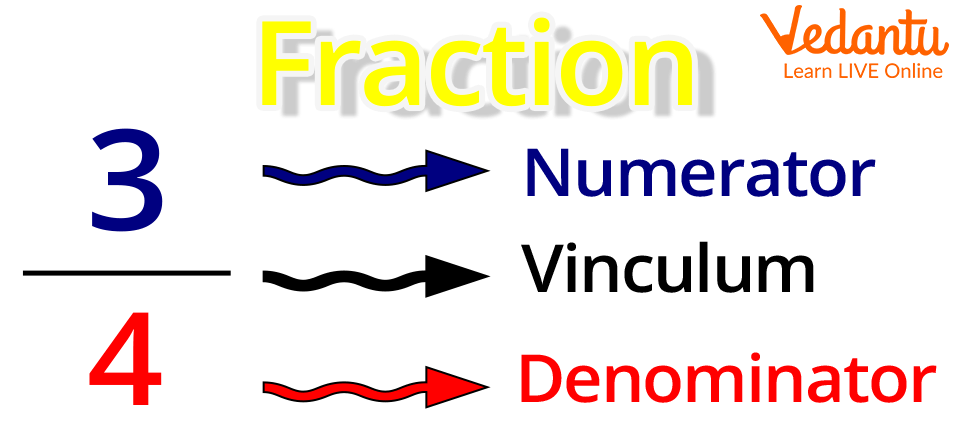
The Fraction Parts – The Numerator and Denominator
Since we have developed an understanding of what fractions are, let us now discuss what halves and their properties.
What Do Halves Mean?
Halves can be defined as the value of the equal division of a whole object. The term half or halves is used to mathematically represent the value of $\frac{1}{2}$. A half divides the whole object into two equivalent parts. Let us see some examples for a better understanding of the concept.
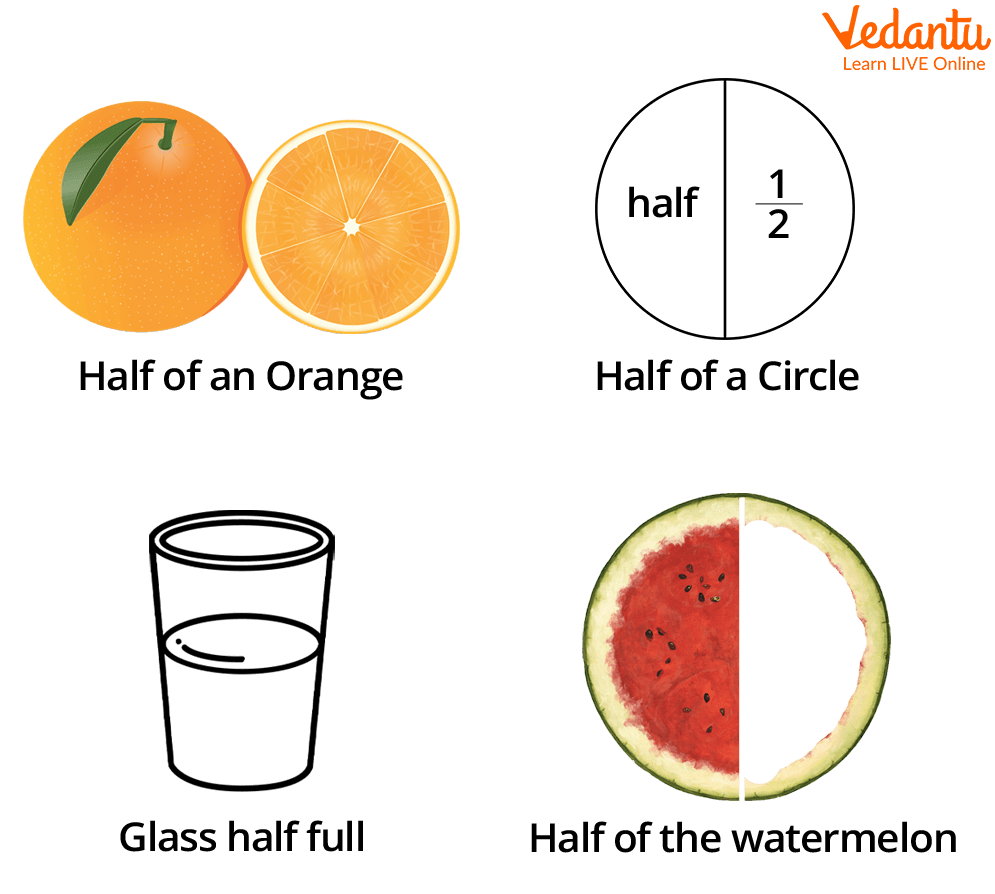
Examples of Halves
Calculation of the Value of Halves
Since we have now understood how to represent half in a fraction. Let us understand how to calculate the half for a given value. A simple method to calculate half of a value is to divide the total value by 2. It can be better understood by an example.
Example
If a person has 10 chocolates and is asked to give exactly half of the chocolate to their friend. How many chocolates would they have to give?
Since we know we can calculate the value of half by dividing the value by 2. The total number of chocolate is 10, so the value of half would be $\frac{10}{2}$ which is equal to 5. The person would give exactly 5 chocolates to their friend.
Representing Half on a Number Line
Let us look at how we can represent the value of half in a number line. On the number line, we can represent fractions. On a number line, the distance from 0 to 1 is always one whole. When we divide this distance in half, we will get two halves.
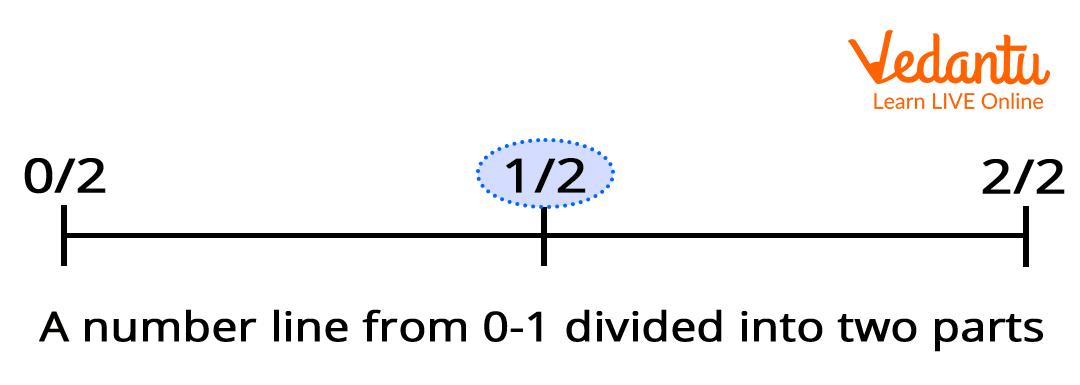
The Value of Half on a Number Line.
Conclusion
The terms half and halves are regularly used in fractions, as we've learnt. We have also learnt how to find the half-value of any number. We hope that we were able to aid in the development of a deeper understanding of the topic. To master the concept of fractions, children can practice solving various questions.
FAQs on What are Halves? Its Meaning and Examples
1. What do two halves constitute?
The two halves when added together give the value of the whole. A half is defined as the equal part of the whole object. This can be understood by the example. If the length of a half of a log is 10 metres, then the length of the whole log would be the sum of both the pieces, i.e. 10+10 = 20. The whole length would be 20 metres.
2. How can we represent halves in decimal?
One-half is represented as 0.5 in decimal form. The fractional representation of the value of half is $\frac{1}{2}$. When $\frac{1}{2}$ is simplified, we get the value as 0.5. We can also prove this by adding 0.5 and 0.5, the sum of this addition is equal to 1.