NCERT Solutions for Class 11 Maths Chapter 5 Linear Inequalities Ex 5.1

Exercise 5.1

1. Solve $\text{24x}<\text{100}$ when 

(i) $\text{x}$ is a natural number 

Ans: We are given $\text{24x}<\text{100}$.

$ 24\text{x}<100 $

$ \Rightarrow \frac{\text{24x}}{\text{24}}\text{}<\frac{\text{100}}{\text{24}} $

$ \Rightarrow \text{x}<\frac{25}{6} $

Therefore, we can say that only $1,2,3,4$ are only natural numbers less than $\frac{25}{6}$.

Therefore, the solution set is $\left\{ 1,2,3,4 \right\}$

(ii) $\text{x}$ is an integer.

Ans: We are given $\text{24x}<\text{100}$.

$ 24\text{x}<100 $

$\Rightarrow \frac{\text{24x}}{\text{24}}<\frac{\text{100}}{\text{24}} $ 

$ \Rightarrow \text{x}<\frac{\text{25}}{\text{6}}$

Therefore, we can say that only $..................-3,-2,-1,0,1,2,3,4.$ the integers less than $\frac{25}{6}$.

So, the solution set is $\left\{ ...............-3,-2,-1,0,1,2,3,4 \right\}$.

2. Solve $\mathrm{-12x}>\mathrm{30}$, when

(i) $\text{x}$ is a natural number 

Ans: We are given $\text{-12x}>\text{30}$.

$ \text{-12x}>\text{30}$ 

$ \Rightarrow \frac{-12}{12}\text{x}>\frac{30}{12} $ 

$ \Rightarrow -\text{x}>\frac{5}{2} $ 

$ \Rightarrow \text{x}<-\frac{5}{2} $

Therefore, we can say that there is no natural number less than $\left( -\frac{5}{2} \right)$

So, there is no solution set for the given condition.

(ii) $\mathrm{x}$ is an integer.

We are given $\text{-12x}>\text{30}$.

$\text{-12x}>\text{30} $ 

$ \Rightarrow \frac{-12}{12}\text{x}>\frac{30}{12} $ 

$ \Rightarrow -\text{x}>-\frac{5}{2} $ 

$ \Rightarrow \text{x}<\frac{5}{2}$ 

Therefore, we can say that $.........-5,-4,-3$ are the numbers that satisfy the given inequality.

Hence, the solution set is $\left\{ .....-5,-4,-3 \right\}$.

3. Solve $\mathrm{5x-3}<\mathrm{7}$, when

(i) $\text{x}$ is an integer. 

Ans: We are given $\text{5x-3}<7$.

$ \text{5x-3}<7 $ 

$ \Rightarrow \text{5x-3+3}<7+3 $ 

$ \Rightarrow \text{5x}<10 $ 

$ \Rightarrow \frac{\text{5}}{\text{5}}\text{x}<\frac{10}{5} $ 

$ \Rightarrow \text{x}<2$

Therefore, we can say that $........-4,-3,-2,-1,0,1$ are the integers that satisfy the given inequality.

So, the solution set is $\left\{ ........-4,-3,-2,-1,0,1 \right\}$

(ii) $\mathrm{x}$ is a real number.

We are given $\text{5x-3}<7$.

$\text{5x-3}<7 $ 

$ \Rightarrow \text{5x-3+3}<7+3 $ 

$ \Rightarrow \text{5x}<10 $ 

$ \Rightarrow \frac{\text{5}}{\text{5}}\text{x}<\frac{10}{5} $ 

$ \Rightarrow \text{x}<2$

Therefore, we can say that the real numbers which are less than $2$ will satisfy the inequality.

Hence, the solution set is $\text{x}\in \left( -\infty ,2 \right)$.

4. Solve $\mathrm{3x+8}>\mathrm{2}$, when

(i) $\text{x}$ is an integer. 

Ans: We are given $\text{3x+8}>2$.

$3\text{x}+8>2 $ 

$ \Rightarrow 3\text{x}+8-8>2-8 $ 

$ \Rightarrow 3\text{x}>-6 $ 

$ \Rightarrow \frac{3}{3}\text{x}>\frac{-6}{3} $ 

$ \Rightarrow \text{x}>-2$

Therefore, we can say that $-1,0,1,2,3.........$ are the integers that satisfy the given inequality.

So, the solution set is $\left\{ -1,0,1,2,3......... \right\}$

(ii) $\mathrm{x}$ is a real number.

$\text{3x+8}>\text{2} $ 

$ \Rightarrow 3\text{x}+8-8>2-8 $ 

$ \Rightarrow 3\text{x}>-6 $ 

$ \Rightarrow \frac{3}{3}\text{x}>\frac{-6}{3} $ 

$ \Rightarrow \text{x}>-2$

Therefore, we can say that the real numbers which are greater than $-2$ will satisfy the inequality.

Hence, the solution set is $\text{x}\in \left( -2,\infty\right)$.

5. Solve the given inequality for real $\mathrm{x:4x+3}<\mathrm{5x+7}$

Ans: Let us rewrite the given inequality, we get

$\text{4x+3}<\text{5x+7} $ 

$ \Rightarrow \text{4x+3-7}<\text{5x+7-7} $ 

$ \Rightarrow \text{4x-4}<\text{5x} $ 

$ \Rightarrow \text{4x-4-4x}<\text{5x-4x} $ 

$ \Rightarrow \text{-4}<\text{x}$

Therefore, we can say that the numbers greater than $\text{-4}$ will be the solutions that satisfy the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -4,\infty\right)$.

6. Solve the given inequality for real $\mathrm{x:3x-7}>\mathrm{5x-1}$

Ans: Let us rewrite the given inequality, we get

$3\text{x}-7>5x-1 $ 

$ \Rightarrow 3\text{x}-7\text{+75x-1+7} $ 

$ \Rightarrow 3\text{x}>\text{5x+6} $ 

$ \Rightarrow 3\text{x}-5\text{x}>5\text{x}-5\text{x}+6 $ 

$ \Rightarrow -2\text{x}>6 $ 

$ \Rightarrow \frac{-2}{-2}\text{x}<-\frac{6}{-2} $ 

$ \Rightarrow \text{x}<-3 $

Therefore, we can say that the numbers less than $\text{-3}$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,-3 \right)$.

7. Solve the given inequality for real $\mathrm{x:3}\left( \mathrm{x-1} \right)\leq\mathrm{2}\left( \mathrm{x-3} \right)$

Ans: Let us rewrite the given inequality, we get

$\text{3}\left( \text{x-1} \right)\leq\text{2}\left( \text{x-3} \right) $ 

$ \Rightarrow \text{3x-3}\leq\text{2x-6} $ 

$ \Rightarrow \text{3x-3+3}\leq\text{2x-6+3} $ 

$ \Rightarrow \text{3x}\leq\text{2x-3} $ 

$ \Rightarrow 3\text{x-2x}\leq\text{2x-2x-3} $ 

$ \Rightarrow \text{x}\leq\text{-3} $

Therefore, we can say that the numbers less than or equal to $\text{-3}$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,-3 \right]$.

8. Solve the given inequality for real $\mathrm{x:3}\left( \mathrm{2-x} \right)\geq\mathrm{2}\left( \mathrm{1-x} \right)$

Ans: Let us rewrite the given inequality, we get

$ \text{3}\left( \text{2-x} \right)\geq\text{2}\left( \text{1-x} \right) $ 

$ \Rightarrow \text{6-3x}\geq\text{2-2x} $ 

$ \Rightarrow \text{6-6-3x}\geq\text{2-6-2x} $ 

$ \Rightarrow \text{-3x}\geq\text{-4-2x} $ 

$ \Rightarrow \text{-3x+2x}\geq\text{-4-2x+2x} $ 

$ \Rightarrow \text{-x}\geq\text{-4} $ 

$ \Rightarrow \text{x}\leq\text{4} $

Therefore, we can say that the numbers less than or equal to $4$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,4 \right]$.

9. Solve the given inequality for real $\mathrm{x:x+}\frac{\mathrm{x}}{\mathrm{2}}\mathrm{+}\frac{\mathrm{x}}{\mathrm{3}}<\mathrm{11}$

Ans: Let us rewrite the given inequality, we get

$\text{x+}\frac{\text{x}}{\text{2}}\text{+}\frac{\text{x}}{\text{3}}<\text{11}$ 

$ \Rightarrow \text{x}\left( \text{1+}\frac{\text{1}}{\text{2}}\text{+}\frac{\text{1}}{\text{3}} \right)<\text{11} $ 

$ \Rightarrow \text{x}\left( \frac{\text{6+3+2}}{\text{6}} \right)<\text{11}$ 

$ \Rightarrow \text{x}\left( \frac{\text{11}}{\text{6}} \right)<\text{11} $ 

$ \Rightarrow \text{x}\left( \frac{\text{11}}{\text{6}} \right)\text{ }\!\!\times\!\!\text{ }\left( \frac{\text{6}}{\text{11}} \right)\text{11 }\!\!\times\!\!\text{ }\frac{\text{6}}{\text{11}} $ 

$ \Rightarrow \text{x}<\text{6} $

Therefore, we can say that the numbers less than $6$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,6 \right)$.

10. Solve the given inequality for real $\mathrm{x:}\frac{\mathrm{x}}{\mathrm{3}}>\frac{\mathrm{x}}{\mathrm{2}}\mathrm{+1}$

Ans: Let us rewrite the given inequality, we get

$\frac{\text{x}}{\text{3}}>\frac{\text{x}}{\text{2}}\text{+1} $ 

$ \Rightarrow \frac{\text{x}}{\text{3}}\text{-}\frac{\text{x}}{\text{2}}>\frac{\text{x}}{\text{2}}\text{-}\frac{\text{x}}{\text{2}}\text{+1} $ 

$ \Rightarrow \text{x}\left( \frac{\text{1}}{\text{3}}\text{-}\frac{\text{1}}{\text{2}} \right)>\text{1} $ 

$ \Rightarrow \text{x}\left( \frac{\text{-1}}{6} \right)>\text{1} $ 

$ \Rightarrow \text{x}\left( \frac{\text{-1}}{6} \right)\text{ }\!\!\times\!\!\text{ }\left( \frac{\text{-6}}{1} \right)<\text{-6} $ 

$ \Rightarrow \text{x}<\text{-6} $

Therefore, we can say that the numbers less than $-6$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,-6 \right)$.

11. Solve the given inequality for real $\mathrm{x:}\frac{\mathrm{3}\left( \mathrm{x-2} \right)}{\mathrm{5}}\leq\frac{\mathrm{5}\left( \mathrm{2-x} \right)}{\mathrm{3}}$

Ans: Let us rewrite the given inequality, we get

$\frac{\text{3}\left( \text{x-2} \right)}{\text{5}}\leq\frac{\text{5}\left( \text{2-x} \right)}{\text{3}} $ 

$ \Rightarrow \text{9}\left( \text{x-2} \right)\leq\text{25}\left( \text{2-x} \right)$ 

$ \Rightarrow \text{9x-18}\leq\text{50-25x} $ 

$ \Rightarrow \text{9x+25x-18}\leq\text{50-25x+25x} $ 

$ \Rightarrow \text{34x-18}\leq\text{50} $ 

$ \Rightarrow \text{34x-18+18}\leq\text{50+18} $ 

$ \Rightarrow \text{34x}\leq\text{68} $ 

$ \Rightarrow \text{x}\leq\frac{\text{68}}{\text{34}} $ 

$ \Rightarrow \text{x}\leq\text{2} $

Therefore, we can say that the numbers less than or equal to $2$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,2 \right]$.

12. Solve the given inequality for real $\mathrm{x:}\frac{\mathrm{1}}{\mathrm{2}}\left( \frac{\mathrm{3x}}{\mathrm{5}}\mathrm{+4} \right)\geq\frac{\mathrm{1}}{\mathrm{3}}\mathrm{x-2}$

Ans: Let us rewrite the given inequality, we get

$\frac{\text{1}}{\text{2}}\left( \frac{\text{3x}}{\text{5}}\text{+4} \right)\geq\frac{\text{1}}{\text{3}}\left( \text{x-6} \right) $ 

$ \Rightarrow \text{3}\left( \frac{\text{3x}}{\text{5}}\text{+4} \right)\geq\text{2}\left( \text{x-6} \right) $ 

$ \Rightarrow \frac{\text{9}}{\text{5}}\text{x+12}\geq\text{2x-12} $ 

$ \Rightarrow \frac{\text{9}}{\text{5}}\text{x+12-12}\geq\text{2x-12-12} $ 

$ \Rightarrow \frac{\text{9}}{\text{5}}\text{x}\geq\text{2x-24} $ 

$ \Rightarrow \text{9x}\geq\text{10x-120} $ 

$ \Rightarrow \text{9x-10x}\geq\text{10x-10x-120} $ 

$ \Rightarrow \text{-x}\geq\text{-120} $ 

$ \Rightarrow \text{x}\leq\text{120} $

Therefore, we can say that the numbers less than or equal to $120$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,120 \right]$.

13. Solve the given inequality for real $\mathrm{x:2}\left( \mathrm{2x+3} \right)\mathrm{-10}<\mathrm{6}\left( \mathrm{x-2} \right)$

Ans: Let us rewrite the given inequality, we get

$\text{2}\left( \text{2x+3} \right)\text{-10}<\text{6}\left( \text{x-2} \right) $ 

$ \Rightarrow \text{4x+6-10}<\text{6x-12} $ 

$ \Rightarrow \text{4x-4}<\text{6x-12} $ 

$ \Rightarrow \text{4x-4+4}<\text{6x-12+4} $ 

$ \Rightarrow \text{4x}<\text{6x-8} $ 

$ \Rightarrow \text{4x-6x}<\text{6x-6x-8} $ 

$ \Rightarrow \text{-2x}<\text{-8} $ 

$ \Rightarrow \text{x}>\text{4}$

Therefore, we can say that the numbers greater than $4$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( 4,\infty\right)$.

14. Solve the given inequality for real $\mathrm{x:37-}\left( \mathrm{3x+5} \right)\geq\mathrm{9x-8}\left( \mathrm{x-3} \right)$

Ans: Let us rewrite the given inequality, we get

$\text{37-}\left( \text{3x+5} \right)\geq\text{9x-8}\left( \text{x-3} \right) $ 

$ \Rightarrow \text{37-3x-5}\geq\text{9x-8x+24} $ 

$ \Rightarrow \text{-3x+32}\geq\text{x+24} $ 

$ \Rightarrow \text{-3x-x+32}\geq\text{x-x+24} $ 

$ \Rightarrow \text{-4x+32}\geq\text{24} $ 

$ \Rightarrow \text{-4x+32-32}\geq\text{24-32} $ 

$ \Rightarrow \text{-4x\geq-8} $ 

$ \Rightarrow \text{x\leq2}$

Therefore, we can say that the numbers less than or equal to $2$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,2 \right]$.

15. Solve the given inequality for real $\mathrm{x:}\frac{\mathrm{x}}{\mathrm{4}}<\frac{\left( \mathrm{5x-2} \right)}{\mathrm{3}}\mathrm{-}\frac{\left( \mathrm{7x-3} \right)}{\mathrm{5}}$

Ans: Let us rewrite the given inequality, we get

$\frac{\text{x}}{\text{4}}<\frac{\left( \text{5x-2} \right)}{\text{3}}\text{-}\frac{\left( \text{7x-3} \right)}{\text{5}} $ 

$ \Rightarrow \frac{\text{x}}{\text{4}}<\frac{\text{5}\left( \text{5x-2} \right)\text{-3}\left( \text{7x-3} \right)}{\text{15}} $ 

$ \Rightarrow \frac{\text{x}}{\text{4}}<\frac{\text{25x-10-21x+9}}{\text{15}} $ 

$ \Rightarrow \frac{\text{x}}{\text{4}}<\frac{\text{4x-1}}{\text{15}} $ 

$ \Rightarrow \text{15x}<\text{4}\left( \text{4x-1} \right) $ 

$ \Rightarrow \text{15x-16x}<\text{16x-16x-4} $ 

$ \Rightarrow \text{-x}<\text{-4} $ 

$\text{x}>\text{4}$

Therefore, we can say that the numbers greater $4$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( 4,\infty\right)$.

16. Solve the given inequality for real $\mathrm{x:}\frac{\left( \mathrm{2x-1} \right)}{\mathrm{3}}\geq\frac{\left( \mathrm{3x-2} \right)}{\mathrm{4}}\mathrm{-}\frac{\left( \mathrm{2-x} \right)}{\mathrm{5}}$

Ans: Let us rewrite the given inequality, we get

$\frac{\left( \text{2x-1} \right)}{\text{3}}\geq\frac{\left( \text{3x-2} \right)}{\text{4}}\text{-}\frac{\left( \text{2-x} \right)}{\text{5}} $ 

$ \Rightarrow \frac{\left( \text{2x-1} \right)}{\text{3}}\geq\frac{\text{5}\left( \text{3x-2} \right)\text{-4}\left( \text{2-x} \right)}{\text{20}} $ 

$ \Rightarrow \frac{\left( \text{2x-1} \right)}{\text{3}}\text{=}\frac{\text{15x-10-8+4x}}{\text{20}} $ 

$ \Rightarrow \frac{\left( \text{2x-1} \right)}{\text{3}}\geq\frac{\text{19x-18}}{\text{20}} $ 

$ \Rightarrow \text{20}\left( \text{2x-1} \right)\geq\text{3}\left( \text{19x-18} \right) $ 

$ \Rightarrow \text{40x-20}\geq\text{57x-54} $ 

$ \Rightarrow \text{-20+54}\geq\text{57x-40x} $ 

$ \Rightarrow \text{34}\geq\text{17x} $ 

$ \Rightarrow \text{2}\geq\text{x}$

Therefore, we can say that the numbers less than or equal to $4$ will be in the solution set for satisfying the given condition.

Therefore, we can say that the solution set for the given inequality is $\left( -\infty ,2 \right]$.

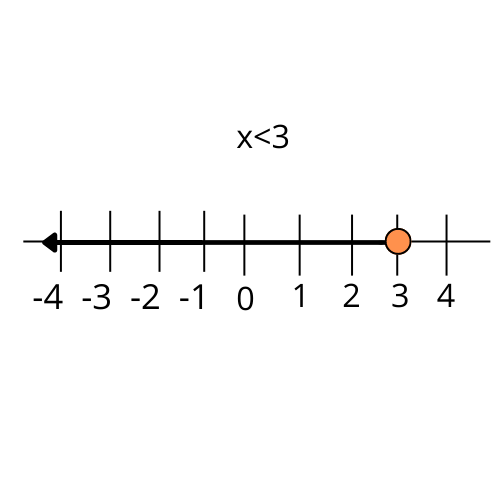
17. Solve the inequality and show the graph of the solution in number line:

 $\mathrm{3x-2}<\mathrm{2x+1}$

Ans: Let us rewrite the given inequality, we get

$\text{3x-2}<\text{2x+1} $ 

$ \Rightarrow \text{3x-2x}<\text{1+2} $ 

$ \Rightarrow \text{x}<\text{3}$

Therefore, the graphical representation for the inequality in number line is:

18. Solve the inequality and show the graph of the solution in number line:

$\mathrm{5x-3}\geq\mathrm{3x-5}$

Ans: Let us rewrite the given inequality, we get

$\text{5x-3}\geq\text{3x-5} $ 

$ \Rightarrow \text{5x-3x}\geq\text{-5+3} $ 

$ \Rightarrow \text{2x}\geq\text{-2} $ 

$ \Rightarrow \frac{\text{2x}}{\text{2}}\geq\frac{\text{-2}}{\text{2}} $ 

$ \Rightarrow \text{x}\geq\text{-1}$

Therefore, the graphical representation for the inequality in number line is:

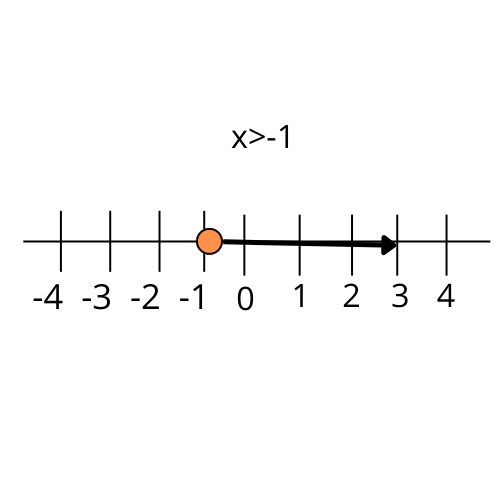
19. Solve the inequality and show the graph of the solution in number line:

$\mathrm{3}\left( \mathrm{1-x} \right)<\mathrm{2}\left( \mathrm{x+4} \right)$

Ans: Let us rewrite the given inequality, we get

$\text{3}\left( \text{1-x} \right)<\text{2}\left( \text{x+4} \right) $ 

$ \Rightarrow 3-3\text{x}<\text{2x+8} $ 

$ \Rightarrow \text{3-8}<\text{2x+3x} $ 

$ \Rightarrow \text{-5}<\text{5x} $ 

$ \Rightarrow \frac{-5}{5}<\frac{5\text{x}}{5} $ 

$ \Rightarrow -1<\text{x} $

Therefore, the graphical representation for the inequality in number line is:

20. Solve the inequality and show the graph of the solution in number line:

$\frac{\mathrm{x}}{\mathrm{2}}\geq\frac{\left( \mathrm{5x-2} \right)}{\mathrm{3}}\mathrm{-}\frac{\left( \mathrm{7x-3} \right)}{\mathrm{5}}$

Ans: Let us rewrite the given inequality, we get

$\frac{\text{x}}{\text{2}}\geq\frac{\left( \text{5x-2} \right)}{\text{3}}\text{-}\frac{\left( \text{7x-3} \right)}{\text{5}} $ 

$ \Rightarrow \frac{\text{x}}{\text{2}}\geq\frac{\text{5}\left( \text{5x-2} \right)\text{-3}\left( \text{7x-3} \right)}{\text{15}} $ 

$ \Rightarrow \frac{\text{x}}{\text{2}}\geq\frac{\text{25x-10-21x+9}}{\text{15}}$ 

$ \Rightarrow \frac{\text{x}}{\text{2}}\geq\frac{\text{4x-1}}{\text{15}} $ 

$ \Rightarrow \text{15x}\geq\text{2}\left( \text{4x-1} \right) $ 

$ \Rightarrow \text{15x}\geq\text{8x-2} $ 

$ \Rightarrow \text{15x-8x}\geq\text{8x-2-8x} $ 

$ \Rightarrow \text{7x}\geq\text{-2} $ 

$ \Rightarrow \text{x}\geq\text{-}\frac{\text{2}}{\text{7}}$

Therefore, the graphical representation for the inequality in number line is:

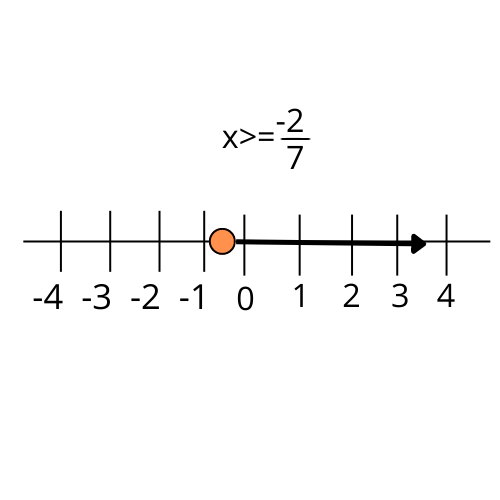
21. Ravi obtained $\mathrm{70}$ and $\mathrm{75}$ marks in the first two unit tests. Find the minimum marks he should get in the third test to have an average of at least $\mathrm{60}$ marks. 

Ans: Let us assume $\text{x}$ as the marks obtained by Ravi in the third unit test.

As it is given that the student must have an average of $\text{60}$ marks,

Therefore, the expression is:

 $\frac{\text{70+75+x}}{\text{3}}\geq\text{60} $ 

$ \Rightarrow \text{145+x}\geq\text{180} $ 

$ \Rightarrow \text{x}\geq\text{180-145} $ 

$ \Rightarrow \text{x}\geq\text{35}$

Therefore, we can say that the student should obtain greater than or equal 

to $35$ marks.

22. To receive Grade ‘A’ in a course, one must obtain an average of $\mathrm{90}$ marks or more in five examinations (each of $\mathrm{100}$ marks). If Sunita’s marks in first examinations are $\mathrm{87,92,94}$ and $\mathrm{95}$, find minimum marks that Sunita must obtain in the fifth examination to get grade ‘A’ in the course.

Ans: Let us assume $\text{x}$ as the fifth examination marked by Sunita.

As it is given that to obtain ‘A’ one should obtain an average of $\text{90}$ marks or

more in five examinations.

So, the expression is:

$\frac{87+92+94+95+\text{x}}{5}\geq90 $ 

$ \Rightarrow \frac{368+\text{x}}{5}\geq90 $ 

$ \Rightarrow 368+\text{x}\geq450 $ 

$ \Rightarrow \text{x}\geq450-368 $ 

$ \Rightarrow \text{x}\geq82$ 

Therefore, we can say that sunita must obtain $\text{82}$ or greater than it to obtain 

‘A’ grade.

23. Find all pairs of consecutive odd positive integers both of which are smaller than $\mathrm{10}$ such that their sum is more than $\mathrm{11}$.

Ans: Let us assume $\text{x}$ as the smallest number in two consecutive odd integers. Then, the other odd integer will be $\text{x+2}$.

As it is given that both the integers are smaller than $\text{10}$, we get 

$\text{x+2}<10 $ 

$ \Rightarrow \text{x}<\text{10-2} $ 

$ \Rightarrow \text{x}8$

Let us assume it as inequality $\left( 1 \right)$, we get

$\text{x}<8.............\left( 1 \right)$

Also, it is given that the sum of the two integers is more than $\text{11}$.

So, 

$\text{x+}\left( \text{x}+2 \right)>11 $ 

$ \Rightarrow 2\text{x}+2>11 $ 

$ \Rightarrow 2\text{x}>11-2 $ 

$ \Rightarrow 2\text{x}>\text{9} $ 

$ \Rightarrow \text{x}>\frac{9}{2} $ 

$ \Rightarrow \text{x}>\text{4}\text{.5}$

Let us assume this as inequality $\left( 2 \right)$

$\text{x}>4.5........\left( 2 \right)$

Therefore, from inequality $\left( 1 \right)$ and $\left( 2 \right)$, we get that

$\text{x}$ can take the values of 

$5$ and $7$. 

Therefore, the required pairs are $\left( 5,7 \right)$ and $\left( 7,9 \right)$.

24. Find all pairs of consecutive even positive integers, both of which are larger than $\mathrm{5}$ such that their sum is less than $\mathrm{23}$.

Ans: Let us assume $\text{x}$ as the smallest number in two consecutive even integers. 

Then, the other odd integer will be $\text{x+2}$.

As it is given that both the integers are greater than $5$, we get

$\text{x}>\text{5}..........\left( 1 \right)$

Also, it is given that the sum of the two integers is less than $23$.

So, 

$\text{x+}\left( \text{x}+2 \right)<23 $ 

$ \Rightarrow 2\text{x}+2<23 $ 

$ \Rightarrow 2\text{x}<\text{23}-2 $ 

$ \Rightarrow 2\text{x}<\text{21} $ 

$ \Rightarrow \text{x}<\frac{21}{2} $ 

$ \Rightarrow \text{x}<\text{10}\text{.5}$

Let us assume this as inequality $\left( 2 \right)$

$\text{x}<10.5........\left( 2 \right)$

From $\left( 1 \right)$ and $\left( 2 \right)$, we obtain

$5<\text{x}<\text{10}\text{.5}$.

Therefore, we can say that $\text{x}$satisfies $\text{6,8}$ and $\text{10}$ values.

Therefore, the pairs that are possible are $\left( 6,8 \right)$, $\left( 8,10 \right)$ and $\left( 10,12 \right)$.

25. The longest side of a triangle is $\mathrm{3}$ times the shortest side and the third side is $\mathrm{2}$cm shorter than the longest side. If the perimeter of the triangle is at least $\mathrm{61}$ cm, find the minimum length of the shortest side.

Ans: Let us assume the shortest side of the given triangle as $\text{x}$.

As it is given that the longest side is the three times the shortest side, we

get longest side$\text{=3x}$ 

Therefore, the length of the other side will be $\left( 3x-2 \right)$

As it is given that the perimeter should be at least $61$ cm, we can write it as:

$\text{xcm+3xcm+}\left( 3\text{x}-2 \right)\text{cm=61cm} $ 

$ \Rightarrow \text{7x-2}\geq\text{61} $ 

$ \Rightarrow \text{7x}\geq\text{61+2} $ 

$ \Rightarrow \text{7x}\geq\text{63} $ 

$ \Rightarrow \text{x}\geq\text{9}$

Therefore, we can say that the shortest side of the given triangle should 

satisfy the inequality$\text{x}\geq\text{9}$.

26. A man wants to cut three lengths from a single piece of board of length $\mathrm{91}$ cm. The second length is to be $\mathrm{3}$ cm longer than the shortest and the third length is to be twice as long as the shortest. What are the possible lengths of the shortest board if the third piece is to be at least $\mathrm{5}$ cm longer than the second?

Ans: Let us assume the length of the shortest piece be $\text{x}$ cm.

From the question we can write that the second and third piece will be $\left( \text{x+3} \right)$ cm and $\text{2x}$ respectively.

As it is given that the three lengths are single piece of board of length $\text{91}$ cm,

$\text{x cm+}\left( \text{x+3} \right)\text{cm+2xcm=91cm}$

$ \Rightarrow \text{4x+3}\leq\text{91} $ 

$ \Rightarrow \text{4x}\leq\text{91-3} $ 

$ \Rightarrow \text{4x}\leq\text{88} $ 

$ \Rightarrow \frac{4}{4}\text{x}\leq\frac{88}{4} $ 

$ \Rightarrow \text{x}\leq22........\left( 1 \right) $

Also, the third piece is at least $5\text{cm}$ longer than the second piece.

$\therefore \text{2x}\geq\left( \text{x+3} \right)+5 $ 

$ \Rightarrow 2\text{x}\geq\text{x+8} $ 

$ \Rightarrow \text{x}\geq\text{8}..........\left( 2 \right)$

Therefore, from $\left( 1 \right)$and $\left( 2 \right)$, we get

$8\leq\text{x}\leq22$

So, we can say that the shortest board should be greater than or equal to 

$8$ cm but less than or equal to $22$ cm.

Conclusion

Linear inequalities are crucial for understanding mathematical conditions involving ranges. Focus on mastering the techniques for solving and graphing inequalities in one and two variables. Pay attention to properties and practical applications. This chapter is significant for building a foundation in algebra and enhancing problem-solving skills. In previous year question papers, 2-3 questions on linear inequalities were typically asked, emphasising their importance in exams. Consistent practice and a clear understanding of concepts are essential for success.

Class 11 Maths Chapter 5: Exercises Breakdown

CBSE Class 11 Maths Chapter 5 Other Study Materials

Chapter-Specific NCERT Solutions for Class 11 Maths

The chapter-wise NCERT Solutions for Class 11 Maths are given below. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.

Important Related Links for CBSE Class 11 Maths