NCERT Solutions for Class 6 Chapter 1 Maths - FREE PDF Download
FAQs on NCERT Solutions for Class 6 Maths Chapter 1 Patterns in Mathematics
1. What topics are covered in Chapter 1, Patterns in Mathematics?
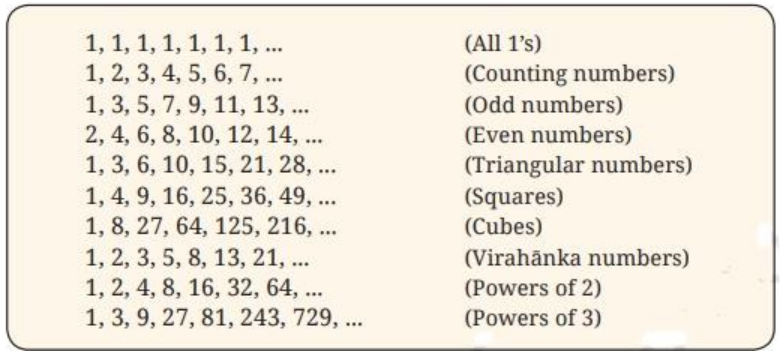
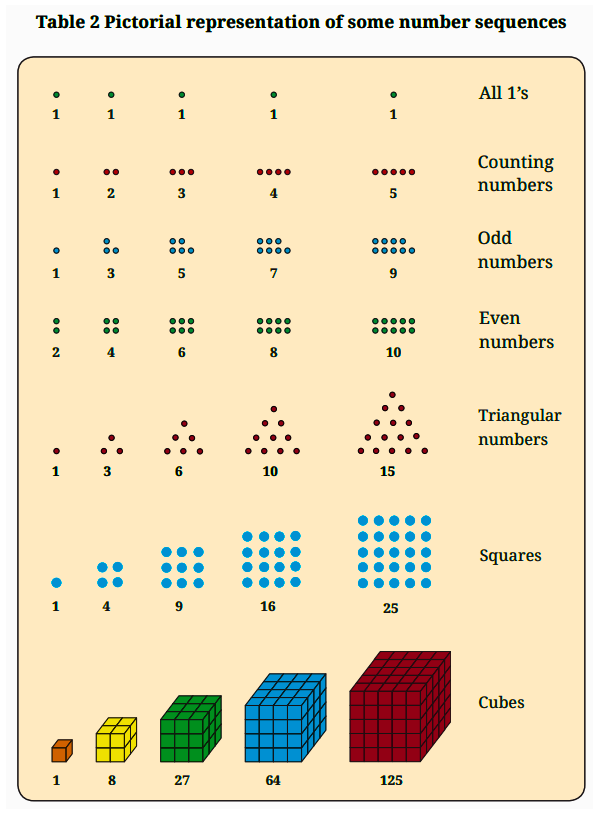
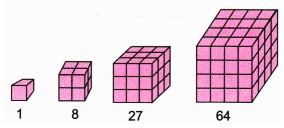
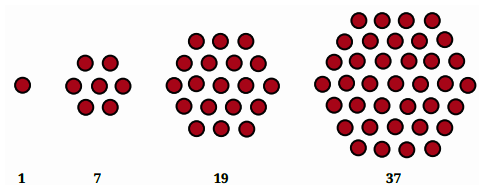
Chapter 1 covers various topics such as patterns in numbers, visualising number sequences, relations among number sequences, patterns in shapes, and their connection to number sequences. These topics help students understand different types of mathematical patterns and their applications.
2. How can NCERT Solutions help with Exercise 1.1?
NCERT Solutions for Exercise 1.1 provides clear explanations about the concept of mathematics and its relevance. They help students understand basic mathematical ideas and their real-life applications, setting a strong foundation for further study.
3. What is the focus of Exercise 1.2 in Chapter 1?
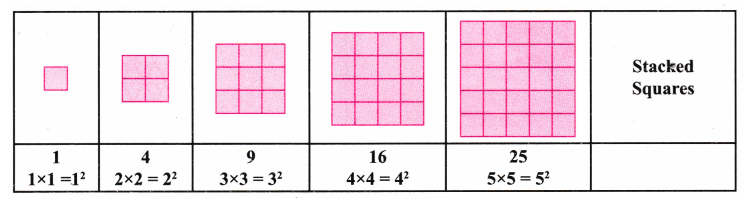
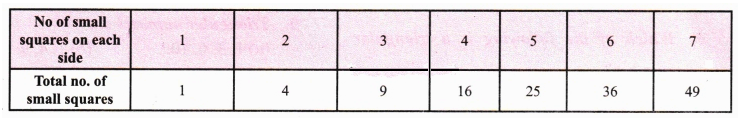
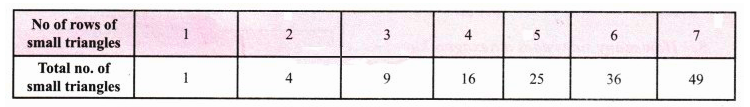
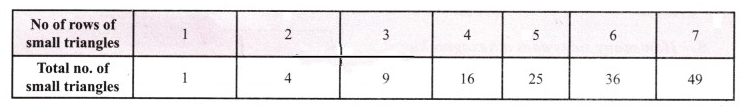
Exercise 1.2 focuses on identifying and analysing patterns in numbers. It teaches students how to recognize number sequences and understand the rules that govern these patterns, which is essential for solving related problems.
4. How does Exercise 1.3 help students?
Exercise 1.3 helps students visualize and interpret number sequences. By practising this exercise, students learn to understand how sequences progress and predict future numbers, enhancing their numerical reasoning skills.
5. What does Exercise 1.4 cover?
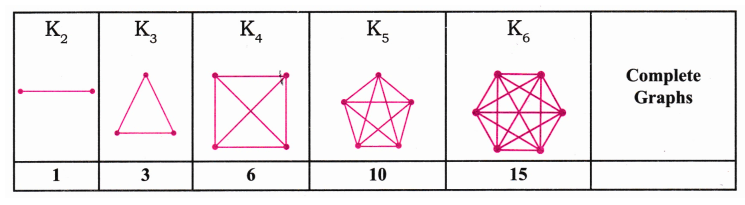
Exercise 1.4 explores the relationships between different number sequences. It helps students analyse how sequences are related to each other and understand the connections, which aids in solving more complex pattern problems.
6. What is the aim of Exercise 1.5?
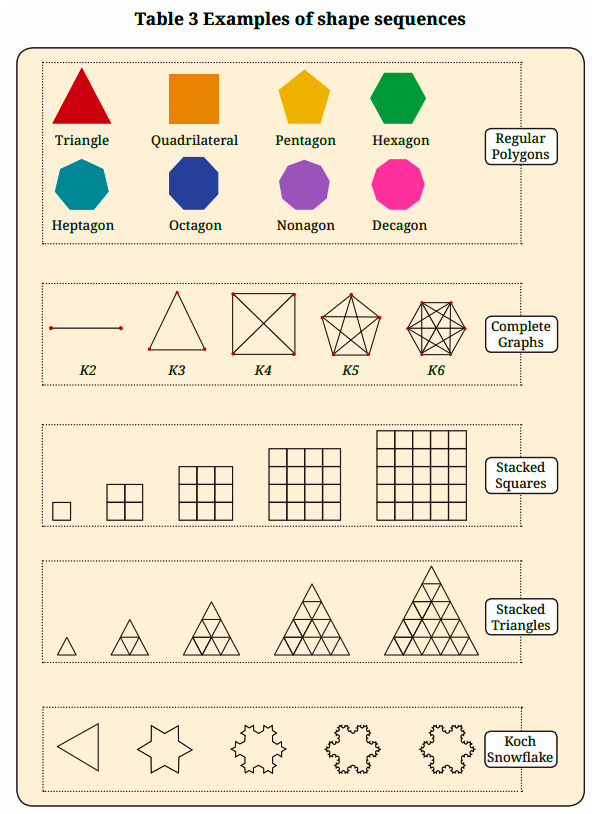
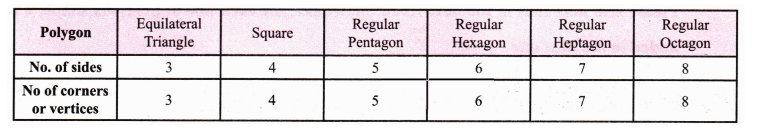
Exercise 1.5 focuses on recognizing and creating patterns using geometric shapes. It helps students develop spatial reasoning skills and understand how different shapes can form patterns, which is crucial for visual learning.
7. How does Exercise 1.6 relate to number sequences?
Exercise 1.6 shows how number sequences can be related to geometric patterns. It reinforces the understanding of how numerical and geometric patterns connect, helping students apply these concepts effectively.
8. How can NCERT Solutions improve my skills in this chapter?
NCERT Solutions provides step-by-step guidance and clear explanations, making it easier to understand and solve problems. Practising these solutions helps improve skills in recognizing and working with different types of patterns.
9. Are the solutions available in a downloadable format?
Yes, the solutions for Chapter 1 are available as a FREE PDF download. This format allows students to study and practice at their own pace, making it easy to review and reinforce their understanding.
10. How do the solutions align with the CBSE syllabus?
The NCERT Solutions for Class 6 Maths Chapter 1 aligns with the CBSE syllabus by covering all required topics and exercises. They follow the curriculum guidelines, ensuring that students meet the learning objectives effectively.
11. Can these solutions help with exam preparation?
Yes, the solutions offer detailed explanations and practice problems that can help in exam preparation. They help students understand key concepts and improve their problem-solving skills for better performance in exams.
12. Are there any tips included in the solutions?
The solutions include useful tips and methods for solving different types of problems. These tips help students approach questions more effectively and develop a clearer understanding of mathematical patterns.
13. What if I need more help with specific problems?
If you need more help with specific problems, you can use the detailed explanations provided in the solutions. For additional support, consider discussing your questions with a teacher or using supplementary study materials.