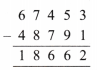
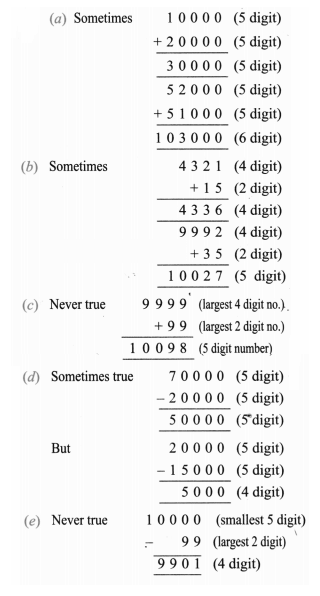
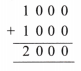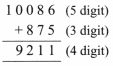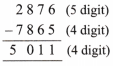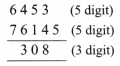Class 6 Maths NCERT Solutions for Chapter 3 Exercise 3.8 FREE PDF Download
Chapter 3 Number Play Class 6 Maths syllabus, introduces students to different concepts related to numbers such as factors, multiples, prime and composite numbers, odd and even numbers, and more. Exercise 3.8 focuses on Mental Math which helps in sharpening problem-solving skills and improving speed in calculations without the use of pen and paper. This chapter is crucial for building a strong foundation in understanding numbers, which is important for more advanced topics in mathematics.
- 3.1Exercise 3.8
Our Class 6 Maths NCERT Solutions PDF breaks the lesson into easy-to-understand explanations, making learning fun and interactive. Students will develop essential language skills with engaging activities and exercises. Check out the revised CBSE Class 6 Maths syllabus and start practising the Maths Class 6 Chapter 3.
Glance on Class 6 Chapter 3 Number Play Exercise 3.8 Number Play
Understanding of number patterns.
Introduction to prime and composite numbers.
Divisibility rules for numbers.
Concepts of factors and multiples.
Mental math techniques for quick calculations.
Problem-solving related to odd and even numbers.
Co-prime numbers and perfect numbers.
Exercise 3.8
Question 1. Write an example for each of the below scenarios whenever possible.
Could you find examples for all the cases? If not, think and discuss what could be the reason. Make other such questions and challenge your classmates.
(a) Let’s divide
90, 250 by 2
Hence to get sum more than 90,250 both numbers should be more than 45,125.
(b) To get a 6 digit sum by adding 5 digit and 3 digit, the 5 digit number should be more than 99,001.
(c) Let’s take minimum 4 digit number 1000
let’s add them
which is a 4 digit number.
Hence 6 digit sum’ from 4 digit number is impossible.
(d) Let’s take 5 digit numbers 67987 and 65783
let’s add them
which is a 6 digit number.
(e) Let’s take minimum 5 digit numbers 1000
let’s add them
which is a 5 digit number.
Hence 6 digit sum from 5 digit numbers is impossible.
(f) 5-digit -5 digit to
give a difference less than 56,503
< 56503
(g) 5-digit-3 digit = 1 008 6 (5 digit) to give a 4 digit = + 875 (3 digit)
difference = 92 11 (4 digit)
(h) 5-digit digit = 1 2 8 7 6 (5 digit) to give a 4 digit = -7865 (4 digit)
difference = 5 0 11 (4 digit)
(i) 5-digit -5 digit 7 = 645 3 (5 digit) to give a 3 digit = 76 145 (5 digit)
difference = 308 (3 digit)
(j) 5-digit -5 digit
Not possible to give 91,500
Question 2. Always, Sometimes, Never?
Below are some statements. Think, explore and find out if each of the statement is ‘Always true’, ‘Only sometimes true’ or ‘Never true’. Why do you think so? Write your reasoning; discuss this with the class.
(a) 5-digit number + 5-digit number gives a 5-digit number
(b) 4-digit number + 2-digit number gives a 4-digit number
(c) 4-digit number + 2-digit number gives a 6-digit number
(d) 5-digit number – 5-digit number gives a 5-digit number
(e) 5-digit number – 2-digit number gives a 3-digit number
Solution:
Benefits of NCERT Solutions for Class 6 Maths Chapter 3 Exercise 3.8
Enhances problem-solving speed through mental math exercises.
Develops a strong foundation in number theory.
Improves understanding of number properties such as divisibility and multiples.
Strengthens logical reasoning by exploring number patterns and sequences.
Prepares students for advanced topics in mathematics.
Encourages independent thinking and mental calculations.
Class 6 Maths Chapter 3: Exercises Breakdown
Important Study Material Links for Maths Chapter 3 Class 6
Conclusion
Chapter 3: Number Play is an essential part of the Class 6 Maths curriculum that fosters an understanding of numbers and their properties. Exercise 3.8, focused on Mental Math, plays a key role in boosting mental agility and calculation speed. By mastering this chapter, students can improve their mathematical fluency and problem-solving skills, which will benefit them in both academic and real-life situations.
Chapter-wise NCERT Solutions Class 6 Maths
After familiarising yourself with the Class 6 Maths Chapters Question Answers, you can access comprehensive NCERT Solutions from all Class 6 Maths textbook chapters.
Related Important Links for Class 6 Maths
Along with this, students can also download additional study materials provided by Vedantu for Maths Class 6-
FAQs on NCERT Solutions for Class 6 Maths Chapter 3 Number Play Ex 3.8
1. What is the importance of Mental Math as introduced in Chapter 3: Number Play?
Mental Math improves calculation speed, sharpens problem-solving skills, and helps perform quick arithmetic operations without using pen and paper.
2. How does Chapter 3: Number Play help in understanding prime and composite numbers?
The chapter explains how prime numbers have only two factors (1 and the number itself), while composite numbers have more than two factors.
3. What are co-prime numbers as explained in NCERT Solutions for Chapter 3: Number Play?
Co-prime numbers are two numbers that share only 1 as a common factor. For example, 9 and 28 are co-prime.
4. What is the role of divisibility rules in Mental Math as per Chapter 3: Number Play?
Divisibility rules help quickly determine whether a number can be divided by another number without remainder, making calculations faster.
5. How does Exercise 3.8 help students in developing problem-solving skills?
Exercise 3.8 uses mental math techniques that challenge students to solve problems quickly, enhancing their arithmetic and reasoning skills.
6. What is the difference between factors and multiples as explained in Chapter 3: Number Play?
A factor is a number that divides another number exactly, while a multiple is the result of multiplying a number by an integer.
7. How can understanding number patterns be beneficial in Mental Math?
Recognizing number patterns helps in predicting future numbers and solving sequences, which enhances problem-solving efficiency.
8. What are perfect numbers according to Chapter 3: Number Play?
A perfect number is a number that equals the sum of its proper divisors. For example, 6 is a perfect number because 1 + 2 + 3 = 6.
9. What is the significance of the Greatest Common Divisor (GCD) in Mental Math?
GCD helps find the largest number that divides two or more numbers exactly, which is useful for simplifying fractions and solving problems quickly.
10. How does mastering Exercise 3.8 contribute to overall mathematical fluency?
It enhances the ability to perform calculations in the mind, improves logical thinking, and prepares students for more complex mathematical concepts.
