NCERT Solutions for Class 7 Maths Chapter 11 Exponents And Powers Ex 11.2

Exercise 11.2

1. Using laws of exponents, simplify and write the answer in exponential form:

i) ${3^2} \times {3^4} \times {3^8}$

Ans: The given expression is: ${3^2} \times {3^4} \times {3^8}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write the given expression as,

${3^2} \times {3^4} \times {3^8} = {3^{\left( {2 + 4 + 8} \right)}}$

${3^2} \times {3^4} \times {3^8} = {3^{14}}$

Hence, the required answer is ${3^2} \times {3^4} \times {3^8} = {3^{14}}$.

ii) ${6^{15}} \div {6^{10}}$

Ans: The given expression is: ${6^{15}} \div {6^{10}}$

By laws of exponents, we have ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write the given expression as,

${6^{15}} \div {6^{10}} = {6^{\left( {15 - 10} \right)}}$

${6^{15}} \div {6^{10}} = {6^5}$

Hence, the required answer is ${6^{15}} \div {6^{10}} = {6^5}$.

iii) ${a^3} \times {a^2}$

Ans: The given expression is: ${a^3} \times {a^2}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write the given expression as,

${a^3} \times {a^2} = {a^{\left( {3 + 2} \right)}}$

${a^3} \times {a^2} = {a^5}$

Hence, the required answer is ${a^3} \times {a^2} = {a^5}$.

iv) ${7^x} \times {7^2}$

Ans: The given expression is: ${7^x} \times {7^2}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write the given expression as,

${7^x} \times {7^2} = {7^{x + 2}}$

Hence, the required answer is ${7^x} \times {7^2} = {7^{x + 2}}$.

v) ${\left( {{5^2}} \right)^3} \div {5^3}$

Ans: The given expression is: ${\left( {{5^2}} \right)^3} \div {5^3}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write the given expression as,

${\left( {{5^2}} \right)^3} \div {5^3} = {5^{\left( {2 \times 3} \right)}} \div {5^3}$

${\left( {{5^2}} \right)^3} \div {5^3} = {5^6} \div {5^3}$

By laws of exponents, we also have ${a^m} \div {a^n} = {a^{m - n}}$

${\left( {{5^2}} \right)^3} \div {5^3} = {5^{6 - 3}}$

${\left( {{5^2}} \right)^3} \div {5^3} = {5^3}$

Hence, the required answer is ${\left( {{5^2}} \right)^3} \div {5^3} = {5^3}$

vi) ${2^5} \times {5^5}$

Ans: The given expression is: ${2^5} \times {5^5}$

By laws of exponents, we have ${a^m} \times {b^m} = {\left( {a \times b} \right)^m}$.

So, we can write the given expression as,

${2^5} \times {5^5} = {\left( {2 \times 5} \right)^5}$

${2^5} \times {5^5} = {10^5}$

Hence, the required answer is ${2^5} \times {5^5} = {10^5}$.

vii) ${a^4} \times {b^4}$

Ans: The given expression is: ${a^4} \times {b^4}$

By laws of exponents, we have ${a^m} \times {b^m} = {\left( {a \times b} \right)^m}$ .

So, we can write the given expression as,

${a^4} \times {b^4} = {\left( {a \times b} \right)^4}$

Hence, the required answer is ${a^4} \times {b^4} = {\left( {a \times b} \right)^4}$ .

viii) ${\left( {{3^4}} \right)^3}$

Ans: The given expression is: ${\left( {{3^4}} \right)^3}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write the given expression as,

${\left( {{3^4}} \right)^3} = {3^{\left( {4 \times 3} \right)}}$

${\left( {{3^4}} \right)^3} = {3^{12}}$

Hence, the required answer is ${\left( {{3^4}} \right)^3} = {3^{12}}$.

ix) $\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3}$

Ans: The given expression is: $\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3}$

By laws of exponents, we also have ${a^m} \div {a^n} = {a^{m - n}}$

$\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3} = {2^{\left( {20 - 15} \right)}} \times {2^3}$

$\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3} = {2^5} \times {2^3}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write 

$\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3} = {2^{5 + 3}}$

$\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3} = {2^8}$

Hence, the required answer is $\left( {{2^{20}} \div {2^{15}}} \right) \times {2^3} = {2^8}$.

x) ${8^t} \div {8^2}$

Ans: The given expression is: ${8^t} \div {8^2}$

By laws of exponents, we have ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write the given expression as,

${8^t} \div {8^2} = {8^{t - 2}}$

Hence, the required answer is ${8^t} \div {8^2} = {8^{t - 2}}$.

2. Simplify and express each of the following in exponential form:

i) $\dfrac{{{2^3} \times {3^4} \times 4}}{{3 \times 32}}$

Ans: The given expression is $\dfrac{{{2^3} \times {3^4} \times 4}}{{3 \times 32}}$

$32$can be written as,

$32 = {2^5}$

Also $4$ can be written as,

$4 = {2^2}$

Then the given expression becomes,

$\dfrac{{{2^3} \times {3^4} \times 4}}{{3 \times 32}} = \dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = \dfrac{{{2^{3 + 2}} \times {3^4}}}{{3 \times {2^5}}}$

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = \dfrac{{{2^5} \times {3^4}}}{{3 \times {2^5}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = {2^{5 - 5}} \times {3^{4 - 1}}$

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = {2^0} \times {3^3}$

We have ${a^0} = 1$

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = 1 \times {3^3}$

$\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = {3^3}$

Hence, the required solution is $\dfrac{{{2^3} \times {3^4} \times {2^2}}}{{3 \times {2^5}}} = {3^3}$.

ii) $\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7}$

Ans: The given expression is $\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write the given expression as

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = \left[ {{5^{2 \times 3}} \times {5^4}} \right] \div {5^7}$

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = \left[ {{5^6} \times {5^4}} \right] \div {5^7}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = {5^{6 + 4}} \div {5^7}$

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = {5^{10}} \div {5^7}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = {5^{10 - 7}}$

$\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = {5^3}$

Hence, the required solution is $\left[ {{{\left( {{5^2}} \right)}^3} \times {5^4}} \right] \div {5^7} = {5^3}$.

iii) ${25^4} \div {5^3}$

Ans: The given expression is ${25^4} \div {5^3}$

$25$can be written as

$25 = {5^2}$

So, the expression will be

${25^4} \div {5^3} = {\left( {{5^2}} \right)^4} \div {5^3}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write 

${25^4} \div {5^3} = {5^{2 \times 4}} \div {5^3}$

${25^4} \div {5^3} = {5^8} \div {5^3}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

${25^4} \div {5^3} = {5^{8 - 3}}$

${25^4} \div {5^3} = {5^5}$

Hence, the required solution is ${25^4} \div {5^3} = {5^5}$.

iv) $\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}}$

Ans: The given expression is $\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}}$

$21$can be written as,

$21 = 7 \times 3$

Then the given expression becomes,

$\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = \dfrac{{3 \times {7^2} \times {{11}^8}}}{{7 \times 3 \times {{11}^3}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = {3^{1 - 1}} \times {7^{2 - 1}} \times {11^{8 - 3}}$

$\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = {3^0} \times {7^1} \times {11^5}$

We have ${a^0} = 1$

$\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = 1 \times 7 \times {11^5}$

$\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = 7 \times {11^5}$

Hence, the required solution is $\dfrac{{3 \times {7^2} \times {{11}^8}}}{{21 \times {{11}^3}}} = 7 \times {11^5}$.

v) $\dfrac{{{3^7}}}{{{3^4} \times {3^3}}}$

Ans: The given expression is $\dfrac{{{3^7}}}{{{3^4} \times {3^3}}}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = \dfrac{{{3^7}}}{{{3^{4 + 3}}}}$

$\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = \dfrac{{{3^7}}}{{{3^7}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = {3^{7 - 7}}$

$\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = {3^0}$

We have ${a^0} = 1$

$\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = 1$

Hence, the required solution is $\dfrac{{{3^7}}}{{{3^4} \times {3^3}}} = 1$.

vi) ${2^0} + {3^0} + {4^0}$

Ans: The given expression is ${2^0} + {3^0} + {4^0}$ .

We have ${a^0} = 1$

${2^0} + {3^0} + {4^0} = 1 + 1 + 1$

By simplify,

${2^0} + {3^0} + {4^0} = 3$

Hence, the required solution is${2^0} + {3^0} + {4^0} = 3$.

vii) ${2^0} \times {3^0} \times {4^0}$

Ans: The given expression is ${2^0} \times {3^0} \times {4^0}$ .

We have ${a^0} = 1$

${2^0} \times {3^0} \times {4^0} = 1 \times 1 \times 1$

By simplify,

${2^0} \times {3^0} \times {4^0} = 1$

Hence, the required solution is${2^0} \times {3^0} \times {4^0} = 1$.

viii) $\left( {{3^0} + {2^0}} \right) \times {5^0}$

Ans: The given expression is $\left( {{3^0} + {2^0}} \right) \times {5^0}$ .

We have ${a^0} = 1$

$\left( {{3^0} + {2^0}} \right) \times {5^0} = \left( {1 + 1} \right) \times 1$

By simplify,

$\left( {{3^0} + {2^0}} \right) \times {5^0} = 2 \times 1$

$\left( {{3^0} + {2^0}} \right) \times {5^0} = 2$

Hence, the required solution is$\left( {{3^0} + {2^0}} \right) \times {5^0} = 2$.

ix) $\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}}$

Ans: The given expression is $\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}}$

$4$can be written as,

$4 = {2^2}$

Then the given expression becomes,

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = \dfrac{{{2^8} \times {a^5}}}{{{{\left( {{2^2}} \right)}^3} \times {a^3}}}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = \dfrac{{{2^8} \times {a^5}}}{{{2^{\left( {2 \times 3} \right)}} \times {a^3}}}$

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = \dfrac{{{2^8} \times {a^5}}}{{{2^6} \times {a^3}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = {2^{8 - 6}} \times {a^{5 - 3}}$

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = {2^2} \times {a^2}$

By laws of exponents, we have ${a^m} \times {b^m} = {\left( {a \times b} \right)^m}$ .

So, we can write

$\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = {\left( {2a} \right)^2}$

Hence, the required solution is $\dfrac{{{2^8} \times {a^5}}}{{{4^3} \times {a^3}}} = {\left( {2a} \right)^2}$.

x) $\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8}$

Ans: The given expression is $\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8} = {a^{5 - 3}} \times {a^8}$

$\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8} = {a^2} \times {a^8}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8} = {a^{2 + 8}}$

$\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8} = {a^{10}}$

Hence, the required solution is $\left( {\dfrac{{{a^5}}}{{{a^3}}}} \right) \times {a^8} = {a^{10}}$.

xi) $\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}}$

Ans: The given expression is $\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}} = {4^{5 - 5}} \times {a^{8 - 5}} \times {b^{3 - 2}}$

$\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}} = {4^0} \times {a^3} \times {b^1}$

We have ${a^0} = 1$

$\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}} = 1 \times {a^3} \times b$

By simplifying, 

$\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}} = {a^3}b$

Hence, the required solution is $\dfrac{{{4^5} \times {a^8}{b^3}}}{{{4^5} \times {a^5}{b^2}}} = {a^3}b$.

xii) ${\left( {{2^3} \times 2} \right)^2}$

Ans: The given expression is ${\left( {{2^3} \times 2} \right)^2}$

By laws of exponents, we have ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

${\left( {{2^3} \times 2} \right)^2} = {\left( {{2^{3 + 1}}} \right)^2}$

${\left( {{2^3} \times 2} \right)^2} = {\left( {{2^4}} \right)^2}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write

${\left( {{2^3} \times 2} \right)^2} = {2^{4 \times 2}}$

${\left( {{2^3} \times 2} \right)^2} = {2^8}$

Hence, the required solution is ${\left( {{2^3} \times 2} \right)^2} = {2^8}$.

3. Say true or false and justify your answer:

i) $10 \times {10^{11}} = {100^{11}}$

Ans: The given expression is $10 \times {10^{11}} = {100^{11}}$

Taking left hand side,

By laws of exponents ${a^m} \times {a^n} = {a^{m + n}}$  we can write,

$10 \times {10^{11}} = {10^{1 + 11}}$

$10 \times {10^{11}} = {10^{12}}$

Taking right hand side,

${100^{11}} = {\left( {{{10}^2}} \right)^{11}}$

${100^{11}} = {10^{2 \times 11}}$

${100^{11}} = {10^{22}}$

So, ${\text{L}}{\text{.H}}{\text{.S}} \ne {\text{R}}{\text{.H}}{\text{.S}}$

Hence, the given statement is false.

ii) ${2^3} > {5^2}$

Ans: The given expression is ${2^3} > {5^2}$

Taking left hand side,

${2^3} = 8$

Taking right hand side,

${5^2} = 25$

Since, $25 > 8$

So, ${5^2} > {2^3}$

Hence, the given statement is false.

iii) ${2^3} \times {3^2} = {6^5}$

Ans: The given expression is ${2^3} \times {3^2} = {6^5}$

Taking left hand side,

${2^3} \times {3^2} = 8 \times 9$

${2^3} \times {3^2} = 72$

Taking right hand side,

${6^5} = 7776$

So,${2^3} \times {3^2} \ne {6^5}$

Hence, the given statement is false.

iv) ${3^0} = {\left( {1000} \right)^0}$

Ans: The given expression is ${3^0} = {\left( {1000} \right)^0}$

Taking left hand side,

Since, ${a^0} = 1$

${3^0} = 1$

Taking right hand side,

Since, ${a^0} = 1$

${\left( {1000} \right)^0} = 1$

So,${3^0} = {\left( {1000} \right)^0}$

Hence, the given statement is true.

4. Express each of the following as a product of prime factors only in exponential form:

i) $108 \times 192$

Ans: The given expression is $108 \times 192$

Finding prime factors of$108$,

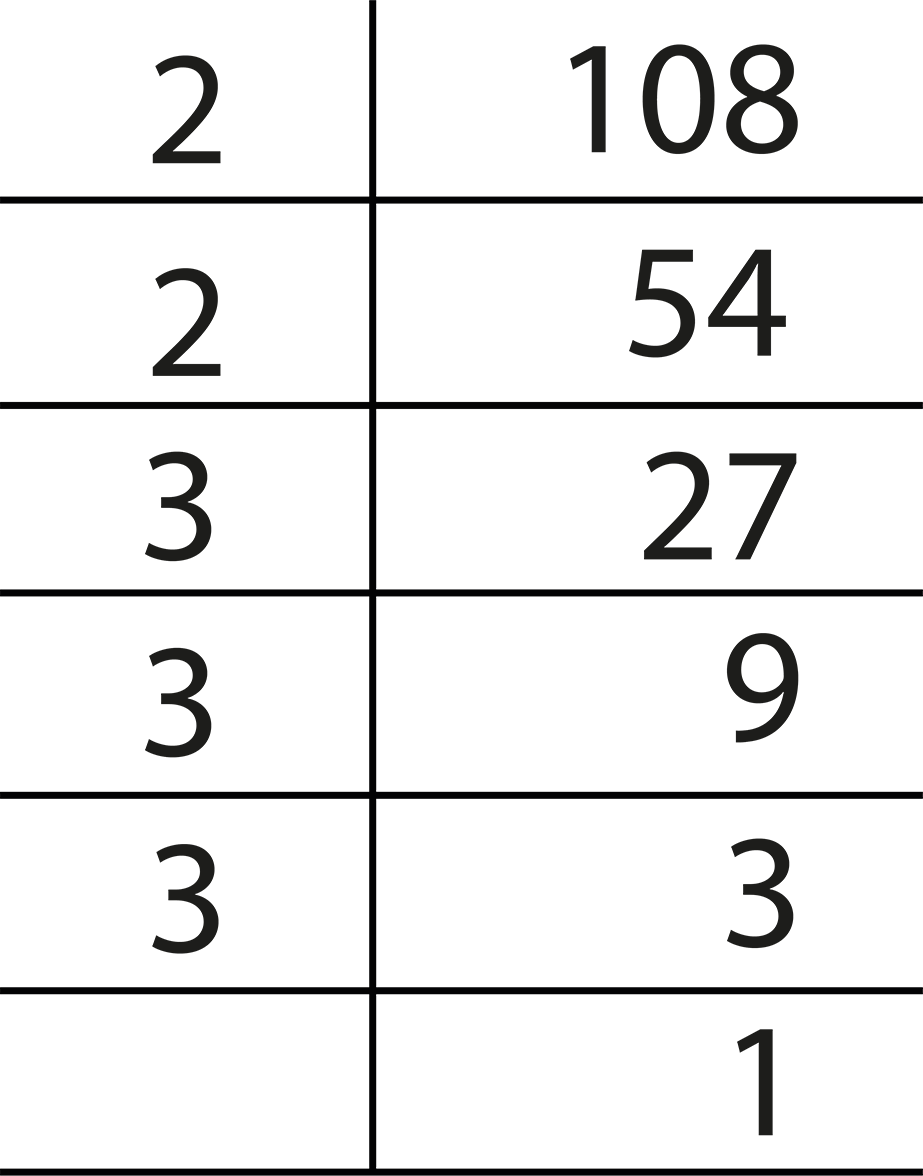
So, $108$can be express as

$108 = 2 \times 2 \times 3 \times 3 \times 3$

$108 = {2^2} \times {3^3}$

Finding prime factors of$192$,

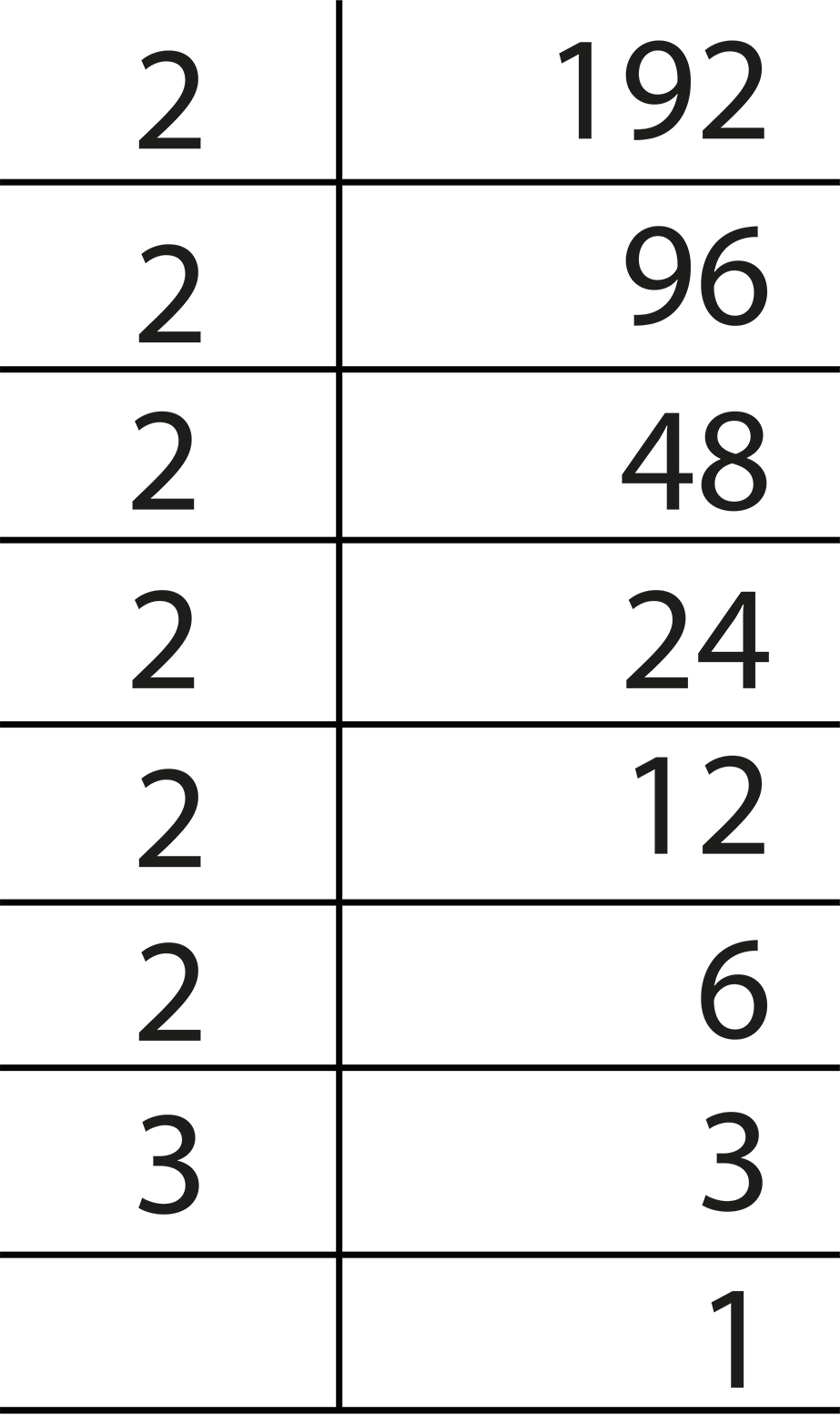
So, $192$can be express as

$192 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3$

$192 = {2^6} \times 3$

So, $108 \times 192 = \left( {{2^2} \times {3^3}} \right) \times \left( {{2^6} \times 3} \right)$

$108 \times 192 = \left( {{2^2} \times {3^3}} \right) \times \left( {{2^6} \times 3} \right)$

Since, ${a^m} \times {a^n} = {a^{m + n}}$

$108 \times 192 = {2^{2 + 6}} \times {3^{3 + 1}}$

$108 \times 192 = {2^8} \times {3^4}$

Hence, the required solution is $108 \times 192 = {2^8} \times {3^4}$.

ii) $270$

Ans: The given expression is $270$

Finding prime factors of$270$,

So, $270$can be express as

$270 = 2 \times 3 \times 3 \times 3 \times 5$

$270 = 2 \times {3^3} \times 5$

Hence, the required solution is $270 = 2 \times {3^3} \times 5$.

iii) $729 \times 64$

Ans: The given expression is $729 \times 64$

Finding prime factors of$729$,

So, $729$can be express as

$729 = 3 \times 3 \times 3 \times 3 \times 3 \times 3$

$729 = {3^6}$

Finding prime factors of$64$ ,

So, $64$can be express as

$64 = 2 \times 2 \times 2 \times 2 \times 2 \times 2$

$64 = {2^6}$

So, $729 \times 64 = {3^6} \times {2^6}$

Hence, the required solution is $729 \times 64 = {3^6} \times {2^6}$ .

iv) $768$

Ans: The given expression is $768$

Finding prime factors of$768$,

So, $768$can be express as

$768 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3$

$768 = {2^8} \times 3$

Hence, the required solution is $768 = {2^8} \times 3$.

5. Simplify:

i) $\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}}$

Ans: The given expression is $\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}}$

$8$can be written as,

$8 = {2^3}$

Then the given expression becomes,

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = \dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{{\left( {{2^3}} \right)}^3} \times 7}}$

By laws of exponents, we have ${\left( {{a^m}} \right)^n} = {a^{m \times n}}$

So, we can write

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = \dfrac{{{2^{\left( {5 \times 2} \right)}} \times {7^3}}}{{{2^{\left( {3 \times 3} \right)}} \times 7}}$

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = \dfrac{{{2^{10}} \times {7^3}}}{{{2^9} \times 7}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = {2^{10 - 9}} \times {7^{3 - 1}}$

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = {2^1} \times {7^2}$

Since, ${7^2} = 49$

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = 2 \times 49$

$\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = 98$

Hence, the required solution is $\dfrac{{{{\left( {{2^5}} \right)}^2} \times {7^3}}}{{{8^3} \times 7}} = 98$.

ii) $\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}}$

Ans:  The given expression is $\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}}$

$25$can be written as,

$25 = {5^2}$

$10$can be express as,

$10 = 2 \times 5$

Then the given expression becomes,

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^2} \times {5^2} \times {t^8}}}{{{{\left( {2 \times 5} \right)}^3} \times {t^4}}}$

By laws of exponents, we have ${\left( {a \times b} \right)^m} = {a^m} \times {b^m}$

So, we can write

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^2} \times {5^2} \times {t^8}}}{{{2^3} \times {5^3} \times {t^4}}}$

By laws of exponents, we have  ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^{2 + 2}} \times {t^8}}}{{{2^3} \times {5^3} \times {t^4}}}$

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^4} \times {t^8}}}{{{2^3} \times {5^3} \times {t^4}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^{4 - 3}} \times {t^{8 - 4}}}}{{{2^3}}}$

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{{5^1} \times {t^4}}}{{{2^3}}}$

Since, ${2^3} = 8$

$\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{5{t^4}}}{8}$

Hence, the required solution is $\dfrac{{25 \times {5^2} \times {t^8}}}{{{{10}^3} \times {t^4}}} = \dfrac{{5{t^4}}}{8}$.

iii) $\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}}$

Ans:  The given expression is $\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}}$

$25$can be written as,

$25 = {5^2}$

$10$can be express as,

$10 = 2 \times 5$

$6$can be express as,

$6 = 2 \times 3$

Then the given expression becomes,

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = \dfrac{{{3^5} \times {{\left( {2 \times 5} \right)}^5} \times {5^2}}}{{{5^7} \times {{\left( {2 \times 3} \right)}^5}}}$

By laws of exponents, we have ${\left( {a \times b} \right)^m} = {a^m} \times {b^m}$

So, we can write

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = \dfrac{{{3^5} \times {2^5} \times {5^5} \times {5^2}}}{{{5^7} \times {2^5} \times {3^5}}}$

By laws of exponents, we have  ${a^m} \times {a^n} = {a^{m + n}}$ .

So, we can write

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = \dfrac{{{3^5} \times {2^5} \times {5^{5 + 2}}}}{{{5^7} \times {2^5} \times {3^5}}}$

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = \dfrac{{{3^5} \times {2^5} \times {5^7}}}{{{5^7} \times {2^5} \times {3^5}}}$

By laws of exponents, we have  ${a^m} \div {a^n} = {a^{m - n}}$ .

So, we can write

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = {3^{5 - 5}} \times {2^{5 - 5}} \times {5^{7 - 7}}$

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = {3^0} \times {2^0} \times {5^0}$

Since, ${a^0} = 1$

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = 1 \times 1 \times 1$

$\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = 1$

Hence, the required solution is $\dfrac{{{3^5} \times {{10}^5} \times 25}}{{{5^7} \times {6^5}}} = 1$.

Conclusion

In Maths Class 7 Chapter 11 Exercise 11.2 Exponents and Powers, we have explored and applied the fundamental laws of exponents to various problems. By understanding the product of powers, quotient of powers, power of a power, power of a product, and power of a quotient rules, students can simplify and solve exponential expressions easily. Class 7 Maths Chapter 11 Exercise 11.2 Solutions Exponents and Powers has provided a solid foundation for working with exponents, essential for higher-level mathematics. The examples and problems presented have demonstrated the practical applications of these rules, ensuring that students can confidently handle exponent-related questions in their future studies.

Class 7 Maths Chapter 11: Exercises Breakdown

CBSE Class 7 Maths Chapter 11 Other Study Materials

Chapter-Specific NCERT Solutions for Class 7 Maths

Given below are the chapter-wise NCERT Solutions for Class 7 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.

Important Related Links for NCERT Class 7 Maths

Access these essential links for NCERT Class 7 Maths, offering comprehensive solutions, study guides, and additional resources to help students master language concepts and excel in their exams.