NCERT Solutions for Class 7 Maths Lines and Angles Chapter 5 - FREE PDF Download






FAQs on NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles
1. What are the main contents of Class 7 Maths Chapter 5 Lines and Angles?
The main topics that are included in the fifth chapter of Class 7 Maths Lines and Angles are:
Introduction
Related Angles
Complementary Angles
Supplementary Angles
Adjacent Angles
Linear Pair
Vertically Opposite Angles
Pairs of Lines
Intersecting Lines
Transversal
Angles made by a Transversal
Transversal of Parallel Lines
Checking for Parallel Lines
Students can find the solutions to the NCERT textbook questions related to these topics on Vedantu’s site. This will help students to clear all their doubts regarding the chapter.
2. How are NCERT Solutions for Class 7 Maths Chapter 5 useful for students?
NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles is the best study material for practising and revising the chapter. Especially for those students who are finding difficulties in solving problems, these solutions by the expert teachers are immensely helpful. NCERT Solutions for Class 7 Maths Chapter 5 is really helpful to understand the chapter and clear the doubts quickly. Students can score well in the exam by referring to NCERT Solutions for Class 7 Maths available in the free PDF format.
3. Where can I find good quality NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles?
There are many online platforms available that cater to chapter-wise NCERT Solution for Class 7 Mathematics. However, it is crucial to download the same from the authentic site which offers the complete coverage of the exercise questions. Vedantu is one such site where one can find good quality NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles. These solutions include stepwise explanations of the exercise problems. Also, these are available for free download, in high-resolution PDF quality. Hence, students need not struggle in finding the solutions. They can instantly clear their doubts regarding the chapter with the help of Vedantu’s NCERT Solutions for Class 7 Maths Chapter 5.
4. What are the key features of Vedantu’s NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles?
Following are some of the key features of NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles provided by Vedantu:
Easy to Access: NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles are available in the free to download PDF form.
Created by Expert Tutors: These solutions are created by experts. Hence, these are 100% verified.
Complete Syllabus Coverage: NCERT Solutions for Class 7 Maths for chapter 5 as well as ad other chapters provide complete coverage of the exercise questions.
As per the Board Guidelines: These solutions are prepared at Vedantu as per the CBSE board guidance and the latest exam pattern.
5. What are the Important Topics Covered in NCERT Solutions Class 7 Maths Chapter 5?
The most important topics covered in NCERT Solutions Class 7 Maths Chapter 5 are Relative Angles And Their Properties, Complementary Angles, Supplementary Angles, Adjacent Angles, Linear Pairs, Vertically Opposite Angles, Pair Of Lines, Intersecting Lines, Transversal, The Angle Made By A Transversal, A Transversal Of Parallel Lines And Checking For Parallel Lines. These topics are explained thoroughly with their respective properties in great detail that are used to solve various questions in this chapter.
6. How Many Questions are there in NCERT Solutions Class 7 Maths Chapter 5 Lines and Angles?
There are two exercises in NCERT Solutions Class 7 Maths Chapter 5 Lines and Angles. Exercise 5.1 has 14 questions with most of its questions having multiple subparts. Exercise 5.2 has six questions with its questions having sub-parts as well. These questions revolve around the basic topics of the chapter Lines and Angles and to solve them, one requires the knowledge of all the concepts that are given in the chapter. To check the solutions, students can download the NCERT Solutions free of cost from Vedantu platform.
7. Mention the topics that are covered in NCERT Solutions for Class 7 Maths Chapter 5?
Relative Angles And Their Properties, Complementary Angles, Supplementary Angles, Adjacent Angles, Linear Pairs, Vertically Opposite Angles, Pair Of Lines, Intersecting Lines, Transversal, The Angle Made By A Transversal, A Transversal Of Parallel Lines, And Checking For Parallel Lines are some of the important topics covered in NCERT Solutions Class 7 Maths Chapter 5. All the solutions to these topics are easily available on Vedantu website and the app.
8. How is NCERT Solution for Class 7 Maths Chapter 5 helpful for board exams?
The NCERT Solution for Class 7 Maths Chapter 5 is very helpful for board exams as all the questions are answered with utmost precision with all the formulae written along to make it convenient for the students to revise along. These questions set the base for board examination as they are very concept based questions and hence have to be answered tactfully. The NCERT Solutions will not only help the students understand the answers better, but they will also help them remember the key concepts.
9. How to score full marks in Class 7 Maths Chapter 5?
To score full marks in any domain of Maths, you must do only one thing - practice. Practice is not only the key to understanding all the concepts on a deeper level, but it will also help you avoid silly mistakes. You will also get to know the kind of answer the question demands and will be able to frame your answers better.
10. What are complementary angles in lines and angles class 7 solutions?
In lines and angles class 7 solutions complementary angles are two angles whose measures add up to 90 degrees. For example, if one angle measures 30 degrees, the other must measure 60 degrees to be complementary. This concept is crucial in understanding various geometric shapes and solving problems involving right angles.
11. How do you identify parallel lines in lines and angles class 7?
In lines and angles class 7 Parallel lines are lines in a plane that do not intersect, no matter how far they are extended. They are always the same distance apart. A common way to identify parallel lines is by looking for the same slope or by using corresponding angles formed by a transversal line.
12. What is the significance of the transversal in geometry in lines and angles class 7 ?
In lines and angles class 7, a transversal is a line that intersects two or more other lines at different points. It plays a significant role in geometry as it helps in identifying angle relationships, such as corresponding angles, alternate interior angles, and alternate exterior angles, which are key in solving various geometric problems.

















![Complement of \[20{}^\circ = 90{}^\circ -20{}^\circ =70{}^\circ \]](https://www.vedantu.com/seo/content-images/bc05539b-3566-4b65-82b4-202a5df72b7e_a..png)
![Complement of \[63{}^\circ =90{}^\circ -63{}^\circ =27{}^\circ \]](https://www.vedantu.com/seo/content-images/caa27bd5-5af0-4ceb-96d8-836f2a681a8f_b..png)
![Complement of \[57{}^\circ =90{}^\circ -57{}^\circ =33{}^\circ \]](https://www.vedantu.com/seo/content-images/69384ef8-b9d0-4a5f-a750-64752e6219ec_c..png)
![Supplement of \[105{}^\circ = 180{}^\circ -105{}^\circ =75{}^\circ \]](https://www.vedantu.com/seo/content-images/db6be495-b61c-4010-ba2d-0b8b182f820b_d..png)
![Supplement of \[87{}^\circ =180{}^\circ -87{}^\circ =93{}^\circ \]](https://www.vedantu.com/seo/content-images/9cc2cbc5-c305-4c3c-89dd-23aa276beba8_e..png)
![Supplement of \[154{}^\circ =180{}^\circ -154{}^\circ =26{}^\circ \]](https://www.vedantu.com/seo/content-images/3650506a-0a23-4a60-920f-d992f790a611_f..png)
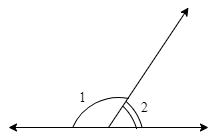
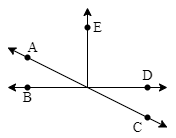
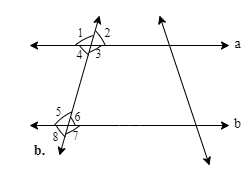
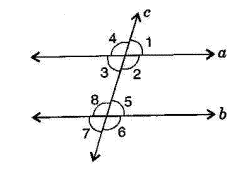
![\[(i)\] Given, \[l\parallel m\]and t is transversal line.](https://www.vedantu.com/seo/content-images/e7266142-03d5-4270-a31a-1792a17eab92_k..png)
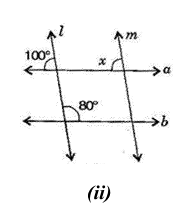
![\[(ii)\] Given, \[l\parallel m\]and t is transversal line.](https://www.vedantu.com/seo/content-images/dbd25bac-0ddc-4f77-80f2-4902f501793f_l..png)
![\[(i)\] \[126{}^\circ +44{}^\circ =170{}^\circ \]](https://www.vedantu.com/seo/content-images/b94d9094-65ba-44f8-b5ed-23f17114abd7_n..png)
![\[(ii)\] \[75{}^\circ +75{}^\circ =150{}^\circ \]](https://www.vedantu.com/seo/content-images/b085cdc1-d512-48d5-8773-f6c8fab0cbe8_o..png)
![\[(ii)\] \[75{}^\circ +75{}^\circ =150{}^\circ \]](https://www.vedantu.com/seo/content-images/4d425726-1c3b-47b7-ba03-c598217b37dc_p..png)
![\[(iii)\]\[57{}^\circ +123{}^\circ =180{}^\circ \]](https://www.vedantu.com/seo/content-images/20b75529-9aac-45ac-a474-c34e2592a602_q%2C.png)
![\[(iv)\]\[98{}^\circ +72{}^\circ =170{}^\circ \]](https://www.vedantu.com/seo/content-images/74604ab7-848d-40ba-a067-47f8f6aa7351_s..png)
