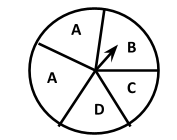
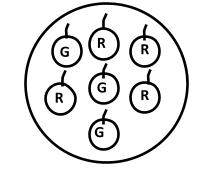
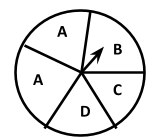
Class 8 Maths NCERT Solutions Chapter 4 Data Handling Exercise 4.2 - FREE PDF Download
NCERT Solutions for Class 8 Maths Chapter 4, Data Handling, continues to build on the basics with Ex 4.2 class 8. This exercise focuses on representing data using bar graphs and double bar graphs. These graphical tools are essential for comparing different sets of data visually, making it easier to identify trends and patterns.
In Ex 4.2 class 8, students should pay attention to accurately plotting data on bar graphs and double bar graphs. Understanding how to represent and interpret data visually is crucial, as it enhances analytical skills and prepares students for more advanced data handling tasks. By mastering these concepts, students can boost their confidence and perform better in exams, as highlighted by Vedantu.
Glance on NCERT Solutions Class 8 Maths Chapter 4 Exercise 4.2 | Vedantu
In this exercise we observe the outcome of a random event or experiment, such as flipping a coin or rolling a dice.
Chance refers to the possibility of a specific outcome occurring while probability quantifies that chance mathematically as a ratio between 0 and 1.
When each has the same probability of occurring, such as each side of a fair die then it is known as Equally Likely Outcomes.
Linking Chances to Probability connects the intuitive idea of chance with the formal calculation of probability, providing a numerical measure of likelihood.
In probability, an event is a set of possible outcomes, like drawing a red card from a deck.
This article contains exercise notes, important questions, exemplar solutions, exercises and video links for class 8 exercise 4.2 - Data Handling, which you can download as PDFs.
There are five questions in class 8 maths exercise 4.2 solutions pdf which are fully solved by experts at Vedantu.
Formulas Used in Class 8 Chapter 4 Exercise 4.2
Probability Formula: Probability = $Probability = \dfrac{Number of favorable outcomes}{Total number of outcomes}$
Sum of Probabilities of All Possible Outcomes: $P\left ( E \right )+P\left ( not E \right )= 1$
Refer to pages 8-10 for exercise 4.2 in the PDF
1. List the outcomes you can see in these experiments.
(a) Spinning a wheel (b) Tossing two coins together
(a) Ans: There are 4 possible outcomes. There are four letters A, B, C and D in a spinning wheel.
(b) Ans: When two coins are tossed together. There are four possible outcomes HH, HT, TH, TT, where H and T means head and tail.
2. When a die is thrown, list the outcomes of an event of getting:
(i) (a) a prime number (b) not a prime number
(ii) (a) a number greater than 5 (b) a number not greater than 5
Ans: When a dice is thrown, the possible outcomes are 1, 2, 3, 4, 5, and 6.
(i) (a) Ans: The outcomes of getting a prime number are 2, 3 and 5.
(b) Ans: 1, 4 and 6 are not prime numbers. Outcomes of events of not getting a prime number are 1, 4 and 6.
(ii) (a) Ans: Outcomes of the event of getting a number greater than 5 is only 6.
(b) Ans: Outcomes of events of not getting a number greater than 5 are 1, 2, 3, 4 and 5.
3. Find the:
(a) Probability of the pointer stopping on D in (Question 1 (a)).
(b) Probability of getting an ace from a well shuffled deck of 52 playing cards.
(c) Probability of getting a red apple. (See figure alongside)
(a) Ans: In a spinning wheel, there are five pointers A, A, B, C, D. So there are five outcomes. Pointer stops at D which is one outcome.
So the probability of the pointer stopping on D= \[\dfrac{1}{5}\]
(b) Ans: There are 4 aces in a deck of 52 playing cards. So, there are four events of getting an ace.
So, probability of getting an ace =\[\dfrac{4}{{52}} = \dfrac{1}{{13}}\]
(c) Ans: Total number of apples is 7 and in that there are 4 that are red.
Probability of getting red apple =\[\dfrac{4}{7}\]
4. Numbers 1 to 10 are written on ten separate slips (one number on one slip), kept in a box and
mixed well. One slip is chosen from the box without looking into it. What is the probability of:
(i) Getting a number 6.
(ii) Getting a number less than 6.
(iii) Getting a number greater than 6.
(iv) Getting a 1-digit number.
(i) Ans: There are 10 slips in the box and 6is written on only one slip.
Therefore, probability of getting a number 6 =\[\dfrac{1}{{10}}\]
(ii) Ans: Numbers less than 6 are 1, 2, 3, 4 and 5. So there are 5 outcomes.
Then the probability of getting a number less than 6 =\[\dfrac{5}{{10}} = \dfrac{1}{2}\]
(iii) Ans: Number greater than 6 out of ten that are 7, 8, 9, 10. So there are 4 possible outcomes.
Therefore, probability of getting a number greater than 6 =\[\dfrac{4}{{10}} = \dfrac{2}{5}\]
(iv) Ans: One digit numbers are 1, 2, 3, 4, 5, 6, 7, 8, 9 out of ten outcomes.
Therefore, probability of getting a 1-digit number =\[\dfrac{9}{{10}}\]
5. If you have a spinning wheel with 3 green sectors, 1 blue sector and 1 red sector, what is the probability of getting a green sector? What is the probability of getting a none-blue sector?
Ans: There are five sectors. Three sectors are green out of five sectors.
Therefore, probability of getting a green sector =\[\dfrac{3}{5}\]
There is one blue sector out of five sectors.
Non-blue sectors $ = 5 - 1 = 4$ sectors
Therefore, probability of getting a non-blue sector =\[\dfrac{4}{5}\]
6. Find the probability of the events given in Question 2.
Ans: When a die is thrown, there are a total six outcomes, i.e., 1, 2, 3, 4, 5 and 6.
(i) (a) Ans: The outcomes of the event of getting a prime number are 2, 3 and 5. So there are 3 outcomes out of 6.
Therefore, probability of getting a prime number =\[\dfrac{3}{6} = \dfrac{1}{2}\]
(b) Ans: 1, 4, 6 are not the prime numbers. So there are 3 outcomes out of 6.
Therefore, probability of getting a prime number =\[\dfrac{3}{6} = \dfrac{1}{2}\]
(ii) (a) Ans: Only 6 is greater than 5 in the possible outcomes. So there is one outcome out of 6.
Therefore, probability of getting a number greater than 5 =\[\dfrac{1}{6}\]
(b) Ans: Out of the 6 possible outcomes the number not greater than 5 are 5.
Therefore, probability of not getting a number greater than 5 = \[\dfrac{5}{6}\]
Conclusion
In Class 8 Maths Exercise 4.2 of Chapter 4 Data Handling, students dive into the practical application of probability concepts. Class 8 maths ex 4.2 emphasizes understanding the probability formula and applying it to various scenarios. Practicing these problems will enhance students' ability to solve probability-related questions efficiently, boosting their confidence in exams. Mastering these concepts is essential for tackling real-life problems involving chance and probability. By practicing these solutions, students can improve their problem-solving skills and achieve better results, as highlighted by Byju's and Vedantu.
Class 8 Maths Chapter 4: Exercises Breakdown
CBSE Class 8 Maths Chapter 4 Other Study Materials
Chapter-Specific NCERT Solutions for Class 8 Maths
Given below are the chapter-wise NCERT Solutions for Class 8 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
Important Related Links for CBSE Class 8 Maths
FAQs on NCERT Solutions for Class 8 Maths Chapter 4 Data Handling Ex 4.2
1. What do you mean by Probability in Class 8 Maths Chapter 5 Data Handling?
The possibility that something will happen or not can be defined as a probability. Therefore, the probability is the likelihood that an event that is fairly likely will really occur. The likelihood of rolling a six in the aforementioned case was one in six. That was the likelihood. Since probability is the same as a possibility, you could say that it is the likelihood that a specific event will occur.
2. Why is Class 8 Chapter 5 Data Handling Important?
Data handling is an important chapter because it paves the way for extracting information from unstructured data. In order to lay the groundwork for more complex ideas that a student might encounter while pursuing higher education, it is included in the class 8 math curriculum. In almost every field of study, it has wide-ranging implications for research and data analysis. Data handling involves a number of processes, all of which have their advantages. For example, these can be used to store and/or retrieve sensitive and high-end data.
3. Do I need to solve NCERT Solutions for Class 8 Maths Chapter 5 Data Handling (EX 5.3) Exercise 5.3?
In a subject like mathematics, simply studying the chapters is insufficient; you must first complete the corresponding exercises on your own. The more you practise, the more proficient you will become in this area. Only once you begin to solve problems will you be able to recognise your areas for improvement. You can refer to Vedantu for NCERT Solutions for Class 8 Maths Chapter 5 Data Handling (EX 5.3) Exercise 5.3 for a better understanding and learn various approaches to solve any problem.
4. Why should one opt for Vedantu for the NCERT Solutions for Class 8 Maths Chapter 5 Data Handling (EX 5.3) Exercise 5.3?
Teachers at Vedantu thrive on having as their main goal providing the students with the highest calibre of information. They have developed these NCERT Solutions for Class 8 Maths, Chapter 5, Exercise 5.3, to lessen the strain of test preparation as a result of their years of expertise. Students may easily comprehend the material provided in this chapter with the use of these solutions for NCERT Class 8 Maths Exercise 5.3 in Chapter 5. Our solutions include an interactive platform that helps students connect with tutors who are familiar with their issues. The Vedantu website makes its solutions freely accessible and absolutely free of charge in PDF format.
5. How many questions are there in NCERT Solutions for class 8 Maths Chapter 5 Data Handling (EX 5.3) Exercise 5.3?
Exercise 5.3 from Chapter 5 of Maths consists of a total of 6 problems. Data handling is one of the subjects covered by NCERT. Most of the questions are about probability. NCERT Solutions for Class 8 Maths Chapter 5 Data Handling (EX 5.3) Exercise 5.3 contains references to Vedantu. Here, subject-matter specialists provide precise answers that always result in good grades.
6. What is Exercise 4.2 in Class 8 Maths Chapter 4 about?
Exercise 4.2 focuses on the concept of probability. It teaches students how to calculate the likelihood of different outcomes. This exercise helps students understand and apply the probability formula. It is a foundational topic for further studies in mathematics and real-life applications.
7. What are equally likely outcomes in maths class 8 chapter 4 exercise 4.2?
In maths class 8 chapter 4 exercise 4.2 equally likely outcomes are those that have the same chance of occurring. For example, each side of a fair coin or die has an equal probability of landing face up. Understanding this concept is crucial for calculating accurate probabilities. It ensures that students can identify and differentiate between different types of outcomes.
8. How does practicing class 8 maths chapter 4 exercise 4.2 benefit students?
Practicing class 8 maths chapter 4 exercise 4.2 helps students develop a strong understanding of probability. It improves their problem-solving skills by applying theoretical knowledge to practical problems. Additionally, it prepares them for exam questions related to this topic. Mastery of these concepts boosts their confidence in handling probability-related questions.
9. What should students focus on while solving class 8 ex 4.2?
In class 8 maths ch 4 ex 4.2 students should focus on identifying the total number of outcomes for each scenario. They need to determine the number of favorable outcomes accurately. Applying the probability formula correctly is essential for solving problems. Understanding and practicing these steps will ensure accurate calculations and a solid grasp of probability concepts.
