NCERT Solutions for Class 9 Maths Chapter 1 - Number Systems Exercise 1.2 - FREE PDF Download
NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2
FAQs on NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2
1. Why should I opt for Vedantu’s NCERT solutions for class 9 maths Chapter 1 Exercise 1.2?
NCERT solutions for class 9 maths chapter 1 – Number Systems Exercise 1.2 is the second exercise of Chapter 1 of class 9 Maths. This exercise deals with Irrational numbers especially. Below are the advantages of opting for Vedantu’s NCERT Solutions.
These NCERT Solutions help you solve and revise all the questions from exercise 1.2.in a very less time.
After going through the stepwise solutions given by our subject expert teachers, you will be able to get more marks.
First and foremost, these solutions will help the students score the highest possible marks.
These are designed as per the NCERT guidelines which help in preparing the Class 9 students accordingly.
The solutions consist of answers to all the important questions from the final examination point of view.
2. What is Class 9 maths Chapter 1 Exercise 1.2 all about?
NCERT Solutions Class 9 Maths Chapter 1 Number Systems Exercise 1.2 offered by Vedantu are prepared by our subject matter experts which makes it easy for the CBSE board students to learn efficiently. The students refer to these while solving the exercise problems. Exercise 1.2 or the second exercise in Number Systems deals with the irrational numbers. These provide an in-depth and stepwise explanation of each of the questions given in the exercises in the NCERT textbook for class 9 chapter 2. The solutions are prepared as per the latest NCERT curriculum and guidelines so that it should cover the whole syllabus accordingly. These are very helpful in scoring the best possible marks in the examinations.
3. How many questions are there in Class 9 maths Chapter 1 Exercise 1.2?
Class 9 Maths Chapter 1 Exercise 1.2 of the NCERT textbook consists of four questions in total. Among which, three are long type questions and one short question. Our Vedantu solutions consist of answers to all these questions crafted by our excellent experts.
4. Can I download NCERT solutions Class 9 maths Chapter 1 Exercise 1.2 for free?
You can download the NCERT solutions Class 9 maths Chapter 1 Exercise 1.2 from Vedantu website and mobile application. These solutions which are provided in PDF format are prepared by our subject matter experts. The students can always use these for reference while solving the exercise problems.
5. What are the topics covered in Exercise 1.2 of Chapter 1 of Class 9 Maths?
There are seven topics covered in Chapter 1 Number System of Class 9 Maths. But the topic on which Exercise 1.2 is based is:
Exercise 1.1- Introduction to Number System
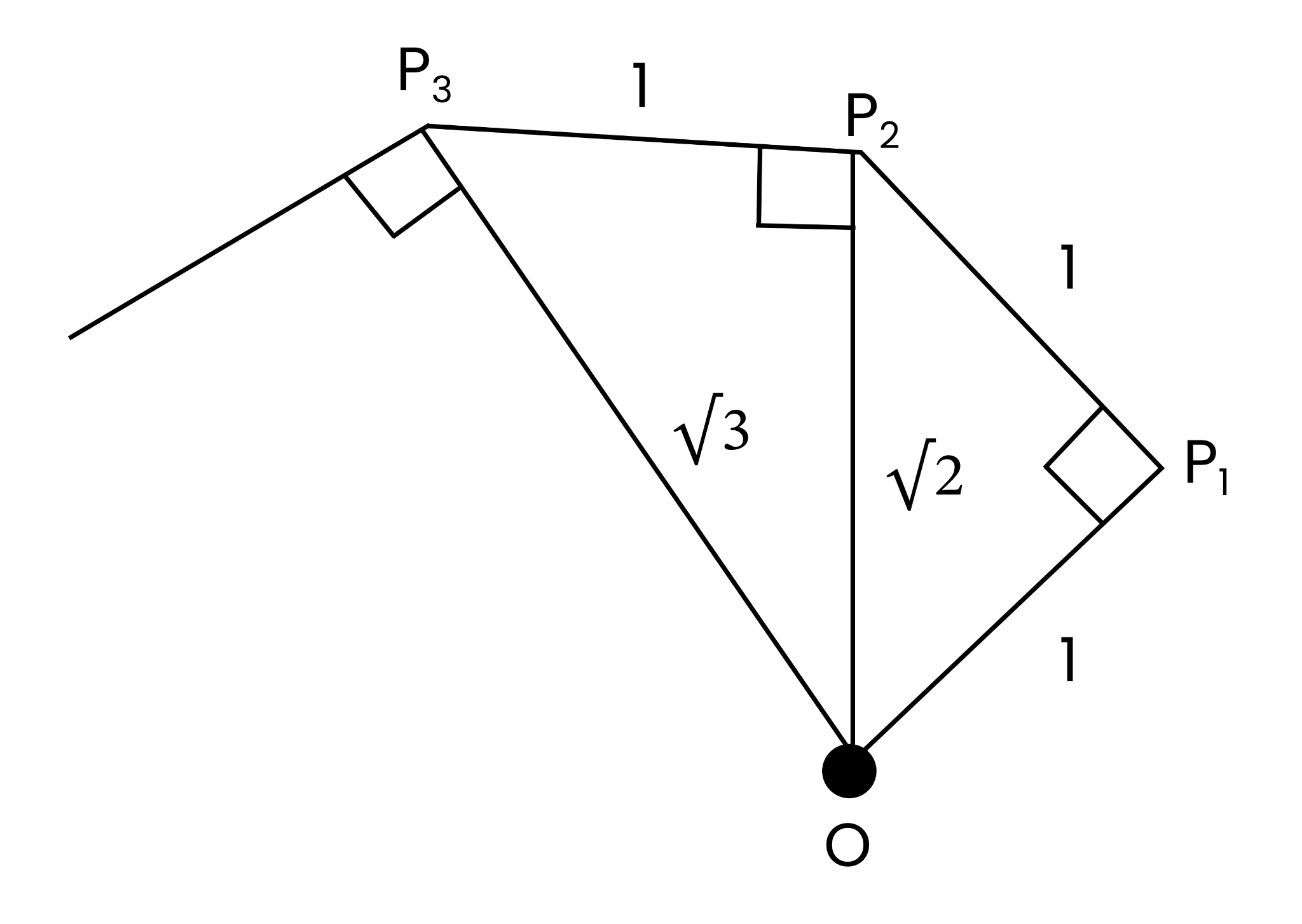
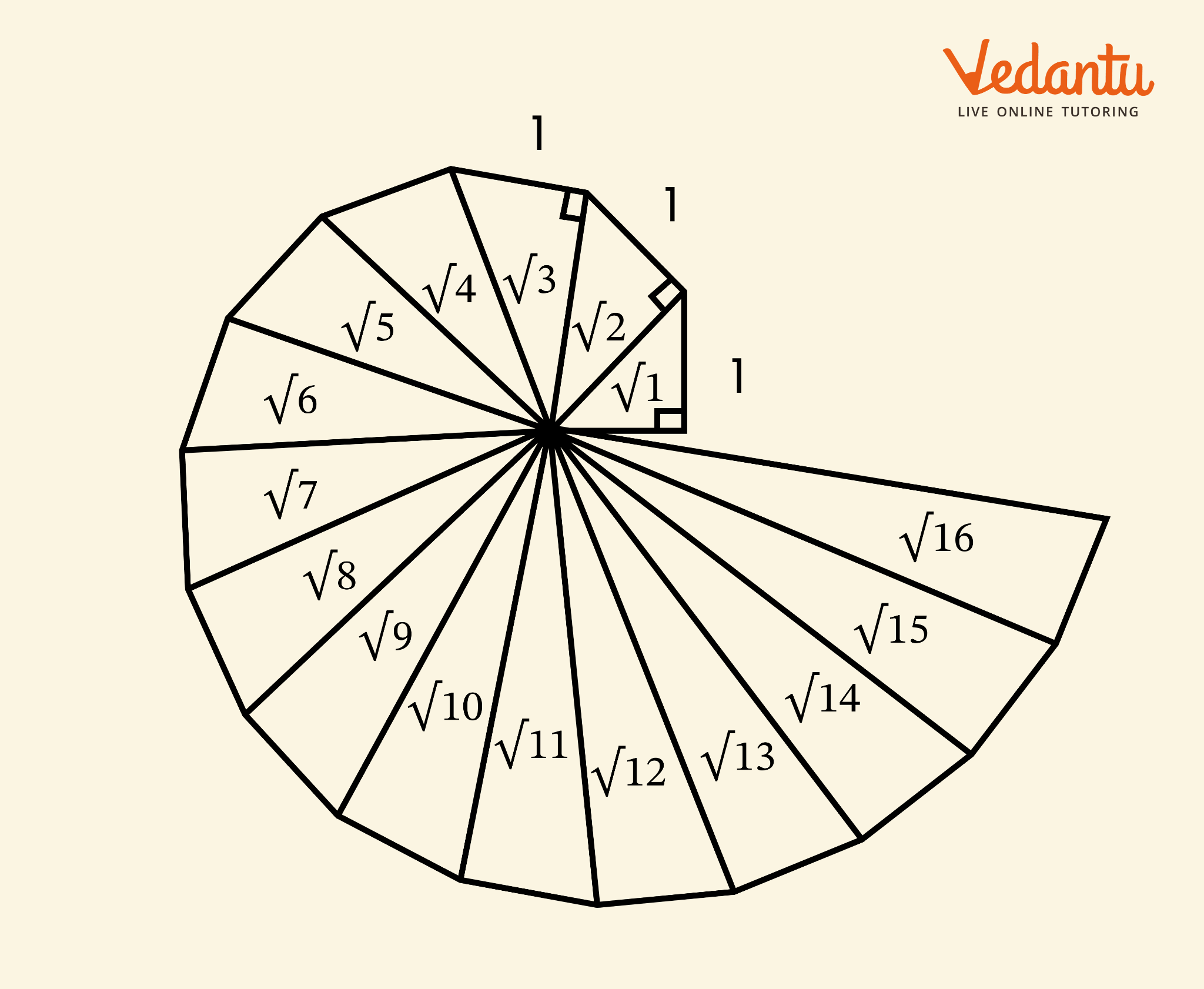
Exercise 1.2 deals with the Introduction to irrational numbers
Exercise 1.3-Real numbers and their decimal expansion
Exercise 1.4- Representing real numbers on the number line
Exercise 1.5- Operations on the real number
Exercise 1.6- Laws of exponents of real numbers
Summary of the chapter
Students can refer to the NCERT book of Class 9 Maths to understand these topics thoroughly.
6. How can I understand the Class 9 Maths Chapter 1 Number System Exercise 1.2?
If you want to understand Class 9 Maths Chapter 1 Number System easily, then check out Vedantu. Here, you will find a detailed explanation of the chapter in an understandable format. Moreover, you'll also get answers to the NCERT Maths questions for Class 9 Maths Chapter 1 Exercise 1.2 and numerous mock test papers to help you to understand the important concepts. These are available for free both on Vedantu’s website and on Vedantu’s mobile app.
7. How can I make a study plan for preparing Chapter 1 of Class 9 Maths?
To make a successful study plan for preparing the Chapter 1 Number System given in the Class 9 Maths book, you should follow the given steps:
Build a schedule balanced with all your daily activities.
Set a timetable and have a designated study area.
Limit your study time to two hours on this chapter to ensure you don’t lose your focus. You can increase or decrease the time limit according to your preference. During this time, read all the important concepts, solve questions, etc.
8. State whether the statement is true or false. Every point on the number line is in the form of √m where m is a natural number.
The given statement is false. It is wrong according to the norm ‘ a negative can’t be expressed as square roots.
For example- √16= 4 where 4 is a natural number. On the other hand, √3= 1.73 which is not a natural number. Similarly, negative numbers can also be represented on the number line but we know that a negative number changes into a complex number if we take the root of a negative number. For instance- √-8= 8i where i = √-1. Hence, the statement- every point on the number line is in the form of √m where m is a natural number is wrong.
9. How can I score full marks in Exercise 1.2 of Chapter 1 of Class 9 Maths?
Here are the useful tips to score good marks in Exercise 1.2 of Chapter 1 Number System in Class 9 Maths:
Keep a notebook for theories, formulas and methods to solve important questions for last moment revision.
Solve tough mathematical problems by yourself.
Understand Chapter 1 along with its weightage to filter important exercises.
Pick out the concepts of the chapter that require extra revision. You can do this by solving different question papers.
Keep your answer sheet clean during the exam.
10. What is the importance of Exercise 1.2 in Class 9 Maths?
Exercise 1.2 is essential for building a strong foundation in the Number System, which is fundamental for understanding more advanced mathematical concepts in higher classes.
11. How do you perform operations with different types of numbers in maths class 9 chapter 1 exercise 1.2?
Operations such as addition, subtraction, multiplication, and division are performed according to the properties of each type of number, such as closure, commutativity, associativity, and distributivity.
12. What are the properties of rational and irrational numbers discussed in class 9 maths chapter 1.2?
Properties such as closure under addition, subtraction, multiplication, and division for rational numbers, and non-repeatability and non-termination for irrational numbers are discussed.
13. How do you classify numbers in Class 9 Maths Exercise 1.2?
Numbers are classified into different categories such as natural numbers, whole numbers, integers, rational numbers, and irrational numbers based on their properties and characteristics.
14. What is the significance of understanding rational and irrational numbers in class 9th maths exercise 1.2?
Understanding rational and irrational numbers is crucial as they form the basis for solving various mathematical problems and are used in real-life applications such as measurements, calculations, and scientific analysis.
15. What are the properties of whole numbers discussed in Exercise 1.2?
Properties such as closure under addition and multiplication, commutativity, associativity, and distributivity are discussed for whole numbers in class 9 math ex 1.2.














 Watch Video
Watch Video