




What is Bohr magneton?: An Introduction
In 1913, values of angular momentum and magnetic moment were obtained by Bohr. In 1920, Wolfgang Pauli gave it the name Bohr Magneton. Bohr magneton is a physical constant value and it is the magnetic moment of an electron due to its angular momentum and also orbital momentum. Its value is 9.274 x 10-24 JT-1.
What is a Magnetic Moment?
Magnetic moment is also sometimes known as magnetic dipole moment. Magnetic moment is the measure of the tendency of an object to align itself with a magnetic field. It can also be stated as the magnetic strength and orientation of an object that produces a magnetic field. Magnetic moment is measured using a device called a Magnetometer. Magnetic moment is a vector quantity. S.I. unit is Am2. It can be given by formula \[\tau = m \times B\]. Here, \[\tau \] is Torque, m is magnetic moment and B is the external magnetic field.
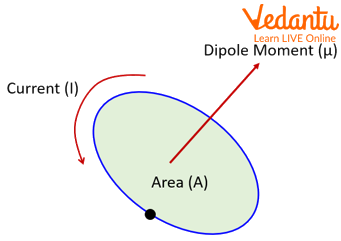
Dipole moment of the electron
What is Bohr Magneton?
Bohr magneton is a physical constant value. Bohr magneton is the magnetic moment of the electron due to its angular momentum and also its orbital momentum.
Bohr Magneton Formula
Electrons move in a circular path with a velocity v and path of radius r. The time is given by equation:
\[T = \dfrac{{2\pi r}}{\upsilon }\],
Because the electron is moving, there is some charge developed which we can give by equation:
\[I = \dfrac{{ - e\upsilon }}{{2\pi r}}\],
The magnetic moment can be given by equation, \[m = IA\],
Here A is the area of the circular path.
\[\mu = IA\]
\[\mu = \dfrac{{ - e\upsilon }}{{2\pi r}}\pi {r^2}\]
\[\mu = \dfrac{{ - evr}}{2}\]
Multiplying and dividing above equation by mass of electron
\[\mu = \dfrac{{ - evr}}{{2{m_e}}}{m_e}\]
\[{m_e}\upsilon r = L\], Which is angular momentum
\[\therefore \mu = \dfrac{{ - e}}{{2{m_e}}}\dfrac{{nh}}{{2\pi }}\] since, \[L = \dfrac{{nh}}{{2\pi }}\]
\[\mu = \dfrac{{neh}}{{4\pi {m_e}}}\]
Here Bohr Magneton is, \[{\mu _B} = \dfrac{{eh}}{{4\pi {m_e}}}\]
We can write \[\dfrac{h}{{2\pi }} = \hbar \]
\[\therefore \] Bohr magneton formula is \[{\mu _B} = \dfrac{{e\hbar }}{{2\pi }}\]
From this formula Bohr magneton value is 9.274 x 10-24 J/T
And SI unit of Bohr magneton is J/T (Joules per Tesla)
Interesting Facts
The strongest magnet in the universe is a star and it is called magnetar.
Our planet earth is like one big bar magnet
Magnets always have two poles
Solved Problems
1. What is the ratio of Bohr magneton to the nuclear magneton?Ans: Bohr magneton is a physical constant value and it is the magnetic moment of an electron due to its angular momentum and also orbital momentum. Nuclear magneton is the magnetic dipole moment of other heavier particles like protons, nuclei, etc. Bohr magneton is given by formula \[{\mu _B} = \dfrac{{e\hbar }}{{2{m_e}}}\]. Nuclear magneton is given by formula \[{\mu _N} = \dfrac{{e\hbar }}{{2{m_p}}}\]. Taking the ratio of these two we get, \[\dfrac{{{\mu _B}}}{{{\mu _N}}} = \dfrac{{{m_p}}}{{{m_e}}}\].
Ans: Electron moves in a circular path with a velocity v and path of radius r. The time is given by equation,
\[T = \dfrac{{2\pi r}}{\upsilon }\]
Because the electron is moving, there is some charge developed which we can give by equation,
\[I = \dfrac{{ - e\upsilon }}{{2\pi r}}\]
The magnetic moment can be given by equation, \[{M_0} = IA\],
Here A is the area of the circular path.
\[{M_0} = IA\]
\[{M_0} = \dfrac{{ - e\upsilon }}{{2\pi r}}\pi {r^2}\]
\[{M_0} = \dfrac{{ - evr}}{2}\]
Multiplying and dividing above equation by mass of electron
\[{M_0} = \dfrac{{ - evr}}{{2{m_e}}}{m_e}\]
\[{M_0} = \dfrac{{ - e}}{{2{m_e}}}{m_e}\upsilon r\]
We know that, angular momentum, \[{m_e}\upsilon r = {L_0}\]
Gyromagnetic ratio=magnetic moment/angular momentum
Gyromagnetic ratio=\[\dfrac{{{M_0}}}{{{L_0}}} = \dfrac{e}{{2{m_e}}}\]
Summary
Bohr magneton is a physical constant value. Bohr magneton is the magnetic moment of the electron due to its angular momentum and also its orbital momentum. The Bohr magneton formula is \[{\mu _B} = \dfrac{{e\hbar }}{{2\pi }}\]. Bohr magneton value is 9.274 x 10-24 J/T. SI unit of Bohr magneton is J/T (Joules per Tesla).
FAQs on Bohr Magneton: A Detailed Summary
1. What is the basic source of magnetism?
Each and every substance is made of tiny particles, which are called electrons. These particles carry electric charges. These electrons revolve around the nucleus which is the core of an atom. The movement of these generates an electric current and this causes each atom to act as a microscopic magnet. Magnetism is a kind of force which is exerted by magnets when they are attracting or repelling each other. This magnetism is the motion of the electric charges in atoms. In metals like Iron, Nickel all the electrons spin in the same direction. This makes these substances highly magnetic.
2. What is nuclear magneton?
Nuclear magneton is a unit of expressing magnetic moment of particles like protons, nucleons which are heavy particles. Bohr magneton has a larger value than the nuclear magneton because it has a larger charge to mass ratio. Nuclear magneton is given by formula \[{\mu _N} = \dfrac{{e\hbar }}{{2{m_p}}}\]. Where, \[{\mu _N}\] is nuclear magneton, e is elementary charge, \[\hbar \]is reduced Planck constant, \[{m_p}\]is proton rest mass. The ratio of Bohr magneton to nuclear magneton is \[\dfrac{{{\mu _B}}}{{{\mu _N}}} = \dfrac{{{m_p}}}{{{m_e}}}\].
3. Bohr magneton is almost 2000 times larger than nuclear magneton. Why?
Bohr magneton is for electrons whereas nuclear magneton is for protons. A proton is 2000 times heavier than the electron. So, the nuclear magnetic moment is 2000 times smaller than the Bohr magneton. The relation between Bohr magneton and nuclear magneton is given by ratio \[\dfrac{{{\mu _B}}}{{{\mu _N}}} = \dfrac{{{m_p}}}{{{m_e}}}\]. From this we get to know that the Bohr magneton and nuclear magneton both the quantities are inversely proportional to the mass of their respective particle. From this, we can say that Bohr magneton is almost 2000 times larger than the nuclear magneton.
























