




Graphical Method To Represent The Motion Of An Object
There are three equations of motion that we can use to derive a few physical quantities like initial velocity, final velocity, acceleration, displacement etc. Equations of motion by graphical methods play an important role when we want to derive such physical quantities. The motion of an object can be explained by a graphical method.
Graphical Method To Represent The Motion Of An Object
In the graphical method, we can study the motion of an object with the help of graphical representation of the curve of motion. Three equations of motion can be derived with the help of graphical representation. The first equation of motion is called velocity-time relation, the second equation of motion is called position-time relation and the third equation of motion is known as position-velocity relation.
Equation Of Motion For Velocity-Time Relation
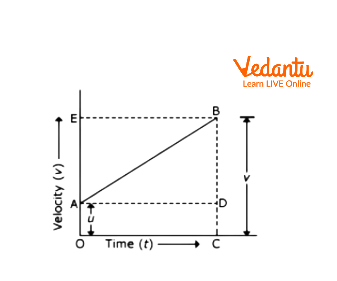
Velocity-Time Graph To Derive First Equation Of Motion
This graph shows that the object is moving with uniform acceleration. Here, we can see that this graph has not started from the origin. This shows that the object has not started from rest. That means, it has some initial velocity. gives us the initial velocity of an object.
Here, = initial velocity = =
= = final velocity =
= time taken by an object to reach at point B =
From the graph,
we can say that,
Therefore,
Acceleration can be calculated by the slope of this graph. So,
Acceleration,
So, in this way, with the help of a graphical method, we can derive the first equation of motion which is also known as velocity-time relation.
Equation Of Motion For Position-Time Relation
From the above figure,
We can calculate the distance travelled by an object.
= Area of
Therefore, = Area of + Area of
=
By substituting,
We will get,
This is the second equation of motion, which is known as position-time relation.
Equation Of Motion For Position-Velocity Relation
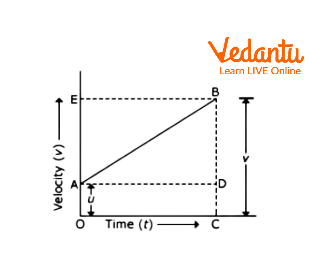
Velocity-Time Graph To Derive Third Equation Of Motion
In order to derive the third equation of motion, we will calculate the displacement of the object from point A to point B.
Here, = initial velocity = =
= = final velocity =
= time taken by an object to at reach point B =
From the graph, we can say that,
displacement = Area under the trapezium
This area will give us the displacement of the object from point A to point B.
Therefore,
Substituting the values, we have,
From the first equation of motion, put the value of in above equation,
We will get,
So, we will get, which is the third equation of motion. In this way, we can derive the third equation of motion by graphical method.
Solved Examples
1. A train starts from rest and accelerate uniformly at the rate of for Calculate the velocity of train in
Ans: Given: Here, a bus is starting from its rest position. So, we can take its initial velocity as
acceleration
time
Here, we will use the relation to find out its velocity,
So,
Hence, the velocity of train would be
2. A ball is gently dropped from a height of If its velocity increases uniformly at the rate of , with what velocity will it strike the ground? After what time will it strike the ground?
Ans: Given: Here, a ball is dropped from a height of
So, its initial velocity can be taken as
height
acceleration
Here, we will use the relation
So,
Therefore,
So, it will strike the ground with the velocity of
Now, we need to find out the value of time
So, we will use the relation
That means, if the ball is released from height, it will strike the ground after
Interesting Facts
Newton was the first scientist who developed laws of motion.
Gravitational forces don’t need to have contact with the objects.
Newton’s second law explains to us that unbalanced force causes acceleration.
Conclusion
The area under velocity versus time graph gives the displacement of the object. The slope of the velocity versus time graph gives us information about acceleration of an object. The physical quantities like distance, velocity, acceleration are related to each other in a certain way. These kinds of relations between these physical quantities are necessary to understand the behaviour of an object. So, graphical representation of motion plays an important role in Physics.
FAQs on Equations Of Motion By Graphical Method
1. Explain uniform motion and non-uniform motion.
If an object moves equal distances in equal intervals of time, then such a motion is called uniform motion. When an object travels unequal distances in equal intervals of time, then the object is said to have a non-uniform motion. This is a very common type of motion as compared to uniform motion. The blades of a fan which covers equal distances in equal intervals of time and the motion of the earth around the sun are some examples of uniform motion. A bouncing ball, motion of a bus on the road are some examples of non-uniform motion.
2. Explain various types of motion.
When an object changes its position with respect to some reference point is called motion.
Circular Motion: It is defined as motion of an object along the circumference of a circle. If an object is moving along the circumference of the circle, we can say that it is performing a circular motion. The satellite orbiting at a certain height is an example of circular motion.
Rotatory Motion: The spinning motion of an object about its axis is called rotatory motion. Rotating wheel and the spinning top are examples of rotational motion.
Oscillatory Motion: The motion of an object about its mean position is called oscillatory motion.
3. Define rest and motion.
Rest and motion are the two states in which an object could be found. The objects which can not move from one place to another and are fixed to their own place are said to be at rest. In other words, an object is said to be at rest if its position does not change with time. The trees and houses on the street are said to be at rest. The objects which seem to move from one place to another are said to be in motion. A moving car on the road and the flying birds are said to be in a state of motion.










