Question

Why is \[{{120}^{\circ }}\] the highest exterior angle for a regular polygon?

Answer 1

Hint: In this problem, we can see about the exterior angle of a regular polygon. We should know that the exterior angle is an angle which is formed by one of the sides of any closed shape structure such as a polygon and the extension of its adjacent side. Exterior angles of a polygon are formed by one of its sides and the extending the other side. We should also know that the sum of all the exterior angles in a polygon is equal to 360 degrees. We can now see about the highest exterior angle of a regular polygon.

Complete step-by-step answer:
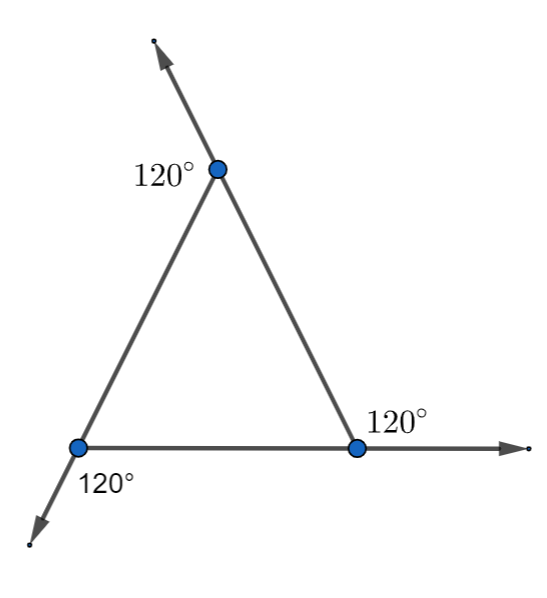
Here we can see about the highest exterior angle of a regular polygon.
We know that the exterior angle is an angle which is formed by one of the sides of any closed shape structure such as a polygon and the extension of its adjacent side.
We should also know that the sum of all the exterior angles in a polygon is equal to 360 degrees.
We know that an equilateral triangle is a polygon with 3 sides which has a minimum number of sides in polygons that will have the largest exterior angle.
So, if we divide the sum of all the exterior angles in a polygon is equal to 360 degrees with the number of sides, we will get,
\[\Rightarrow \dfrac{{{360}^{\circ }}}{3}={{120}^{\circ }}\]
Here we can see that, as the number of sides of a polygon decreases then the exterior angle increases.
So, we can come to a conclusion that, for the polygon with the minimum number of sides, it has the largest exterior angle (120 degrees).

Therefore, \[{{120}^{\circ }}\] is the highest exterior angle for a regular polygon.

Note: We should remember that as the number of sides of a polygon decreases then the exterior angle increases. An equilateral triangle is a polygon with 3 sides which has a minimum number of sides in polygons that will have the largest exterior angle. We should also know that the sum of all the exterior angles in a polygon is equal to 360 degrees.