
A
Answer
490.5k+ views
Hint: In a closed system total momentum before an event is same as that of total momentum after the event. Conservation of momentum is applied only to an isolated system of particles. Explosions and collisions can be used as an example for conservation of momentum.
Here a stationary bomb is exploded; hence conservation of momentum can be applied. Mass of particles and velocity are given in the question. We need to find the velocity of bigger parts. We have the equation which relates velocity and mass i.e., momentum
Formula used:
Complete answer:
Complete step by step solution:
Given that masses of three parts are in the ratio
i.e.
Given,
Here parts with the same mass travel perpendicular to the velocity.
Let’s draw the vector diagram of the particles with the same mass.
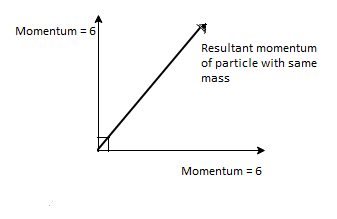
The momentum of particles with same mass,
Angle between the two momentum vectors is 90
Here we can apply the law of conservation of linear momentum.
Here the bomb is stationary. Hence,
Since the total momentum is conserved here.
Here the momentum is zero. Hence, momentum of particles with bigger mass should be opposite to the above resultant momentum of other two particles.

Momentum of particle with bigger mass,
Let’s find the magnitude of the above resultant momentum.
We have,
Applying the above formula to find the magnitude of momentum vector of particle with same mass,
Where,
This should be equal to momentum of particle with bigger mass,
Where,
So, the correct answer is “Option A”.
Note:
Alternate method to solve the question
Given that masses of three parts are in the ratio
Here the bomb was stationary. Hence,
By applying law of conservation of momentum,
Then,
Given,
Then,
We have,
Then,
Here a stationary bomb is exploded; hence conservation of momentum can be applied. Mass of particles and velocity are given in the question. We need to find the velocity of bigger parts. We have the equation which relates velocity and mass i.e., momentum
Formula used:
Complete answer:
Complete step by step solution:
Given that masses of three parts are in the ratio
i.e.
Given,
Here parts with the same mass travel perpendicular to the velocity.
Let’s draw the vector diagram of the particles with the same mass.

The momentum of particles with same mass,
Angle between the two momentum vectors is 90
Here we can apply the law of conservation of linear momentum.
Here the bomb is stationary. Hence,
Since the total momentum is conserved here.
Here the momentum is zero. Hence, momentum of particles with bigger mass should be opposite to the above resultant momentum of other two particles.

Momentum of particle with bigger mass,
Let’s find the magnitude of the above resultant momentum.
We have,
Applying the above formula to find the magnitude of momentum vector of particle with same mass,
Where,
This should be equal to momentum of particle with bigger mass,
Where,
So, the correct answer is “Option A”.
Note:
Alternate method to solve the question
Given that masses of three parts are in the ratio
Here the bomb was stationary. Hence,
By applying law of conservation of momentum,
Then,
Given,
Then,
We have,
Then,
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




