
A 2

Answer
497.7k+ views
1 likes
Hint: First, we need to find out the initial energy stored in the
Formula used:
The energy stored in a capacitor having capacitance C and voltage V is given as
Complete answer:
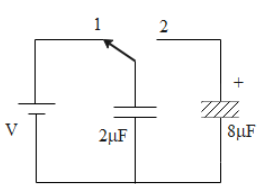
In the given diagram when the switch S is connected to position 1 then the charging of the
Now when the switch S is connected to position two then the total voltage V will be shared between the two capacitors as the
Now the total energy stored in the system of two capacitors will be
Now we can calculate the energy dissipated by dividing the change in energy with the initial energy and multiplying with 100 to get value in percentage.
Therefore, the percentage energy dissipated
This is the required value.
Hence, the correct answer is option D.
Note:
1. It should be noted that 80
2. The energy is stored in a capacitor in the form of charge. The expression of energy stored can be given in the form of charge by using the relation
Formula used:
The energy stored in a capacitor having capacitance C and voltage V is given as
Complete answer:
In the given diagram when the switch S is connected to position 1 then the charging of the
Now when the switch S is connected to position two then the total voltage V will be shared between the two capacitors as the
Now the total energy stored in the system of two capacitors will be
Now we can calculate the energy dissipated by dividing the change in energy with the initial energy and multiplying with 100 to get value in percentage.
Therefore, the percentage energy dissipated
This is the required value.
Hence, the correct answer is option D.
Note:
1. It should be noted that 80
2. The energy is stored in a capacitor in the form of charge. The expression of energy stored can be given in the form of charge by using the relation
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




