
A ball whose kinetic energy is E, is thrown at an angle of ${{45}^{0}}$ with the horizontal, its kinetic energy at the highest point of its flight will be?
$\begin{align}
& \text{A}\text{. }E \\
& \text{B}\text{. }\dfrac{E}{\sqrt{2}} \\
& \text{C}\text{. }\dfrac{E}{2} \\
& \text{D}\text{. zero} \\
\end{align}$
Answer
422.8k+ views
Hint: The ball is thrown at angle with the horizontal. So, the ball will follow a projectile motion. Find the velocity of an object in projectile motion at the highest point of flight. Find the kinetic energy and compare it with the initial kinetic energy to find the required answer.
Complete answer:
A ball is projected at an angle of ${{45}^{0}}$ with the horizontal. This is a projectile motion.
Let the initial velocity of the ball is u.
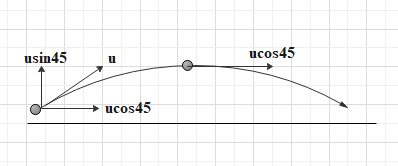
Now, the horizontal component of the velocity will be, $u\cos {{45}^{0}}=\dfrac{u}{\sqrt{2}}$ .
Again, the vertical component of the velocity will be, $u\sin {{45}^{0}}=\dfrac{u}{\sqrt{2}}$
In a projectile motion, at the highest point of its flight, the vertical component of the velocity becomes zero and the velocity of the object is given only by the horizontal component of the velocity.
So, at the height of point of flight of a projectile motion, the velocity of the object will be $\dfrac{u}{\sqrt{2}}$ .
Now, initially the kinetic energy of the ball is E when the object starts moving with velocity u.
So, we can write that,
$E=\dfrac{1}{2}m{{u}^{2}}$
Where, m is the mass of the ball.
Now, at the highest point of flight, the ball is moving with velocity $\dfrac{u}{\sqrt{2}}$ . so, its kinetic energy will be,
${{E}_{f}}=\dfrac{1}{2}m{{\left( \dfrac{u}{\sqrt{2}} \right)}^{2}}=\dfrac{1}{4}m{{u}^{2}}$
Now, dividing the second equation with the first equation we get that,
$\begin{align}
& \dfrac{{{E}_{f}}}{E}=\dfrac{\dfrac{1}{4}m{{u}^{2}}}{\dfrac{1}{2}m{{u}^{2}}} \\
& {{E}_{f}}=\dfrac{E}{2} \\
\end{align}$
So, the kinetic energy of the ball at its highest point of flight will be, $\dfrac{E}{2}$.
So, the correct answer is “Option C”.
Note:
For an object in projectile motion we can directly say that the velocity of the object at its highest point of flight is the horizontal component of the initial velocity. If u, the initial velocity of the object makes an angle of $\theta $ with the horizontal, the velocity of the object at its highest point of flight will be $u\cos \theta $.
Complete answer:
A ball is projected at an angle of ${{45}^{0}}$ with the horizontal. This is a projectile motion.
Let the initial velocity of the ball is u.

Now, the horizontal component of the velocity will be, $u\cos {{45}^{0}}=\dfrac{u}{\sqrt{2}}$ .
Again, the vertical component of the velocity will be, $u\sin {{45}^{0}}=\dfrac{u}{\sqrt{2}}$
In a projectile motion, at the highest point of its flight, the vertical component of the velocity becomes zero and the velocity of the object is given only by the horizontal component of the velocity.
So, at the height of point of flight of a projectile motion, the velocity of the object will be $\dfrac{u}{\sqrt{2}}$ .
Now, initially the kinetic energy of the ball is E when the object starts moving with velocity u.
So, we can write that,
$E=\dfrac{1}{2}m{{u}^{2}}$
Where, m is the mass of the ball.
Now, at the highest point of flight, the ball is moving with velocity $\dfrac{u}{\sqrt{2}}$ . so, its kinetic energy will be,
${{E}_{f}}=\dfrac{1}{2}m{{\left( \dfrac{u}{\sqrt{2}} \right)}^{2}}=\dfrac{1}{4}m{{u}^{2}}$
Now, dividing the second equation with the first equation we get that,
$\begin{align}
& \dfrac{{{E}_{f}}}{E}=\dfrac{\dfrac{1}{4}m{{u}^{2}}}{\dfrac{1}{2}m{{u}^{2}}} \\
& {{E}_{f}}=\dfrac{E}{2} \\
\end{align}$
So, the kinetic energy of the ball at its highest point of flight will be, $\dfrac{E}{2}$.
So, the correct answer is “Option C”.
Note:
For an object in projectile motion we can directly say that the velocity of the object at its highest point of flight is the horizontal component of the initial velocity. If u, the initial velocity of the object makes an angle of $\theta $ with the horizontal, the velocity of the object at its highest point of flight will be $u\cos \theta $.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

Trending doubts
10 examples of friction in our daily life

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

State the laws of reflection of light

Write down 5 differences between Ntype and Ptype s class 11 physics CBSE




