
A binary star system consists of two stars of masses
Answer
499.2k+ views
Hint: The point around which the stars in the binary star system revolve acts as the centre of mass of the system. By the property of the centre of mass, the product of mass and radius of one star in the binary star system is equal to the product of mass and radius of the other star. Also, gravitational force between the two stars in the binary star system is equal to the centripetal force acting on each star.
Formula used:
Complete step by step answer:
We are provided with a binary star system, consisting of two stars of masses
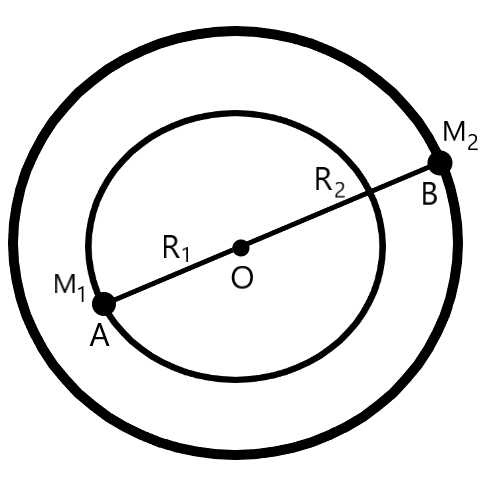
The point around which both the stars in the binary star system revolve acts as the centre of mass of the system. Clearly, in the given figure,
where
Let this be equation 1.
Now, force of gravitation between star
where
Let this be equation 2.
Another force which acts on each star is centripetal force, which keeps each star revolving around
where
Let this be equation 3.
Similarly, if
where
Let this be equation 4.
Now, for the binary system of stars to be stable, we know that all these forces acting on each star should be equal. Therefore, we can equate equation 2, equation 3 and equation 4, as follows:
Let this be equation 5.
Here, we know that
and
where
Let this set of equations be denoted by X.
Substituting the set of equations denoted by X in equation 5, we have
Let this be equation 6.
Using equation 1 in equation 6, we have
This result suggests that time periods of revolution of both the stars in the given binary system of stars are equal.
Therefore, the correct answer is option
Note:
Students need not get confused with the derivation given by equation 5. Equation 5 is nothing but a consequence of Kepler’s third law of planetary motion, which states that
where
This expression looks very similar to option
which contradicts the assumptions put forward by the question. Therefore, option
Formula used:
Complete step by step answer:
We are provided with a binary star system, consisting of two stars of masses

The point around which both the stars in the binary star system revolve acts as the centre of mass of the system. Clearly, in the given figure,
where
Let this be equation 1.
Now, force of gravitation between star
where
Let this be equation 2.
Another force which acts on each star is centripetal force, which keeps each star revolving around
where
Let this be equation 3.
Similarly, if
where
Let this be equation 4.
Now, for the binary system of stars to be stable, we know that all these forces acting on each star should be equal. Therefore, we can equate equation 2, equation 3 and equation 4, as follows:
Let this be equation 5.
Here, we know that
and
where
Let this set of equations be denoted by X.
Substituting the set of equations denoted by X in equation 5, we have
Let this be equation 6.
Using equation 1 in equation 6, we have
This result suggests that time periods of revolution of both the stars in the given binary system of stars are equal.
Therefore, the correct answer is option
Note:
Students need not get confused with the derivation given by equation 5. Equation 5 is nothing but a consequence of Kepler’s third law of planetary motion, which states that
where
This expression looks very similar to option
which contradicts the assumptions put forward by the question. Therefore, option
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




