
A block (B) is attached to two unstretched springs
(A)
(B)
(C)
(D)

Answer
402.9k+ views
1 likes
Hint: We are asked to find the ratio
Potential energy of a spring is given by the formula,
Where
Complete answer:
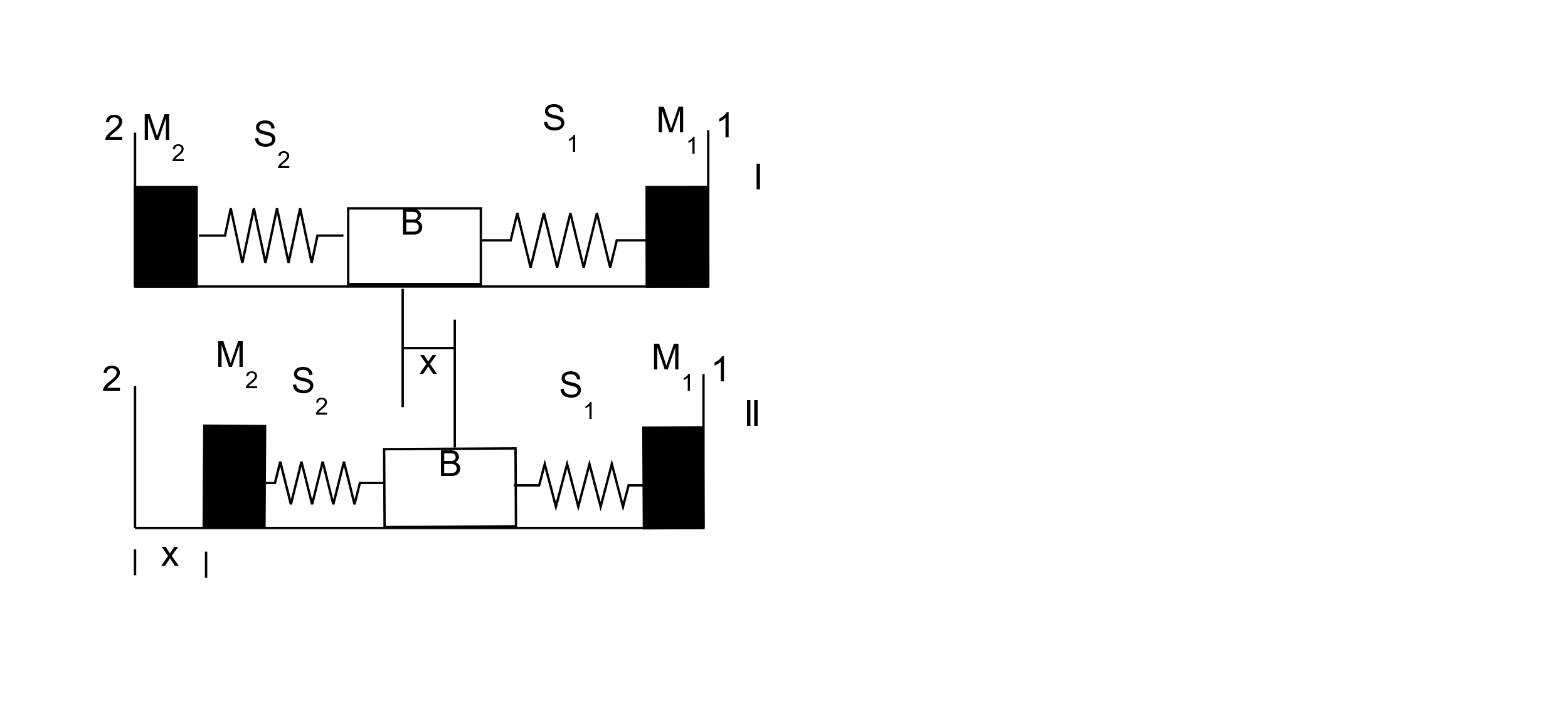
We can start by considering the two diagrams as said in the question. The two diagrams (displacement with respect to x and displacement with respect to y) will be as follows
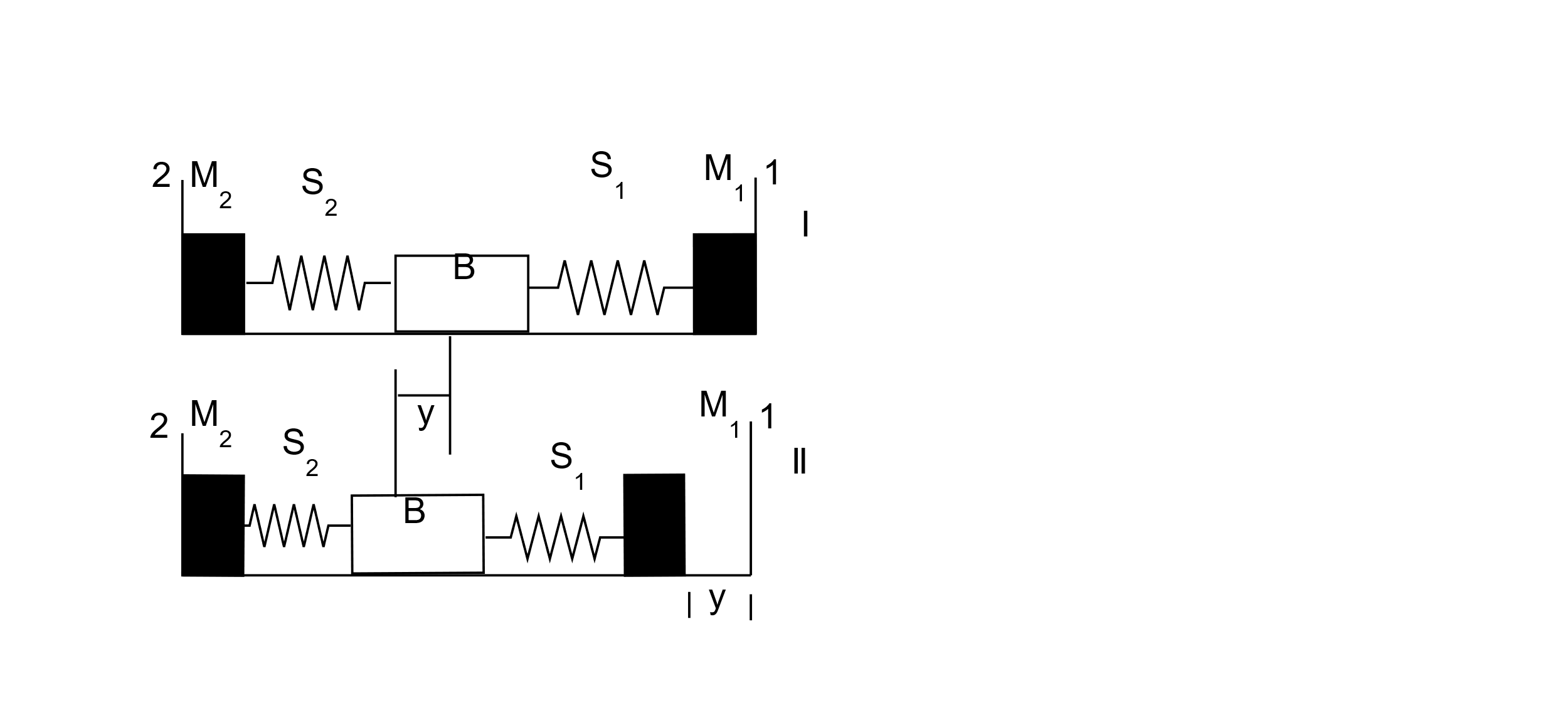
Now that we have the right diagrams with respect to the displacement x and y, we can move onto finding the ratio as asked. In order to do this, we need to establish a relationship so as to move forward. The velocity of the blocks is considered first, but the velocity of both cases is zero. Now we move onto potential energy of the spring
We can use the sum of kinetic energy and potential energy (total energy) here.
The kinetic energy of the springs are zero hence we arrive at,
But one of the spring constants (spring constant of the second displacement, y) is
Substituting that we get,
We bring the like terms to one side and get
We can now take the square root of this equation to get the final answer as,
In conclusion, the right answer is option (C)
Note:
Spring constant or the force constant is derived by Hooke's law. Hooke's law, law of elasticity discovered by the English scientist Robert Hooke in 1660, states that, for relatively small deformations of an object, the displacement or size of the deformation is directly proportional to the deforming force or load.
Potential energy of a spring is given by the formula,
Where
Complete answer:
We can start by considering the two diagrams as said in the question. The two diagrams (displacement with respect to x and displacement with respect to y) will be as follows


Now that we have the right diagrams with respect to the displacement x and y, we can move onto finding the ratio as asked. In order to do this, we need to establish a relationship so as to move forward. The velocity of the blocks is considered first, but the velocity of both cases is zero. Now we move onto potential energy of the spring
We can use the sum of kinetic energy and potential energy (total energy) here.
The kinetic energy of the springs are zero hence we arrive at,
But one of the spring constants (spring constant of the second displacement, y) is
Substituting that we get,
We bring the like terms to one side and get
We can now take the square root of this equation to get the final answer as,
In conclusion, the right answer is option (C)
Note:
Spring constant or the force constant is derived by Hooke's law. Hooke's law, law of elasticity discovered by the English scientist Robert Hooke in 1660, states that, for relatively small deformations of an object, the displacement or size of the deformation is directly proportional to the deforming force or load.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




