
A body that starts from rest accelerates uniformly along a straight line at the rate of

Answer
405.9k+ views
Hint: The distance travelled is shown by the area under the speed-time graph. For the motion of a particle, distance-time graphs and acceleration-time graphs can be produced, with time always displayed on the horizontal axis. A particle with a constant speed is represented by a straight line on a distance-time graph.
Complete step by step answer:
Let us first write the given values
Acceleration
Time
Therefore the velocity can be found by using equation of motion that is given by:
Now, it moves for
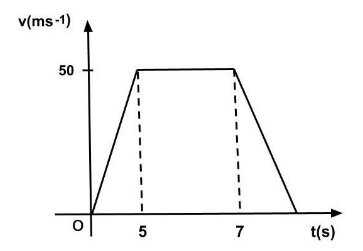
Now, according to the graph: the total distance travelled is
Hence, the total distance travelled by the body is
Note: It's important to remember that finding the area doesn't fully describe displacement because, being a vector quantity, displacement also requires a direction.Finding the location simply provides a number, not a direction. The magnitude of the displacement, which is equivalent to the distance travelled, is represented by the area under the curve (only for constant acceleration).
Complete step by step answer:
Let us first write the given values
Acceleration
Time
Therefore the velocity can be found by using equation of motion that is given by:
Now, it moves for

Now, according to the graph: the total distance travelled is
Hence, the total distance travelled by the body is
Note: It's important to remember that finding the area doesn't fully describe displacement because, being a vector quantity, displacement also requires a direction.Finding the location simply provides a number, not a direction. The magnitude of the displacement, which is equivalent to the distance travelled, is represented by the area under the curve (only for constant acceleration).
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




