
A bomb is dropped from an aeroplane when it is directly above a target at a height of 1000 m. The aeroplane is moving horizontally with a speed of 500km/h. By how much distance will the bomb miss the target?
Answer
501.6k+ views
Hint: A bomb is dropped from a moving aeroplane. We know that the bomb will have a velocity in the direction of motion of the aeroplane. And its velocity along the y direction will be zero. By using the equations of motion we can solve for time of flight. Thus we have time and speed is already given, using this we can solve for distance.
Formula used:
Third kinematic equation,
Complete answer:
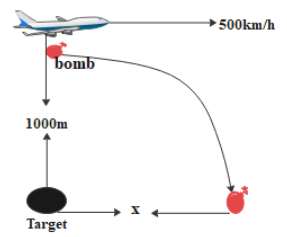
We have an aeroplane moving horizontally in the x direction with a velocity 500km/h.
A bomb is dropped from the plane when it is right above its target.
The height from the target to the plane is given as 1000m.
We know that when the bomb is dropped from a moving plane, the bomb will have a velocity in the horizontal direction. Hence the bomb will not hit the target; it will fall at a distance ‘x’ from the target.
We can say that the initial velocity of the bomb in the vertical direction will be zero.
By the third kinematic equation, we have
Here displacement, ‘s’ in y direction is 1000m, initial velocity is zero and acceleration ‘a’ is the acceleration due to gravity
Therefore,
Now we have time of flight
We have the horizontal velocity,
Therefore the distance from the target to the bomb ‘x’ will be
Hence the bomb missed the target by 1.96km.
Note:
Here the motion of the bomb is projectile motion and the bomb is a projectile.
A projectile motion is the motion of a body only under the influence of gravity. The body in projectile motion is a projectile.
Here the initial velocity of the bomb is zero because we are not giving any kind of external velocity to the bomb. The bomb is just dropped and it continues to fall only with the influence of gravity.
Formula used:
Third kinematic equation,
Complete answer:

We have an aeroplane moving horizontally in the x direction with a velocity 500km/h.
A bomb is dropped from the plane when it is right above its target.
The height from the target to the plane is given as 1000m.
We know that when the bomb is dropped from a moving plane, the bomb will have a velocity in the horizontal direction. Hence the bomb will not hit the target; it will fall at a distance ‘x’ from the target.
We can say that the initial velocity of the bomb in the vertical direction will be zero.
By the third kinematic equation, we have
Here displacement, ‘s’ in y direction is 1000m, initial velocity is zero and acceleration ‘a’ is the acceleration due to gravity
Therefore,
Now we have time of flight
We have the horizontal velocity,
Therefore the distance from the target to the bomb ‘x’ will be
Hence the bomb missed the target by 1.96km.
Note:
Here the motion of the bomb is projectile motion and the bomb is a projectile.
A projectile motion is the motion of a body only under the influence of gravity. The body in projectile motion is a projectile.
Here the initial velocity of the bomb is zero because we are not giving any kind of external velocity to the bomb. The bomb is just dropped and it continues to fall only with the influence of gravity.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




