
A bomb is dropped from an airplane flying horizontally with a velocity of
A.
B.
C.
D.
Answer
487.8k+ views
Hint: The bomb is moving with the velocity same as that of the plane when it is dropped. After it is dropped, gravitational force due to earth acts on it which accelerates it in a vertically downward direction. The bomb, when seen from the plane, hits directly below the plane.
Time taken can be calculated by using the equation of motion.
Formula used:
Complete step-by-step answer:
When the bomb is dropped its vertical velocity is zero as it moves with the velocity the same as that of the plane. The trajectory of bomb is shown in figure below.
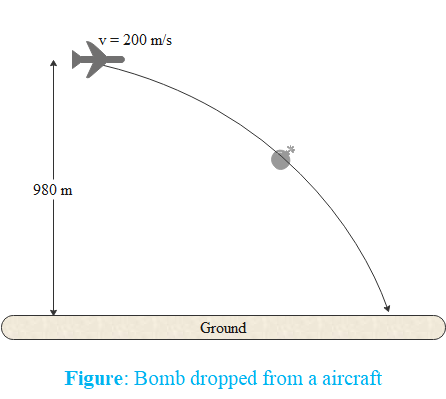
The height of the airplane from the ground is 980 m. So, the bomb will have to travel 980 m vertically downward to reach the ground.
During downward motion, the bomb can be considered as an object under free fall.
If u is the initial velocity of an object and a is its acceleration then, it will cover distance s in time, t. Mathematically,
Since, the vertical initial velocity of the bomb is zero,
Assuming time taken by bomb to hit the ground as T, we get
Substituting the values, we have
The bomb will take 14.14 seconds to hit the ground.
So, the correct answer is “Option C”.
Note: The expression for time taken during free fall should be learned as it helps us save a lot of time by directly getting the result.
Although the bomb travels in a horizontal direction, the vertical distance travelled depends only on the vertical component of velocity.
Time taken can be calculated by using the equation of motion.
Formula used:
Complete step-by-step answer:
When the bomb is dropped its vertical velocity is zero as it moves with the velocity the same as that of the plane. The trajectory of bomb is shown in figure below.

The height of the airplane from the ground is 980 m. So, the bomb will have to travel 980 m vertically downward to reach the ground.
During downward motion, the bomb can be considered as an object under free fall.
If u is the initial velocity of an object and a is its acceleration then, it will cover distance s in time, t. Mathematically,
Since, the vertical initial velocity of the bomb is zero,
Assuming time taken by bomb to hit the ground as T, we get
Substituting the values, we have
The bomb will take 14.14 seconds to hit the ground.
So, the correct answer is “Option C”.
Note: The expression for time taken during free fall should be learned as it helps us save a lot of time by directly getting the result.
Although the bomb travels in a horizontal direction, the vertical distance travelled depends only on the vertical component of velocity.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




