
A boy was asked to find the LCM of 3, 5, 12 and another number. But while calculating, he wrote 21 instead of 12 and yet came with the correct answer. What could be the fourth number?
Answer
480.6k+ views
Hint: LCM refers to the least common multiple among the given numbers. It is given that in both cases, the LCM calculated was the same. Then the fourth number will be the product of those numbers that aren’t common between the two cases because only then the LCM will be the same.
Complete step-by-step answer:
Let the fourth number be x.
Then the numbers in respective cases are:
Case 1: 3, 5, 12, x
In the next case he wrote 21 instead of 12, so
Case 2: 3, 5, 21, x
The number different in the cases are 12 and 21, their prime factorization is given as:
\[
\Rightarrow {12 = 2 \times 2 \times 3} \\
\Rightarrow {21 = 7 \times 3}
\]
For the LCM to be the same in both the cases, the fourth number should be the product of those numbers which are uncommon between the factors of 12 and 21, therefore:
\[
\Rightarrow x = 7 \times 2 \times 2 \\
\Rightarrow x = 7 \times 4 \\
\]
x = 28
Thus the fourth number is 28.
This can also be verified by equating the LCMs in both cases by substituting the value of x.
LCM (3, 5, 12, 28) = LCM (3, 5, 21, 28)
The prime factors for both LHS and RHS can be written as:
\[3 \times 5 \times 3 \times 2 \times 2 \times 7 \times 2 \times 2 = 3 \times 5 \times 7 \times 2 \times 2 \times 7 \times 2 \times 2\]
Taking common values out of these for LCM:
\[3 \times 2 \times 2 \times 7 = 3 \times 2 \times 2 \times 7\]
420 = 420
It is proved the LCM is the same for both the cases.
Therefore, the fourth number the boy took for calculating LCM is 4.
Note: To avoid confusion calculate LCM after writing prime factors like:
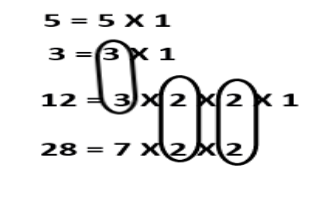
The LCM here will be \[3 \times 2 \times 2 \times 5 \times 7\]
Remember: the pair among the factors will be counted only once whereas the numbers occurring not in pairs will be written as it is.
LCM calculated cannot be less than either of the numbers.
Prime factors are those numbers whose factors are one and number itself.
Complete step-by-step answer:
Let the fourth number be x.
Then the numbers in respective cases are:
Case 1: 3, 5, 12, x
In the next case he wrote 21 instead of 12, so
Case 2: 3, 5, 21, x
The number different in the cases are 12 and 21, their prime factorization is given as:
\[
\Rightarrow {12 = 2 \times 2 \times 3} \\
\Rightarrow {21 = 7 \times 3}
\]
For the LCM to be the same in both the cases, the fourth number should be the product of those numbers which are uncommon between the factors of 12 and 21, therefore:
\[
\Rightarrow x = 7 \times 2 \times 2 \\
\Rightarrow x = 7 \times 4 \\
\]
x = 28
Thus the fourth number is 28.
This can also be verified by equating the LCMs in both cases by substituting the value of x.
LCM (3, 5, 12, 28) = LCM (3, 5, 21, 28)
The prime factors for both LHS and RHS can be written as:
\[3 \times 5 \times 3 \times 2 \times 2 \times 7 \times 2 \times 2 = 3 \times 5 \times 7 \times 2 \times 2 \times 7 \times 2 \times 2\]
Taking common values out of these for LCM:
\[3 \times 2 \times 2 \times 7 = 3 \times 2 \times 2 \times 7\]
420 = 420
It is proved the LCM is the same for both the cases.
Therefore, the fourth number the boy took for calculating LCM is 4.
Note: To avoid confusion calculate LCM after writing prime factors like:
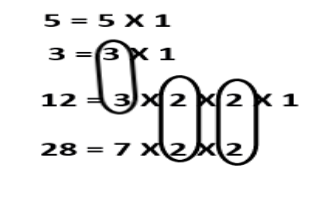
The LCM here will be \[3 \times 2 \times 2 \times 5 \times 7\]
Remember: the pair among the factors will be counted only once whereas the numbers occurring not in pairs will be written as it is.
LCM calculated cannot be less than either of the numbers.
Prime factors are those numbers whose factors are one and number itself.
Recently Updated Pages
The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

A certain household has consumed 250 units of energy class 11 physics CBSE

The lightest metal known is A beryllium B lithium C class 11 chemistry CBSE

What is the formula mass of the iodine molecule class 11 chemistry CBSE

Trending doubts
When Sambhaji Maharaj died a 11 February 1689 b 11 class 8 social science CBSE

Explain the system of Dual Government class 8 social science CBSE

What is Kayal in Geography class 8 social science CBSE

Who is the author of Kadambari AKalidas B Panini C class 8 social science CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science




