Question

A bullet is fired from a gun at the speed of $5000m/\sec $. At what height should the gun aim above the target if it has to strike the target at a distance of $500m$? Take $g = 10m/{s^2}$and negligible air resistance.
A. $0.05m = 5cm$
B. $0.03m = 3cm$
C. $0.09m = 9cm$
D. $0.08m = 8cm$

Answer 1

Hint: When a gun is aimed at a target generally it would be aimed horizontally. A bullet would come out of the barrel with some velocity horizontally. But due to gravity the bullet travels vertically too. So the bullet will have both horizontal and vertical motion. Time taken to hit the target will be found and from that we will find out distance bullet travelled vertically by using kinematic formula
Formula used:
$s = ut + \dfrac{1}{2}a{t^2}$

Complete step-by-step solution
let the time taken to hit the target be ‘t’ seconds.
Bullet will be having initial horizontal velocity only and it doesn’t have an initial vertical velocity.
Now from the formula
$s = ut + \dfrac{1}{2}a{t^2}$ … eq(1)
Where in this problem
S = vertical distance traveled by bullet
u = initial vertical velocity of the bullet
t = time taken for the bullet to hit the target
a = bullet acceleration in the vertical direction
The situation is represented in the diagram below

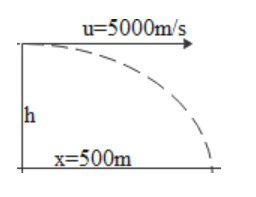
According to diagram above along y direction u = 0, a = acceleration due to gravity(g) = 10
Substituting the above values in equation 1 we get
$s = ut + \dfrac{1}{2}a{t^2}$
$\eqalign{
  & \Rightarrow {s_y} = 0 \times t + \dfrac{1}{2} \times 10 \times {[t]^2} \cr
  & \Rightarrow {s_y} = \dfrac{1}{2} \times 10 \times {[t]^2} = 5{t^2}m \cr} $
Now along the horizontal direction there is no acceleration and distance to be travelled is 500m and initial horizontal velocity is 5000m/sec
By applying $s = ut + \dfrac{1}{2}a{t^2}$ in horizontal direction we get time.
$s = ut + \dfrac{1}{2}a{t^2}$
$\eqalign{
  & \Rightarrow 500 = 5000t + \dfrac{1}{2}(0){t^2} \cr
  & \Rightarrow t = \dfrac{{500}}{{5000}} \cr
  & \Rightarrow t = \dfrac{1}{{10}}\sec \cr} $
Put $t = \dfrac{1}{{10}}\sec $ in ${s_y} = 5{t^2}m$
Then we will get
${s_y} = 5{t^2}m$
$\eqalign{
  & \Rightarrow {s_y} = 5{(\dfrac{1}{{10}})^2}m \cr
  & \Rightarrow {s_y} = 0.05m = 5cm \cr} $
Hence the vertical height gun should aim above the target is 0.05m i.e 5cm. Hence option A will be the answer.

Note: In this case a bullet is fired from the gun horizontally. If a bullet is projected diagonally then it will have an initial y component of velocity too. Hence in the formula along y-direction ‘u’ will not be equal to zero. This is a particular condition in which the aim is at a horizontal level to the barrel of the gun.